Method for extracting fractal profile for representing fabric texture and Sobel operator filtering detail mixed characteristic vector
A fabric texture and mixed feature technology, applied in the field of digital image processing and pattern recognition, can solve the problems of not being able to fully and meticulously characterize the essential characteristics of fabric texture, not being able to achieve more adequate and appropriate texture, and not considering the distribution of boundary points, etc.
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment 1
[0089] (1) Get the fabric image, such as figure 2 shown.
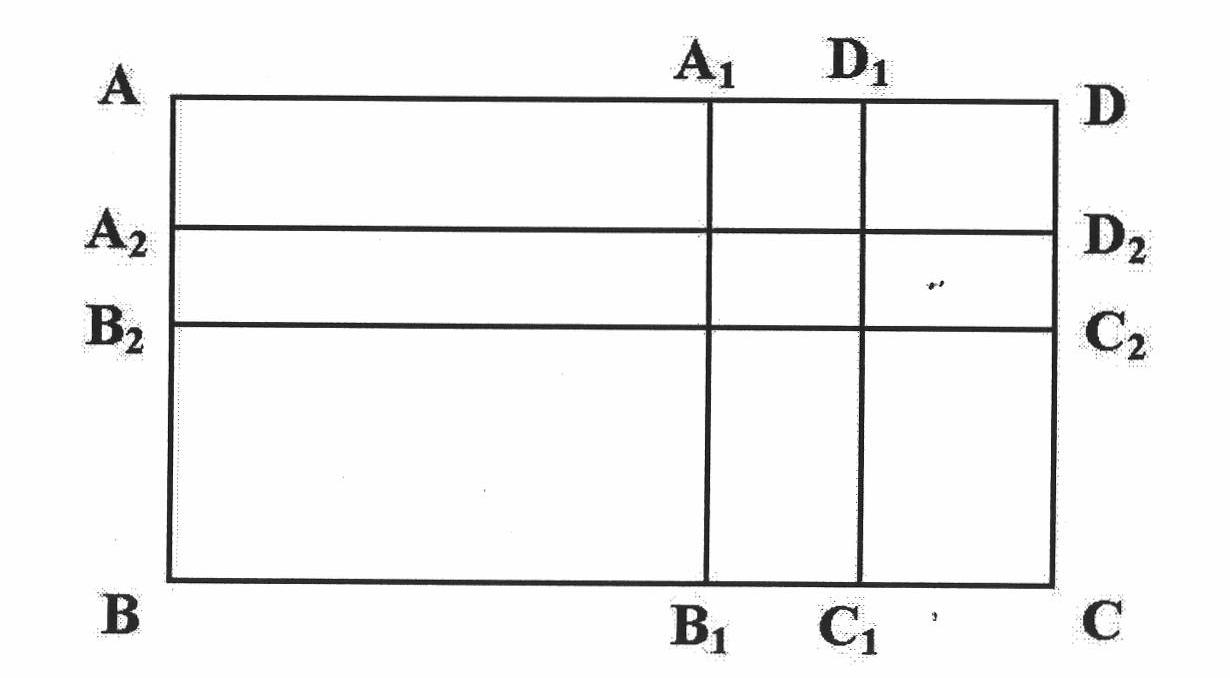
[0090](2) Calculate the mean value of each column and the mean value of each row of the image respectively to obtain two one-dimensional time series, connect the two sequences end to end to form a new time series, and use 2 to 6 pixels in the box size sequence δ In the case of , the box dimension of the time series is estimated by the box counting method, and the result is 1.55.
[0091] (3) Use one-dimensional FFT to calculate the period of any row of grayscale data in the original image, and obtain the column basic period P 1 = 6 pixels.
[0092] (4) Use one-dimensional FFT to calculate the period of any column of grayscale data in the original image, and obtain the basic period P of the row 2 = 4 pixels.
[0093] (5) Implement Sobel operator horizontal filtering on the original image to obtain the filtered image as image 3 shown.
[0094] (6) Implement Sobel operator vertical filtering on the original image ...
Embodiment 2
[0103] (1) Get the fabric image, such as Figure 5 shown.
[0104] (2) Calculate the mean value of each column and the mean value of each row of the image respectively to obtain two one-dimensional time series, connect the two sequences end to end to form a new time series, and use 2 to 6 pixels in the box size sequence δ In the case of , the box dimension of the time series is estimated by the box counting method, and the result is 1.34.
[0105] (3) Use one-dimensional FFT to calculate the period of any row of grayscale data in the original image, and obtain the column basic period P 1 = 20 pixels.
[0106] (4) Use one-dimensional FFT to calculate the period of any column of grayscale data in the original image, and obtain the basic period P of the row 2 = 11 pixels.
[0107] (5) Implement Sobel operator horizontal filtering on the original image to obtain the filtered image as Figure 6 shown.
[0108] (6) Implement Sobel operator vertical filtering on the original im...
Embodiment 3
[0117] (1) Get the fabric image, such as Figure 8 shown.
[0118] (2) Calculate the mean value of each column and the mean value of each row of the image respectively to obtain two one-dimensional time series, connect the two sequences end to end to form a new time series, and use 2 to 6 pixels in the box size sequence δ In the case of , the box dimension of the time series is estimated by the box counting method, and the result is 1.26.
[0119] (3) Use one-dimensional FFT to calculate the period of any row of grayscale data in the original image, and obtain the column basic period P 1 = 8 pixels.
[0120] (4) Use one-dimensional FFT to calculate the period of any column of grayscale data in the original image, and obtain the basic period P of the row 2 = 15 pixels.
[0121] (5) Implement Sobel operator horizontal filtering on the original image to obtain the filtered image as Figure 9 shown.
[0122] (6) Implement Sobel operator vertical filtering on the original ima...
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com