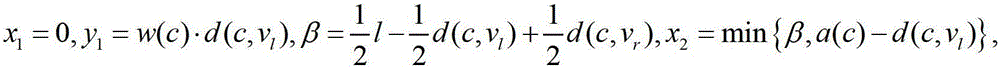Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Dynamic monitoring method and system for minimizing maximum distance position

A maximum distance and dynamic monitoring technology, applied in special data processing applications, instruments, electrical digital data processing, etc., can solve problems such as inability to solve the best position query problem

Inactive Publication Date: 2017-03-01
SHANGHAI JIAOTONG UNIV
View PDF4 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

The techniques in these research works do not solve the optimal location query problem, because the closest point query problem is different from the optimal location query problem

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Dynamic monitoring method and system for minimizing maximum distance position
  • Dynamic monitoring method and system for minimizing maximum distance position
  • Dynamic monitoring method and system for minimizing maximum distance position

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

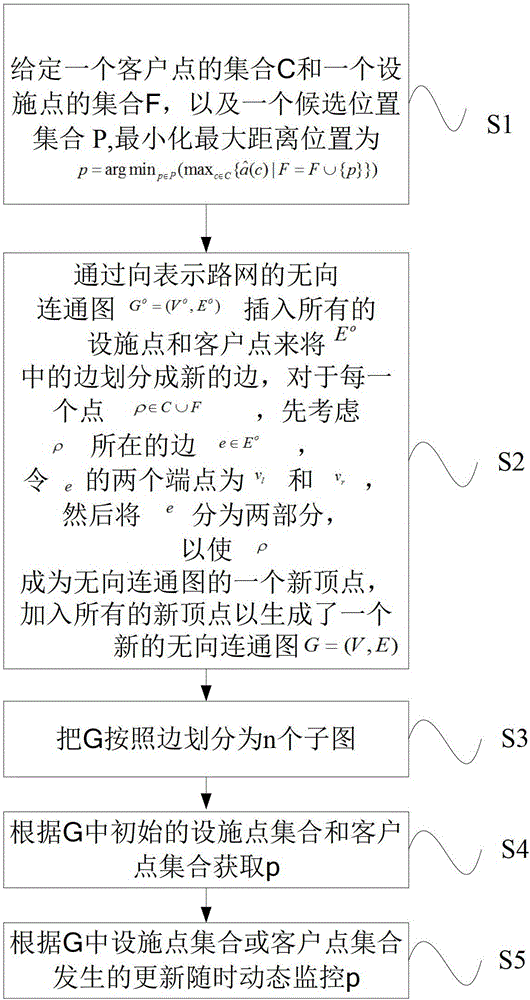
[0136] Such as figure 1 As shown, the present invention provides a dynamic monitoring method for minimizing the maximum distance position, including steps S1 to S5.

[0137] Step S1, given a set C of customer points, a set F of facility points, and a set P of candidate locations, the minimum maximum distance location is ,in is the weighted attraction distance of customer point c, w(c) is the weight of customer point c, if the distance d(c,f) between customer point c and facility point f in the road network is the minimum of the points in c and F value, then define f as the attractor of c, c is attracted by f, a(c)=d(c,f) is the attracting distance of c;

[0138] Step S2, through the undirected connected graph G representing the road network o =(V o ,E o ) to insert all facilities f and customers c to E o The edges in are divided into new edges. For each point ρ∈C∪F, first consider the edge e∈E where ρ is located o , let the two endpoints of e be v l and v r , and th...

Embodiment 2

[0195] The present invention also provides another dynamic monitoring system for minimizing the maximum distance position, comprising:

[0196] The definition module is used to give a set C of customer points, a set F of facility points, and a set P of candidate positions, and the minimum maximum distance position is ,in is the weighted attraction distance of customer point c, w(c) is the weight of customer point c, if the distance d(c,f) between customer point c and facility point f in the road network is the minimum of the points in c and F value, then define f as the attractor of c, c is attracted by f, a(c)=d(c,f) is the attracting distance of c;

[0197] The undirected connected graph module is used to represent the undirected connected graph G of the road network through directions o =(V o ,E o ) to insert all facilities f and customers c to E o The edges in are divided into new edges. For each point ρ∈C∪F, first consider the edge e∈E where ρ is located o , let t...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention provides a method and a system for dynamic monitoring of the minimized maximum distance location. The method includes presetting a customer point set C, a facility point set F and a candidate position set P; inserting all the facility points f and customer points c into an undirected graph shown as the formula Go=(Vo,Eo) for representing a road net to divide edges of the Eo into new edges; to each point p<CUF and on first consideration that the edge e where the points p located belongs to Eo, setting two end points of the e as v1 and vr, dividing the e into two parts, namely the part from the v1 to the p and the part from the p to the vr so as to make the p as a new vertex of the undirected graph, adding all the new vertexes to generate a new undirected graph shown as the formula G=(VE); dividing the undirected graph G into n sub graphs from G1 to Gn according to edges; acquiring the p according to the initial facility point set F and customer point set C of the undirected graph G; dynamically monitoring the p at any time according to updating of the facility point set F or customer point set C of the undirected graph G; wherein the minimized maximum distance location is shown as the following formula; the value of the n is set according to requirements of users. By the method and the system, the minimized maximum distance location can be searched quickly and dynamically.

Description

technical field [0001] The invention relates to a dynamic monitoring method and system for minimizing the maximum distance position. Background technique [0002] In the past few years, there has been a lot of work on a class of "facility placement problems" in the presence of sets of customer points (see reference 8: Farahani, R.Z., Hekmatfar, M.: Facility Location: Concepts, Models, Algorithms and Case Studies, 1st edn. Physica-Verlag HD (2009), Literature 15: Nickel, S., Puerto, J.: Location Theory: A Unified Approach, 1st edn. Springer (2005)). In the most general case, the problem consists of: (1) a set C of customer points and a set P of facility candidates, and (2) query the locations of k new facilities in P such that a pre-defined optimal condition. This kind of problem has a polynomial time algorithm when k is a constant, and it is an NP-hard problem when k is a general variable (see literature 8 and 15). The existing work mainly studies its approximate algorithm...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Patents(China)
IPC IPC(8): G06F17/30
Inventor 姚斌吴亦凡李飞飞肖小奎
Owner SHANGHAI JIAOTONG UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com