Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Compressive sensing based Gaussian matrix optimizing method

A technology of Gaussian matrix and optimization method, applied in the field of compressed sensing, can solve the problem of low signal reconstruction ability of Gaussian measurement matrix, achieve the effect of improving signal reconstruction ability and wide application prospect

Active Publication Date: 2012-08-01
GUANGXI UNIVERSITY OF TECHNOLOGY
View PDF3 Cites 13 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0004] In order to solve the problem of low signal reconstruction ability of Gaussian measurement matrix, the present invention provides an optimization method of Gaussian matrix

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Compressive sensing based Gaussian matrix optimizing method
  • Compressive sensing based Gaussian matrix optimizing method
  • Compressive sensing based Gaussian matrix optimizing method

Examples

Experimental program
Comparison scheme
Effect test

specific Embodiment approach 1

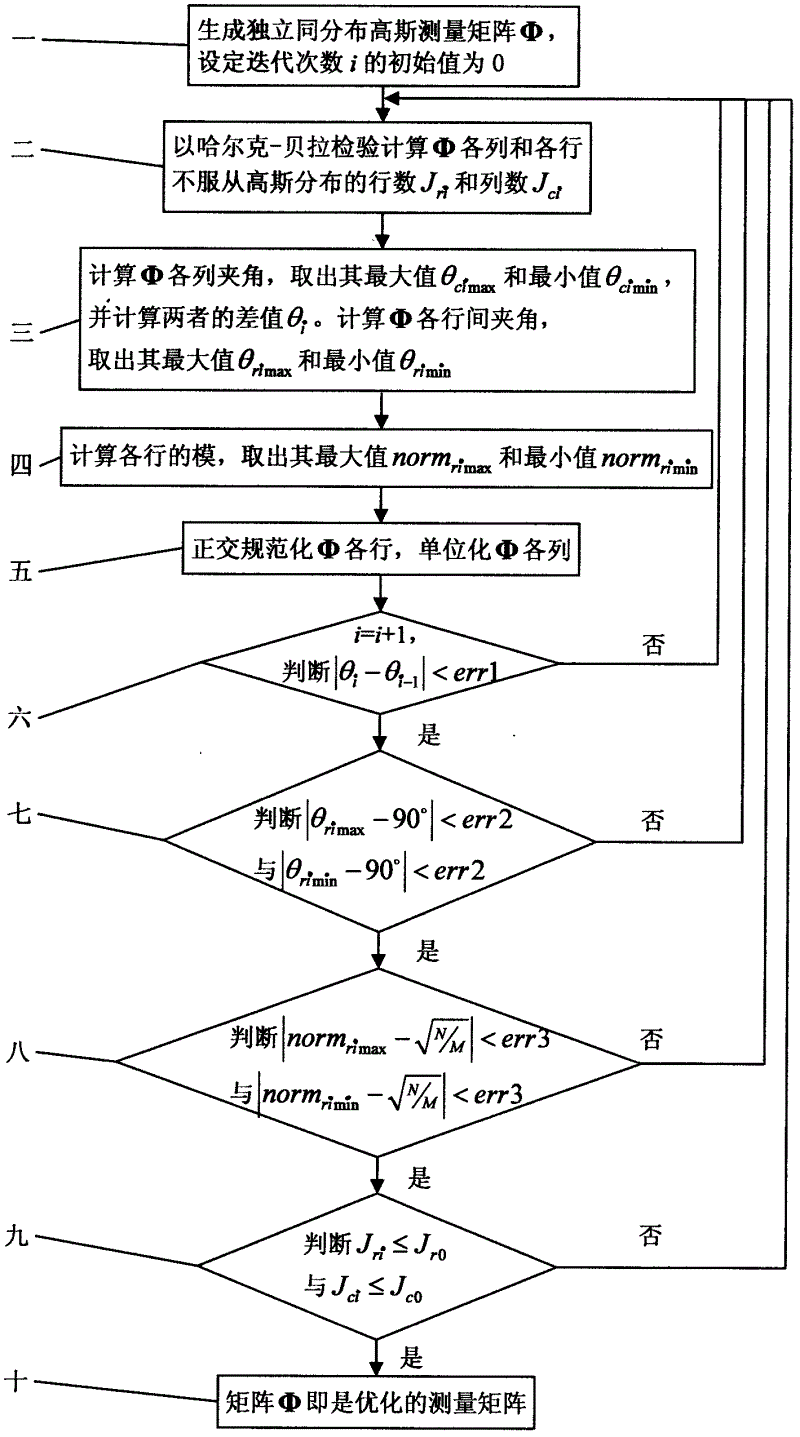
[0018] Specific implementation mode one: according to the instructions attached figure 1 This embodiment will be specifically described. The optimization process of a Gaussian matrix optimization method based on compressed sensing described in this embodiment is:

[0019] Step 1: Generate independent and identically distributed Gaussian measurement matrix Φ, where Φ∈R M×N , M

[0020] Step 2: Use the Jarque-Bera test to calculate the number of rows J that do not obey the Gaussian distribution in each column and row of Φ ri and column number J ci ;

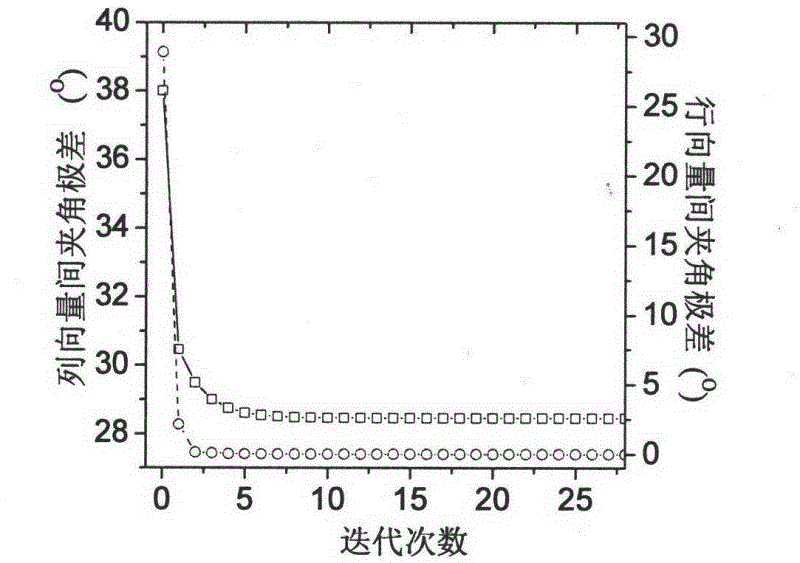
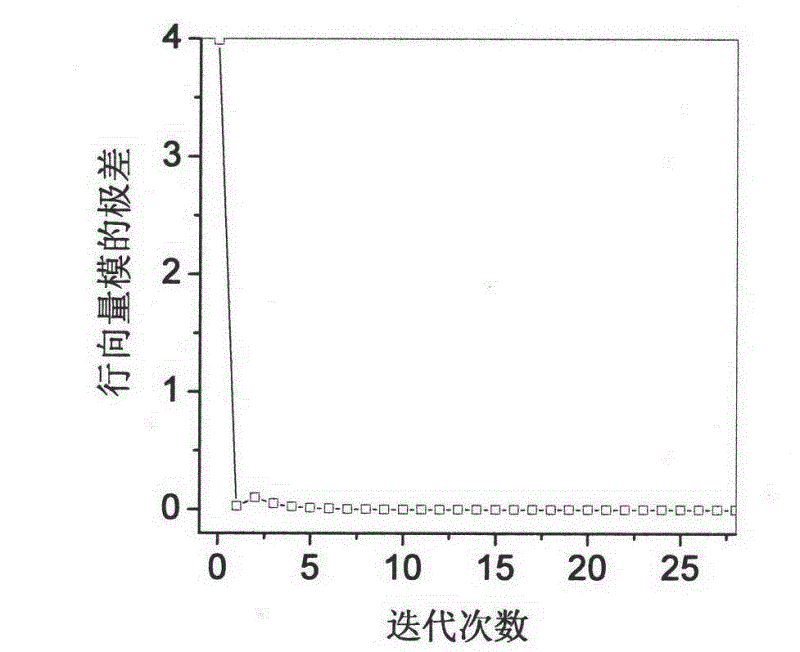
[0021] Step 3: Calculate the angle between each column vector of Φ, and take out its maximum value θ cimax and minimum θ cimin , and calculate the difference θ between the two i , calculate the angle between each ro...

specific Embodiment approach 2

[0029] Specific embodiment 2: This specific embodiment is a further description of a Gaussian matrix optimization method based on compressed sensing described in specific embodiment 1. In step 1, the iterative error err1 is set to 10 -9 , err2 is 10 -9 , err3 is 10 -9 .

specific Embodiment approach 3

[0030] Specific embodiment three: This specific embodiment is a further description of a Gaussian matrix optimization method based on compressed sensing described in specific embodiment one, the orthogonal normalization of each row vector of Φ described in step five, and the unitization of each column vector of Φ The specific process of is as follows: firstly, each row vector of Φ is orthogonalized, then each row vector is normalized, and finally each column vector is normalized.

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

A compressive sensing based Gaussian matrix optimizing method belongs to the technical field of measuring matrix optimization in compressive sensing and solves the problem of poor signal reconstruction capability of Gaussian matrix measuring matrix. The compressive sensing based Gaussian matrix optimizing method includes: subjecting row vectors to orthogonal standardization and column vectors to unitization for measuring matrix computed by the (i-1)th iteration through the ith iteration and completing optimization of Gaussian matrix by utilizing range of included angles among the column vectors, the maximum value and the minimum value of the included angles among the row vectors, the maximum value and the minimum value of row vector modules and the number of rows and columns inadaptive to Gaussian distribution as reference. The compressive sensing based Gaussian matrix optimizing method is applicable to optimization of Gaussian measuring matrix in the compressive sensing.

Description

technical field [0001] The invention belongs to the technical field of compressed sensing, and specifically provides an optimization method of a Gaussian matrix. Background technique [0002] Compressed sensing (Compressive sensing) can reconstruct the signal at a sampling rate far lower than that required by the Nyquist sampling theorem; it realizes the simplification of the data encoding end (acquisition, compression, encryption, transmission) and the decoding end (decompression, Refactoring) complex data processing landscape. Data compression and encryption are realized during the data acquisition process, and high-dimensional signals are directly converted into low-dimensional signals. Compressed sensing has broad application prospects in image processing, video analysis, radar remote sensing, communication coding, data mining and other fields, and will generate huge economic benefits in military reconnaissance, resource detection, medical biology, ultrasonic images, in...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06F17/16
Inventor 程涛
Owner GUANGXI UNIVERSITY OF TECHNOLOGY
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com