Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

A method for calculating the internal space charge distribution of XLPE cables using electromagnetic theory

A cross-linked polyethylene, charge distribution technology, applied in the direction of measuring electrical variables, instruments, measuring devices, etc., to achieve cost-saving effects

Active Publication Date: 2019-06-18
CHINA THREE GORGES UNIV
View PDF3 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0004] In view of this, the technical problem to be solved by the present invention is: in view of the current lack of theoretical calculation methods to calculate the space charge distribution inside the XLPE cable, a method for calculating the space charge distribution inside the XLPE cable using electromagnetic theory is proposed. method

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • A method for calculating the internal space charge distribution of XLPE cables using electromagnetic theory
  • A method for calculating the internal space charge distribution of XLPE cables using electromagnetic theory
  • A method for calculating the internal space charge distribution of XLPE cables using electromagnetic theory

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0019] A method for calculating the space charge distribution inside a cross-linked polyethylene cable with electromagnetic theory, comprising the following steps:

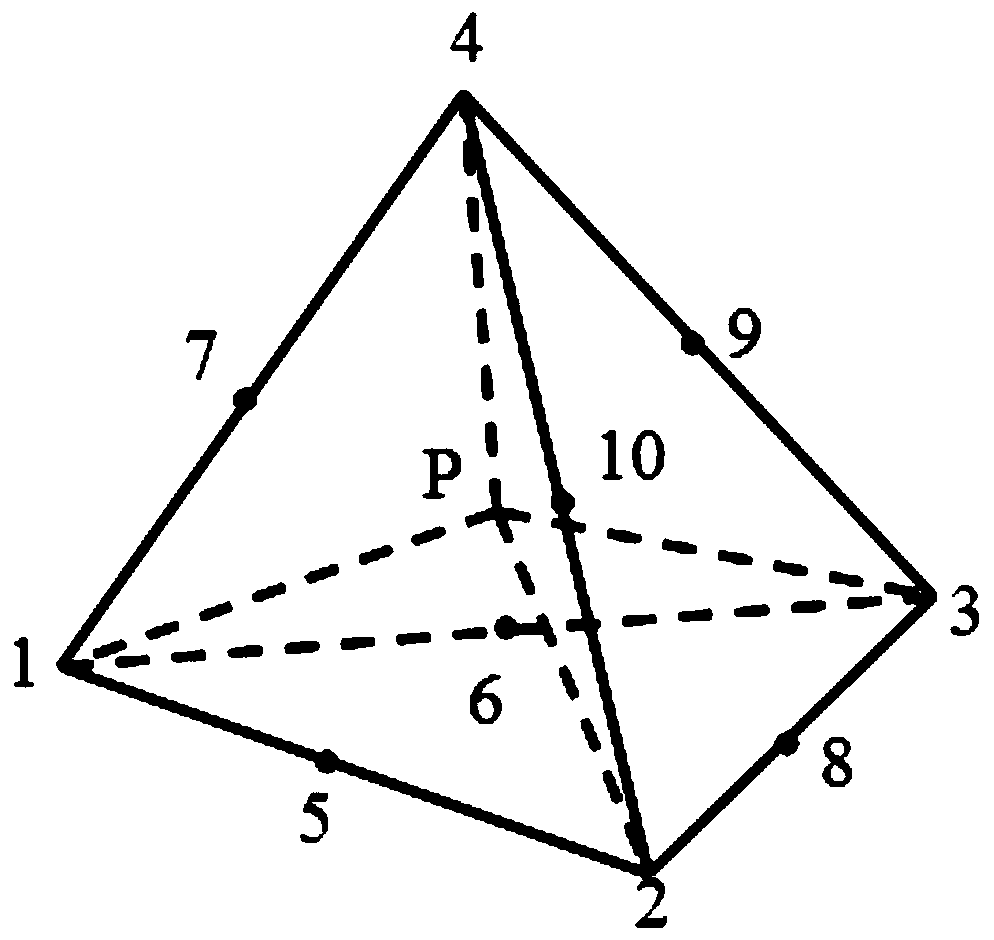
[0020] Step 1: Use the finite element analysis software to establish the cable model, use the second-order tetrahedron element to divide, apply the corresponding load to calculate the potential of each node, and export the node file, element file and potential file.

[0021] Step 2: According to the geometric characteristics of the three-dimensional tetrahedron unit, the volume coordinates are introduced as natural coordinates, such as figure 1 As shown, the volume coordinates of any point P(x, y, z) in the unit is:

[0022]

[0023] In the formula, L1, L2, L3, and L4 respectively represent the shape functions of the four vertices of the first-order tetrahedron, and vol represents the volume of the tetrahedron.

[0024]

[0025]

[0026]

[0027] Therefore there is L 1 +L 2 +L 3 +L 4 =1, the verte...

Embodiment 2

[0049] A method for calculating the space charge distribution inside a cross-linked polyethylene cable with electromagnetic theory, comprising the following steps:

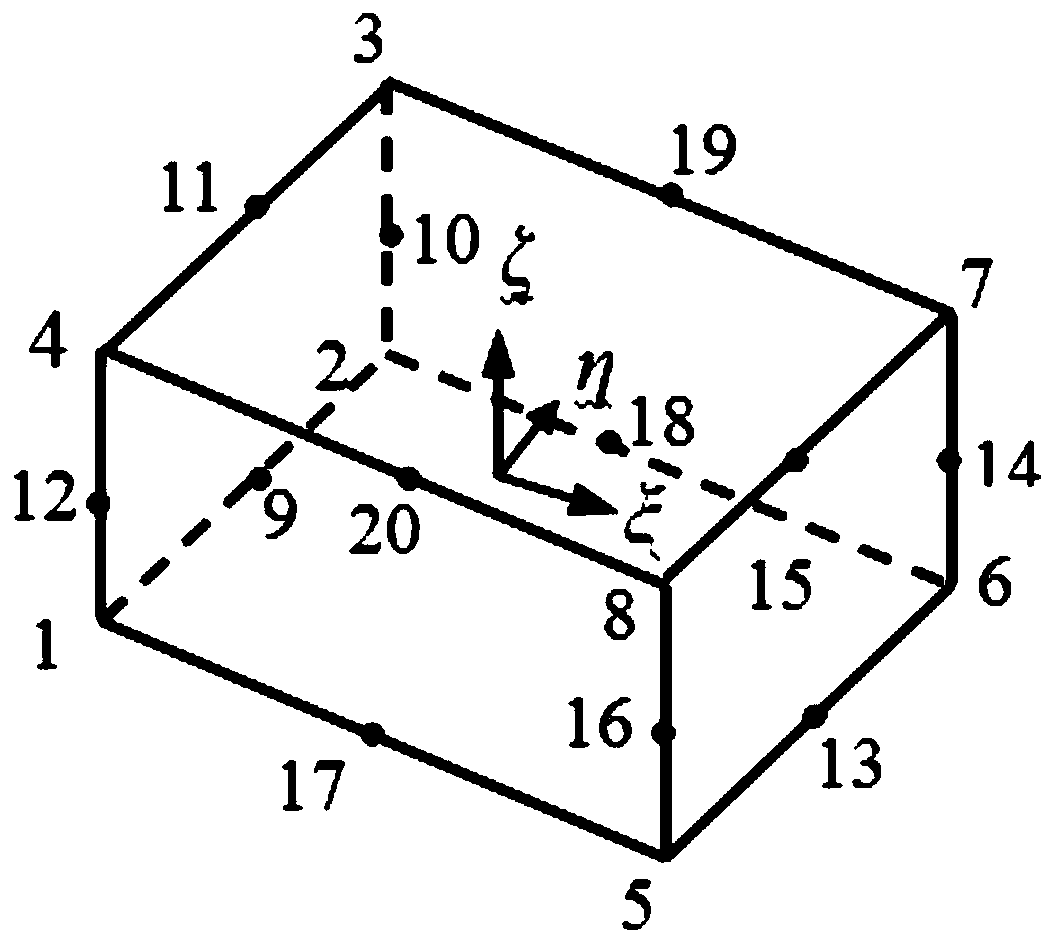
[0050] Step 1: Use the finite element analysis software to establish the cable model, use the second-order hexahedron element division, apply the corresponding load to calculate the potential of each node, and export the node file, element file and potential file;

[0051] Step 2: Introduce three natural coordinates ξ, η, ζ, such as figure 2 As shown, ξ represents the ratio of any point on the ξ axis to the distance between the origin and half the length of the hexahedron, η represents the ratio of any point on the η axis to the distance from the origin to half the width of the hexahedron, and ζ represents the distance between any point on the ζ axis and the origin and The ratio of half of the height of the hexahedron, so the variation range of ξ, η, ζ is:

[0052] -1≤ξ≤1

[0053] -1≤η≤1

[0054] -1≤ζ≤1

[00...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention relates to a method of calculating internal space charge distribution in a cross-linked polyethylene cable by means of an electromagnetic theory. The method of calculating internal space charge distribution in a cross-linked polyethylene cable by means of an electromagnetic theory includes the steps: using finite element analysis software to establish a cable model; utilizing a second order tetrahedron or hexahedron unit to perform dissection, and applying the corresponding load to calculate the potential of each node; performing antigradient operation on the potential of each node to obtain the electric field intensity of each node; and finally according to the electric field intensity, calculating the electric displacement vector, and obtaining the charge density of each node by performing divergence solution on the electric displacement vector. Compared with the current commonly used measuring method, the method of calculating internal space charge distribution in cross-linked polyethylene cable by means of electromagnetic theory has the advantages of having no loss, saving the cost, and being simple and easy. The method of calculating internal space charge distribution in a cross-linked polyethylene cable by means of an electromagnetic theory is suitable for distribution calculation of space charge accumulation, caused by the situation that impurities and bubbles exist in the cable, thus having great significance for judgment of the elevator fault and the service life.

Description

technical field [0001] The invention discloses a method for calculating the space charge distribution inside a cross-linked polyethylene cable by using electromagnetic theory, and relates to the fields of high-voltage power transmission and electrical equipment insulation aging. Background technique [0002] Power cables are important equipment for power transmission. Because the laying area of ​​the power cable is small, it is suitable to be buried underground, and it is widely used in the construction of urban power grids. With the rapid development of urbanization in China, the use of power cables will be huge in the future, and the safety of power cables will become very important. However, the aging and breakdown of XLPE cables are a big problem that plagues people. The space charge distribution inside the insulating material has a great influence on the electrical properties of the dielectric. The space charge will cause the distortion distribution of the electric fi...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Patents(China)
IPC IPC(8): G01R29/24
CPCG01R29/24
Inventor 李亚莎代亚平花旭沈星如
Owner CHINA THREE GORGES UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com