Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

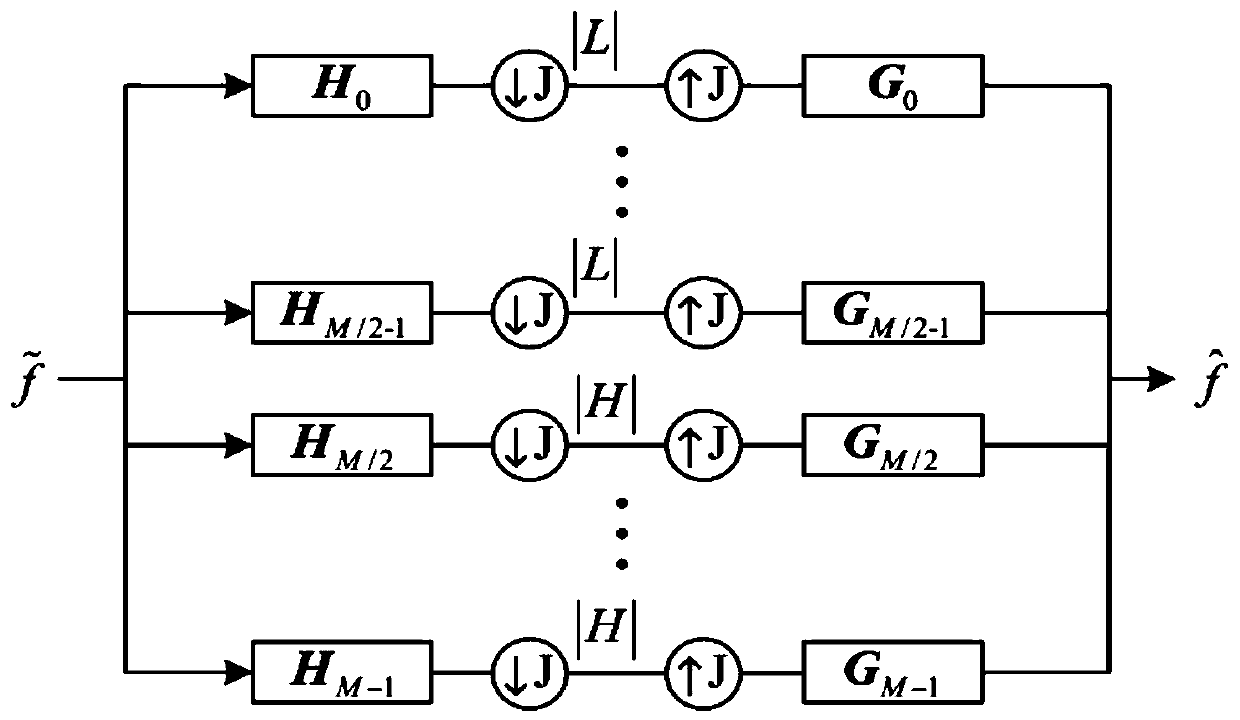
Optimal design method of m-channel oversampling graph filter bank based on convex optimization

A filter bank and optimization design technology, applied in design optimization/simulation, computer-aided design, CAD circuit design, etc., can solve problems such as poor performance, improve overall performance, restore simple and effective reconstruction, and reduce reconstruction errors Effect

Active Publication Date: 2020-07-10
GUILIN UNIV OF ELECTRONIC TECH
View PDF7 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0004] What the present invention is to solve is the problem that the overall performance of the existing M-channel oversampling image filter bank is relatively poor, and a method for optimizing the design of the M-channel oversampling image filter bank based on convex optimization is provided.

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Optimal design method of m-channel oversampling graph filter bank based on convex optimization
  • Optimal design method of m-channel oversampling graph filter bank based on convex optimization
  • Optimal design method of m-channel oversampling graph filter bank based on convex optimization

Examples

Experimental program
Comparison scheme
Effect test

example 1

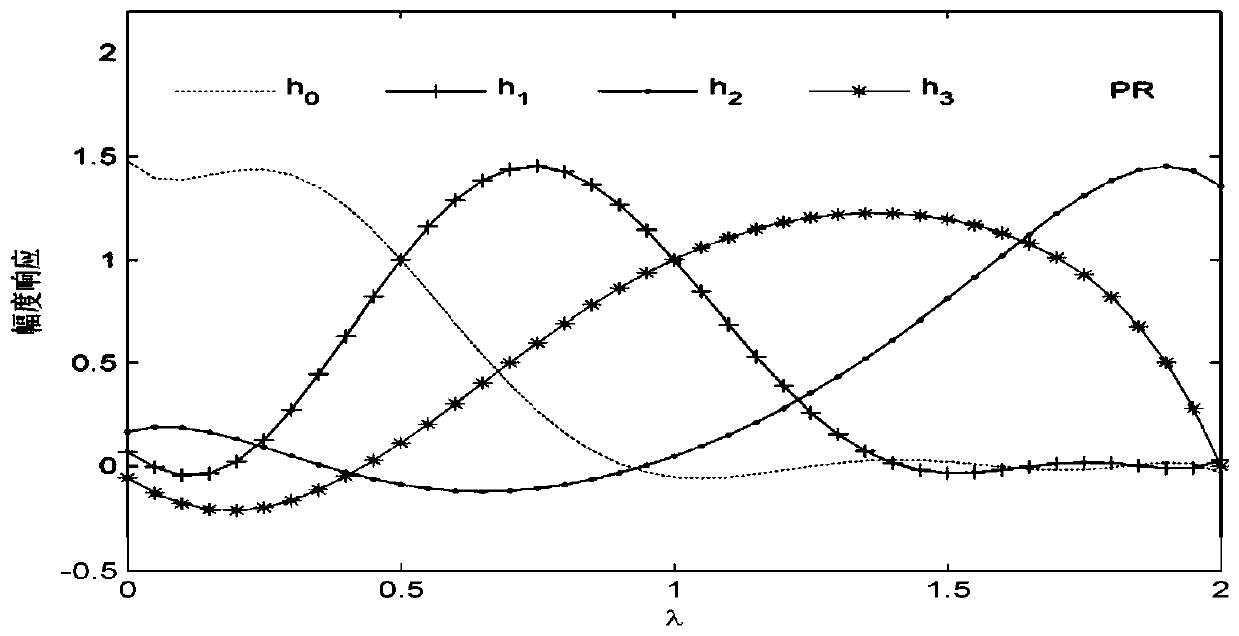
[0084] Design a graph filter bank with the following parameters: L h0 =12,L h1 =12,L g0 =11, L g1 =11,λ pd0 =0.4,λ pd1 =0.6,λ pd2 =0.8,λ sd0 =0.75,λ sd1 =0.25,λ sd2 = 1.3, α = 0.1, ε r =10 -9 , resulting in a graph filter bank magnitude response as figure 2 shown. PR in the figure represents the value of l(λ)+l(2-λ), λ∈[0,2], which is used to measure the reconstruction error of the graph filter bank. The values ​​of maximum reconstruction error and orthogonality calculated by simulation are E max =3.9346×10 -9, Θ=0.9353, under the same graph filter bank length and operating environment, Table 1 provides the reconstruction performance and Boundary ratio comparison.

[0085] Table 1

[0086]

[0087] R B is the boundary ratio, Take R during simulation B Compared with the maximum value of R B Equal to 1 indicates that the filter bank is fully reconstructed, and the reconstruction characteristics of the filter bank are better at this time. The maximum bou...

example 2

[0089] Design a graph filter bank with the following parameters: L h0 =8,L h1 =8,L g0 =7,L g1 =7,λ pd0 =0.3,λ pd1 =0.6,λ pd2 =0.8,λ sd0 =0.75,λ sd1 =0.25,λ sd2 = 1.3, α = 0.1, ε r =10 -13 , resulting in a graph filter bank magnitude response as image 3 shown. Under the same graph filter bank length and operating environment, Table 2 shows the reconstruction performance and orthogonality comparison between the graph filter bank designed by the present invention and the existing method 2 (M channel oversampling).

[0090] Table 2

[0091]

[0092] The closer the orthogonality Θ is to 1, the better the orthogonality of the filter bank is. It can be seen from the comparison that the reconstruction error of the graph filter bank designed by the present invention is obviously smaller than that of the existing method, and its orthogonality and overall spectral characteristics are slightly better than the existing method. Compared with Example 1, changing the paramet...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses an optimum design method for an M channel oversampling image filter set based on convex optimization. By means of a two-step method, target optimization is performed, the spectral selectivity and perfect reconstruction conditions of the image filter set are comprehensively considered, and therefore it is ensured that the whole performance of the image filter set is improved under the small reconstruction error condition. The simple and effective solution is provided for lowering the reconstruction error of the image filter set and achieving signal restoration and reconstruction.

Description

technical field [0001] The invention relates to the technical field of multi-rate signal processing, in particular to an optimal design method of an M-channel oversampling graph filter bank based on convex optimization. Background technique [0002] Graph signal processing has important application significance in social, biological, transportation, sensor network processing, and image processing. In practical applications, the amount of graph data is very large, which brings certain difficulties to graph signal processing. Multi-resolution analysis is a kind of localized time-frequency analysis, which can perform multi-scale fine-grained analysis on the signal and effectively extract the characteristic information of the signal, but it has certain limitations for big data processing. However, for big data, wavelets and filter banks are more suitable for processing large-scale graph signals. Wavelets and filter banks can sparsely represent graph signals, which is more advan...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Patents(China)
IPC IPC(8): G06F30/373G06F30/367
CPCG06F30/20G06F30/36
Inventor 蒋俊正刘松辽欧阳缮刘庆华谢跃雷晋良念丁勇曹想杨玉琳江庆
Owner GUILIN UNIV OF ELECTRONIC TECH
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com