Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Method for improving motion planning control precision of mechanical arm

A technology of control accuracy and motion planning, applied in manipulators, program-controlled manipulators, manufacturing tools, etc., can solve problems such as the decline of tracking performance at the end of the manipulator, and achieve the effect of solving the decline in tracking performance and improving control accuracy.

Active Publication Date: 2019-12-13
SUN YAT SEN UNIV
View PDF8 Cites 1 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

At the same time, by introducing the extrapolation method, the control model under the condition that the control signal may be lost is realized to solve the problem of the degradation of the tracking performance at the end of the manipulator

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Method for improving motion planning control precision of mechanical arm
  • Method for improving motion planning control precision of mechanical arm
  • Method for improving motion planning control precision of mechanical arm

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0041] Embodiment 1 is an existing tension-dynamic model without a strategy in the case of possible loss of control signals, and Embodiment 2 is a tension-dynamic model of an extrapolation strategy in the case of possible loss of control signals in this patent.

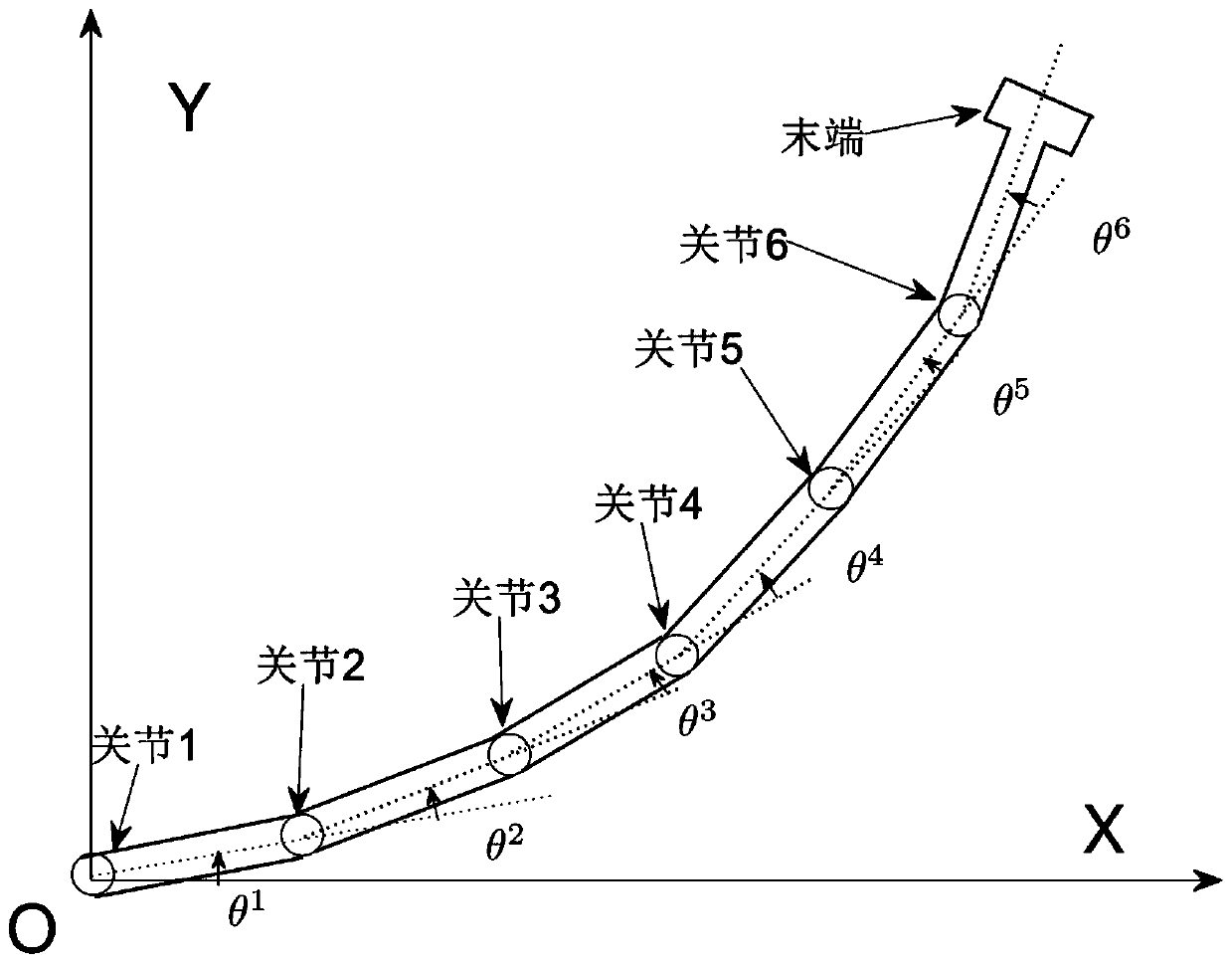
[0042] Such as figure 2 As shown, Embodiment 1 and Embodiment 2 are based on the six-link robotic arm model.

[0043] Example 1

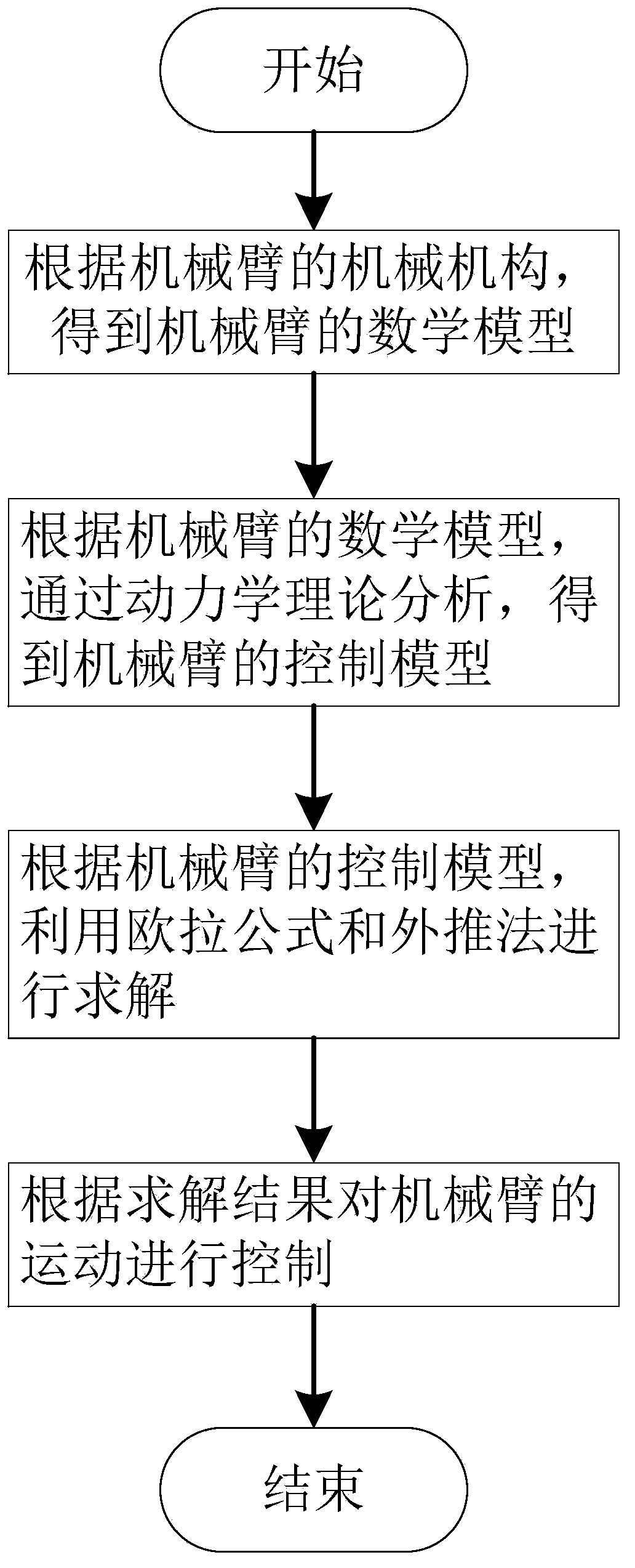
[0044] A method for motion planning control of a mechanical arm, comprising the following steps:

[0045] According to the mechanical mechanism of the mechanical arm, the mathematical model of the mechanical arm is obtained, and the mathematical model is expressed by the following formula:

[0046]

[0047]

[0048] k represents the kth moment, f( ) is a nonlinear mapping function, θ k is the angle of the joint angle of the manipulator at the kth moment, is the actual end trajectory of the manipulator at the kth moment, is the ideal end trajectory of the manipulator at the kth...

Embodiment 2

[0061] Such as figure 1 As shown, a method of improving the control accuracy of the motion planning of the manipulator includes the following steps:

[0062] According to the mechanical mechanism of the mechanical arm, the mathematical model of the mechanical arm is obtained, and the mathematical model is expressed by the following formula:

[0063]

[0064]

[0065] k represents the kth moment, f( ) is a nonlinear mapping function, θ k is the angle of the joint angle of the manipulator at the kth moment, is the actual end trajectory of the manipulator at the kth moment, is the ideal end trajectory of the manipulator at the kth moment, is the time derivative of the angle of the joint angle of the manipulator at the kth moment, is the time derivative of the end trajectory of the manipulator at the actual kth moment, J( ) is the Jacobian matrix;

[0066] According to the mathematical model of the manipulator, the control model of the manipulator is obtained throug...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a method for improving motion planning control precision of a mechanical arm. The method comprises the steps of obtaining a mathematical model of the mechanical arm according to the mechanical mechanism of the mechanical arm; according to the mathematical model of the mechanical arm, obtaining a control model of the mechanical arm through dynamic theoretical analysis; carrying out solving by utilizing an Euler formula and an extrapolation method according to the control model of the mechanical arm; and controlling the motion of the mechanical arm according to the solvedresult. According to the method, the dynamic method is adopted, and under the ideal condition, the tracking control of the actual tail end track of the mechanical arm on the ideal track is successfully realized on the speed layer; and meanwhile, by introducing the extrapolation method, the control model is obtained under the condition that a control signal can be lost, so that the motion planningcontrol precision of the mechanical arm is improved, and the problem that the tracking performance of the tail end of the mechanical arm is reduced is solved.

Description

technical field [0001] The invention relates to the field of manipulator control, and more specifically, to a method for improving the control precision of the motion planning of the manipulator. Background technique [0002] As a booming research field, robots have been widely used in industries such as industry and service industries, such as spraying robots, welding robots, vehicle-mounted mechanical arms, etc. in the industry, customer service robots, and rehabilitation assistance robots in the service industry. Wait. Many robot control problems can be classified as manipulator motion planning control problems. The key to solving such problems is to obtain an effective manipulator motion planning control model, so that the manipulator can rotate the joint angle under certain constraints to achieve the ideal end trajectory tracking. track requirements. Robotic arm control systems are usually divided into offline control systems and online control systems. For the off-l...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): B25J9/16
CPCB25J9/1664
Inventor 张雨浓凌一宏黄焕昌施妮妮毛明志
Owner SUN YAT SEN UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com