Methods for converting partial Hadamard matrix to Gauss matrix
A Gaussian matrix and Hadar technology, which is applied in the field of transformation from partial Hadamard matrix to Gaussian matrix, can solve the problem of poor randomness of Hadamard matrix
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
specific Embodiment approach 1
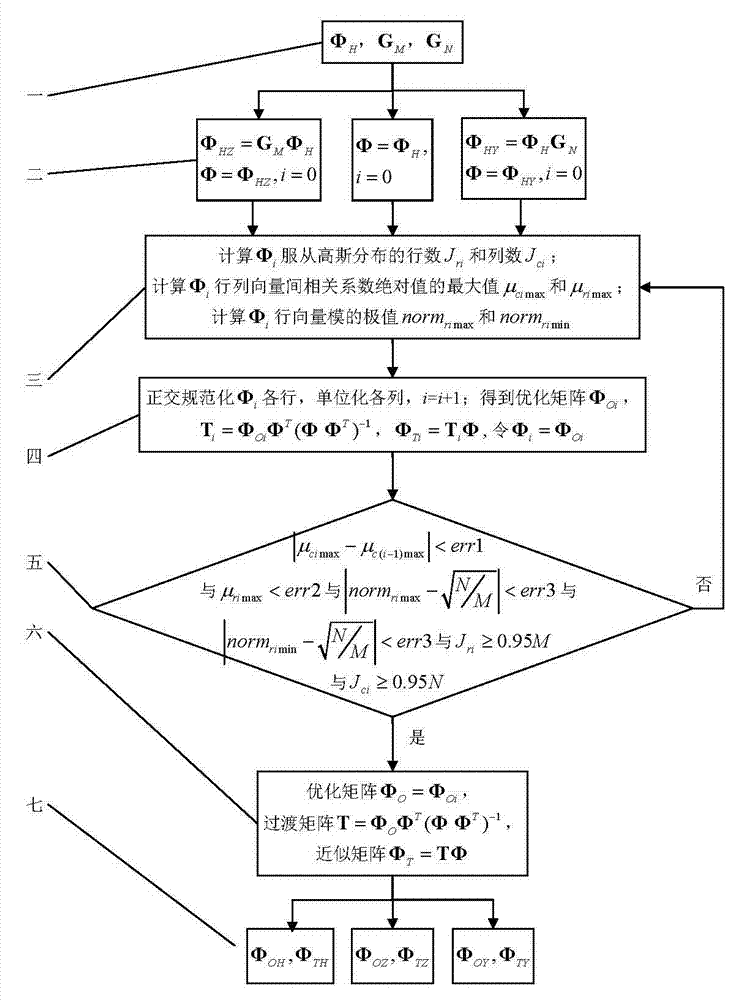
[0015] Specific implementation mode one: according to the instructions attached figure 1 This embodiment will be specifically described. Partial Hadamard matrix to Gaussian matrix conversion method, the process of the method is:
[0016] Step 1: Generate Hadamard Matrix , left-multiplied random matrix and right multiplying the random matrix ,in , , , , and are all natural numbers;
[0017] Step two: Left multiply random matrix generate , right multiply random matrix generate ,make or or or , set the number of iterations i The initial value of 0, set the iteration error ;
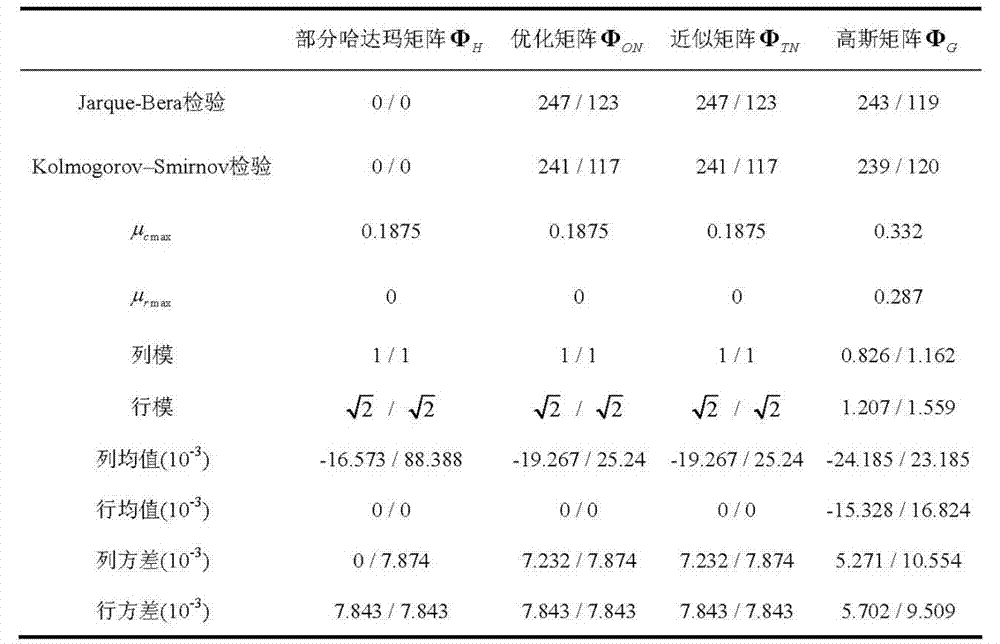
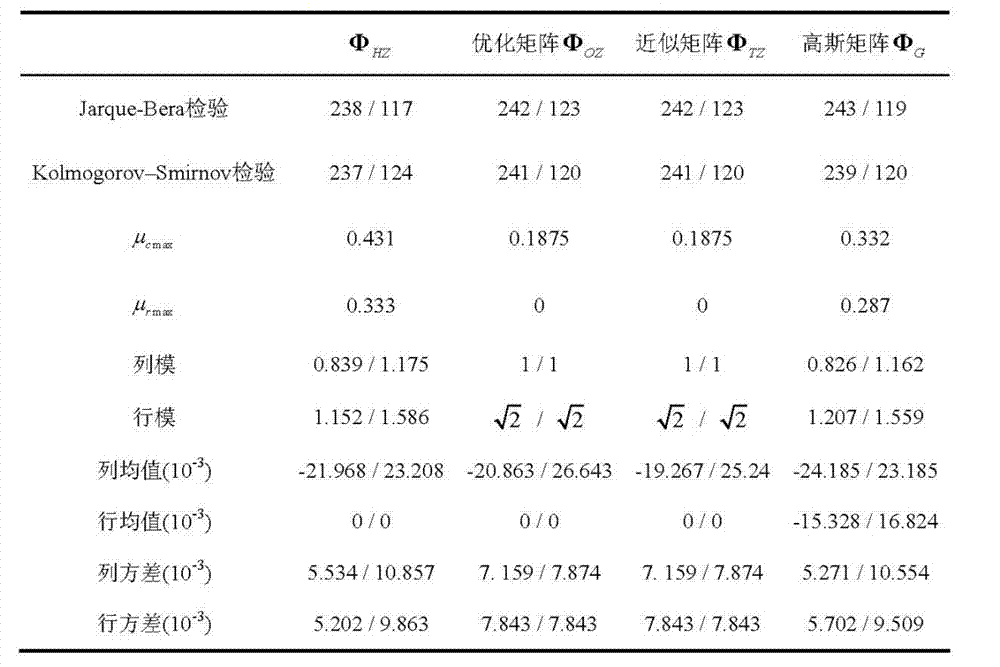
[0018] Step 3: Calculation by Jarque-Bera test The number of rows that follow a Gaussian distribution for each column and row and number of columns ;calculate The correlation coefficient between each column vector, take the maximum value of its absolute value ;Calculate the correlation coefficient between each row vector, and take out the maximum value...
specific Embodiment approach 2
[0023] Specific implementation mode two: this specific implementation mode is a further description of the conversion method from the partial Hadamard matrix to the Gaussian matrix described in the specific implementation mode one, and the iterative error is set in step 2 err 1 for , err 2 for , err 3 for .
specific Embodiment approach 3
[0024] Specific embodiment three: This specific embodiment is a further description of the conversion method from the partial Hadamard matrix to the Gaussian matrix described in the specific embodiment one, and the orthogonal normalization described in step four Each row vector, and then the specific process of unitizing each column vector is: first Orthogonalize the row vectors, then normalize the row vectors, and finally normalize the column vectors.
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com