Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Automobile active suspension anti-saturation control method based on self-adaptive control

An adaptive control, active suspension technology, used in suspension, elastic suspension, vehicle components, etc.

Active Publication Date: 2015-10-28
哈尔滨工业大学人工智能研究院有限公司
View PDF6 Cites 7 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0009] The purpose of the present invention is to solve the problems in the prior art that the output force of the actuator can be measured, the saturation value of the actuator is known, and the passive control method cannot solve the nonlinear problem in the suspension system well, and a proposed method is proposed. An anti-saturation control method for automotive active suspension based on adaptive control

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Automobile active suspension anti-saturation control method based on self-adaptive control
  • Automobile active suspension anti-saturation control method based on self-adaptive control
  • Automobile active suspension anti-saturation control method based on self-adaptive control

Examples

Experimental program
Comparison scheme
Effect test

specific Embodiment approach 1

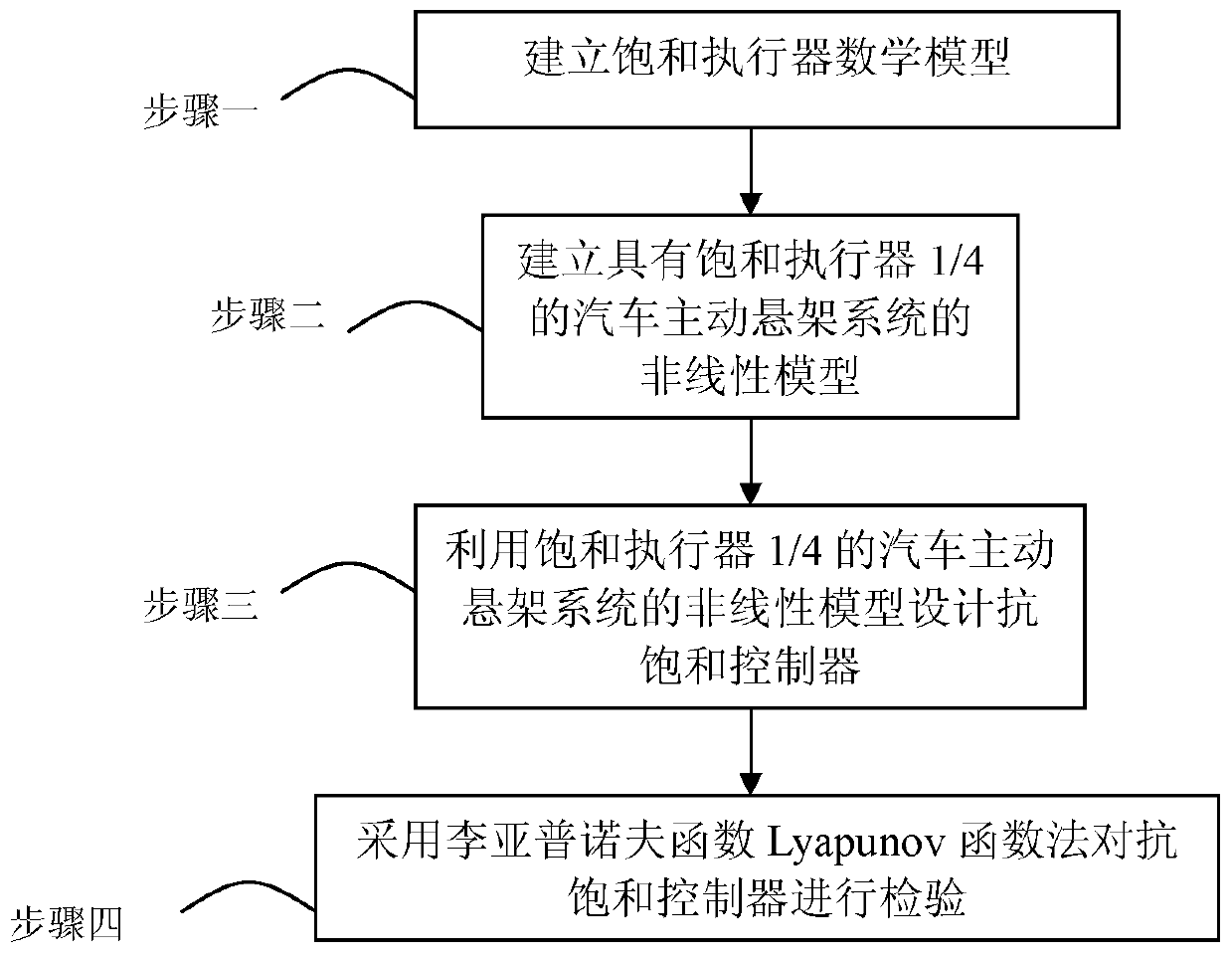
[0043] Specific implementation manner 1: The anti-saturation control method of an automobile active suspension based on adaptive control of this embodiment is specifically prepared according to the following steps:

[0044] Step 1: Establish a mathematical model of the saturation actuator;

[0045] The standard saturated actuator mathematical model is as follows:

[0046] u = s a t ( ν ) = u max , i f ν ≥ u max , ν , i f u min ν u max , u min , i f ν ≤ u min , - - - ( 1 )

[0047] It can be seen from the saturated actuator function that the input of the actuator ν≤u min Or ν≥u max In the interval of, the output of the actuator maintains a maximum or minimum value, which has an obvious nonlinear characteristic; the nonlinearity of the actuator output brings unstable factors to the entire suspension system and affects the suspens...

specific Embodiment approach 2

[0072] Specific embodiment two: this embodiment is different from specific embodiment one in: combination figure 2 The specific process of establishing the nonlinear model of the automobile active suspension system with saturated actuator 1 / 4 in step two is:

[0073] The mathematical model of the automobile suspension system with saturated actuator 1 / 4 is as follows:

[0074] m s z ·· s = - F d ( z · s , z · u , t ) - F s ( z s , z u , t ) + s a t ( ν ( t ) ) + f ( t ) m u z ·· u = F d ( z · s , z · u , t ) + F s ( z s , z u , t ) - F t ( z u , z r , t ) - F b ( z · u , z · r , t ) - s a t ( ν ( t ) ) - - - ( 5 )

[0075] among them, F s (z s ,z u ,t)...

specific Embodiment approach 3

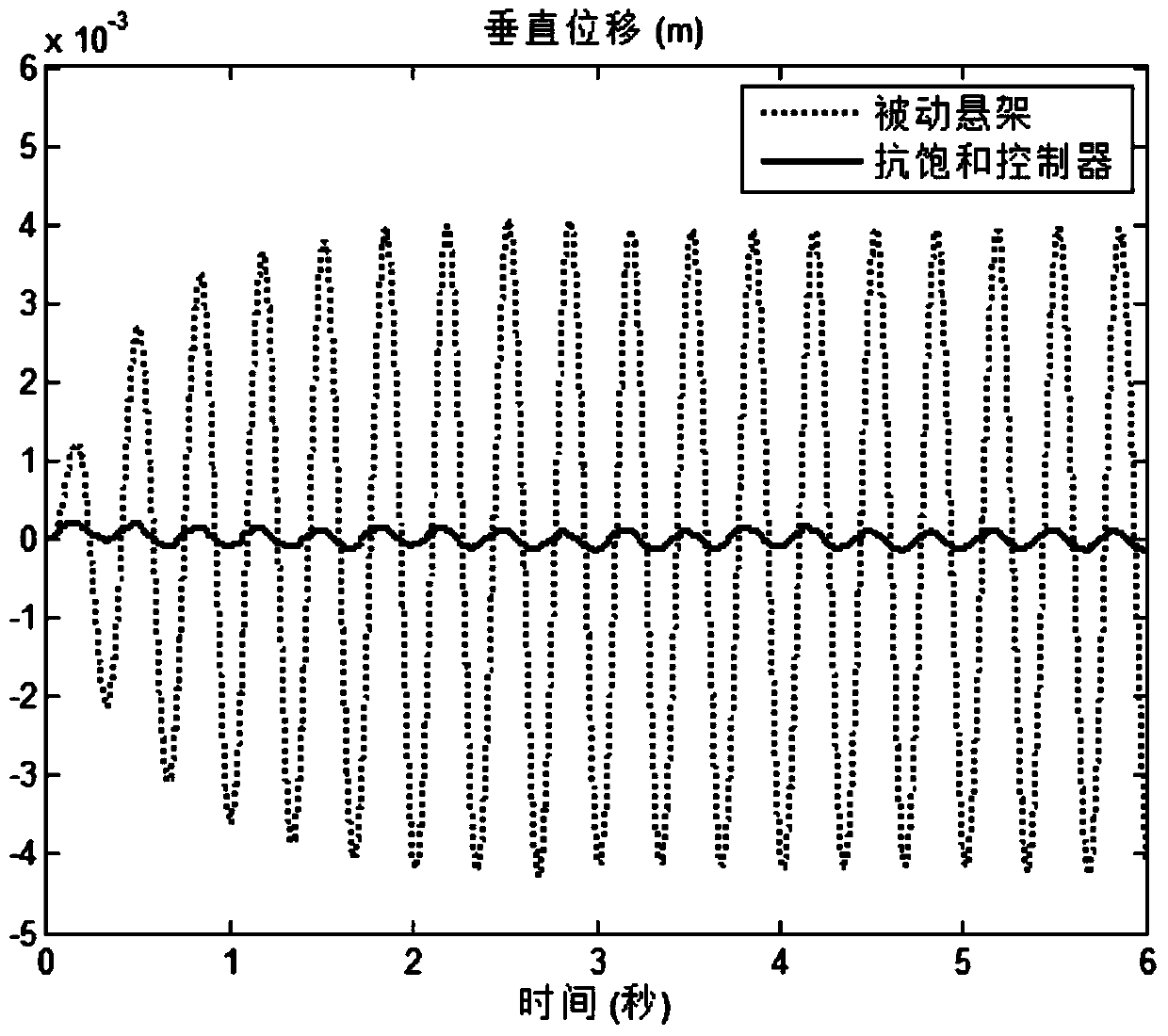
[0078] Specific embodiment three: This embodiment is different from specific embodiment one or two in that the specific process of designing the anti-windup controller in step three:

[0079] (1) The state variable of 1 / 4 car active suspension is determined as: x 1 =z s , x 3 =z u with

[0080] Rewrite formula (5) into the state equation:

[0081] x · 1 = x 2 , x · 2 = θ 1 s a t ( ν ) + θ 2 T F ( x , t ) + f ( t ) , x · 3 = x 4 , x · 4 = 1 m u ( F d ( z · s , z · u , t ) + F s ( z s , z u , t ) s - F t ( z u , z r , t ) - F b ( z · u , z · r , t ) - s a t ( ν ) ) , - - - ( 7 )

[0082] among them, θ 1 = 1 m s , θ 2 ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses an automobile active suspension anti-saturation control method based on self-adaptive control, and relates to an anti-saturation control method. To solve the problems in the prior art that the output power of an actuator needs to be considered, the saturation value of the actuator is known, and a linear control method cannot well solve nonlinear problems in a suspension system, the automobile active suspension anti-saturation control method based on self-adaptive control is provided. The method comprises the following steps: 1, establishing a saturated actuator mathematical model; 2, establishing a nonlinear model of a 1 / 4 automobile active suspension system of the saturated actuator; 3, designing an anti-saturation controller by utilizing the 1 / 4 automobile active suspension system of the saturated actuator; 4, checking the anti-saturation controller according to a liapunov function method. The automobile active suspension anti-saturation control method is applied to the field of anti-saturation control.

Description

Technical field [0001] The invention relates to a method for controlling the active suspension of an automobile, in particular to the anti-saturation design of the suspension actuator. Background technique [0002] Automobile suspension is mainly used to isolate the interference from uneven road surface when the vehicle is running, and is designed to improve the driving comfort of the vehicle. Compared with the traditional passive suspension and semi-active suspension system, the active suspension system is more superior in controlling the performance of the car body. [0003] Suspension systems usually mainly include springs and shock absorbers. There are a large number of nonlinear factors and uncertain factors in the vehicle suspension system itself and the application environment, which mainly include the following two parts: [0004] 1. Each component of the vehicle suspension system exhibits nonlinearity. For example: the spring load capacity in the suspension system is limi...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): B60G17/00
Inventor 孙维超潘惠惠张云霁高会军
Owner 哈尔滨工业大学人工智能研究院有限公司
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com