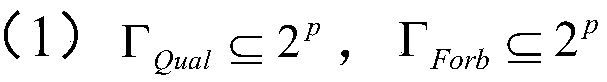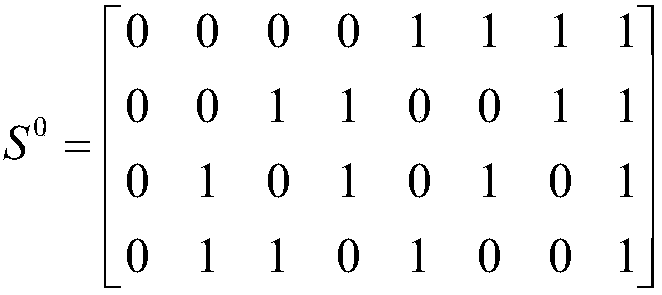Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

A threshold visual cryptography matrix construction method

A visual password and threshold technology, applied in the field of secret sharing and visual passwords, can solve the problems of disorganized sharing and obtaining secret information, and achieve the effect of simple and efficient construction process and quality assurance.

Pending Publication Date: 2019-05-07
CHENGDU UNIV OF INFORMATION TECH
View PDF2 Cites 3 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

Each share is as chaotic as random noise, and attackers cannot directly obtain secret information from a single share

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • A threshold visual cryptography matrix construction method
  • A threshold visual cryptography matrix construction method
  • A threshold visual cryptography matrix construction method

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0048] The (n, n) threshold scheme is illustrated below according to a specific embodiment:

[0049] If n=4, the number of rows of the basic matrix is ​​4, and the construction of the columns is according to S 0 Contains only 0, 2, 4 with even Hamming weight, S 1 The method that only includes 1 and 3 whose Hamming weight is an odd number is carried out, and the two base matrices are:

[0050]

[0051]

Embodiment 2

[0053] Let n=2, then the number of rows of the basic matrix is ​​2, and the structure of the columns is according to S 0 Contains only 0, 2, S with an even Hamming weight 1 The method that only includes 1s with an odd Hamming weight is performed, and the two basis matrices are:

[0054]

[0055] The following is the method for constructing the visual password matrix of any structure provided by the present invention by accumulating from the array.

[0056] The accumulation from the array is constructed on the basis of the above (n, n) threshold scheme. First build a cumulative array, the construction method of the cumulative array: construct a matrix CA of |P|×|T|, where |T|=|Γ ∞ |, if and only if , CA(i,j)=1, F is the set Γ ∞ collection of neutrons.

[0057] P is the set of participants in the visual cryptography scheme, and we consider the array P to be known. And T refers to the collection contained in the forbidden subset and the authorized subset; the collection...

Embodiment 3

[0067] The matrix construction method of the present invention is described below through the present embodiment:

[0068] Let n=4, P={1,2,3,4}, Γ 0 ={{1,2},{2,3},{3,4}},Γ ∞ ={{1,4},{1,3},{2,4}} can get F 1 = {1,4}, F 2 ={1,3},F 3 ={2,4}, then |T|=|Γ ∞ |=3, first construct the accumulative array, you can get:

[0069]

[0070] Then construct the basis matrix of the (3,3) threshold:

[0071]

[0072] At this time, observing CA can get col1={3}, col2={1,2}, col3={1,3}, col4={2}, from which the basis matrix can be calculated, as follows, col1=3, so S 0' and S 1' Line 3 of S as 0 and S 1 The first line of ; col2={1,2}, the S 0' and S 1' Lines 1 and 2 are ORed as S 0 and S 1 The second line of , so by analogy, the basis matrix can be obtained as follows:

[0073]

[0074]The method of the invention can realize the construction of the basic matrix of visual cryptography with any structure. As a visual cipher with hidden and shared secret technology, one of th...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention provides a threshold visual cryptography matrix construction method, which comprises the following steps of: constructing an accumulation counting group which consists of an | P | * | T| matrix CA; After the array P, the authorization subset and the forbidden subset are determined, determining F, wherein F is a set in Gamma infinity; Determining a set Gamma infinity, wherein the number of sets in the Gamma infinity is equal to that of the Gamma infinity; Constructing a matrix CA; Constructing basis matrixes S0'and S1 'of the accumulation array according to a (n, n) threshold scheme; Observing the distribution condition of the position with the Hamming weight of 1 in each line in the matrix CA, and taking the line sequence of the position with the Hamming weight of 1 in thematrix CA as the line sequence of the base matrixes S0 and S1; And so on, obtaining final basis matrixes S0 and S1. Due to the fact that the column number of the formed basis matrix is relatively small, the smaller the column number is, the smaller the pixel expansion degree is, the phenomenon that the decryption effect is affected due to the fact that an image is excessively stretched when secrets are recovered is avoided, and the quality of the decrypted image is guaranteed to a great extent.

Description

technical field [0001] The invention belongs to the technical field of secret sharing and visual cryptography, and in particular relates to a novel threshold visual cryptography matrix construction method. Background technique [0002] Visual Cryptography (Visual Cryptography) was proposed by Naor and Shamir at the European Cryptography Annual Conference in 1994. Based on the idea of ​​threshold secret sharing, it combines secret sharing and digital images to form a new research hotspot. The principle of visual cryptography is that the original image is decomposed into two random visual codon graphs, and it is impossible to obtain the original image information from a single visual code subgraph. The original image could only be reproduced if the two visual codon maps were completely coincident. [0003] The secret sharing algorithm in visual cryptography is to encode the secret image into several images called Shares by pixels. The black and white pixels in the Shares are ...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06T9/00
Inventor 唐聃于瀛舒红平高燕范迪
Owner CHENGDU UNIV OF INFORMATION TECH
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com