Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

A Decentralized Adaptive Tracking Control Method Based on Extreme Learning Machine

An extreme learning machine and self-adaptive tracking technology, applied in self-adaptive control, general control system, control/regulation system, etc., can solve problems such as slow speed, cumbersome repeated differential operation process of virtual controller, complexity explosion, etc., to achieve Good calculation efficiency, avoiding the effect of complexity explosion problem

Active Publication Date: 2022-04-01
NANJING UNIV OF POSTS & TELECOMM
View PDF4 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0004] (1) Many literatures assume that the state of the system is known, which is not realistic in the actual interconnected system. For the unmeasurable state in the interconnected system, most of the current literature uses the construction of observers to estimate the state. And most of them are fuzzy observers or neural network observers, which are not fast
[0005] (2) The design of the tracking error is also designed using the traditional adaptive inversion control method, which will make the subsequent repeated differential calculation process for the virtual controller quite cumbersome, that is, it will lead to the complexity explosion problem

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • A Decentralized Adaptive Tracking Control Method Based on Extreme Learning Machine
  • A Decentralized Adaptive Tracking Control Method Based on Extreme Learning Machine
  • A Decentralized Adaptive Tracking Control Method Based on Extreme Learning Machine

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

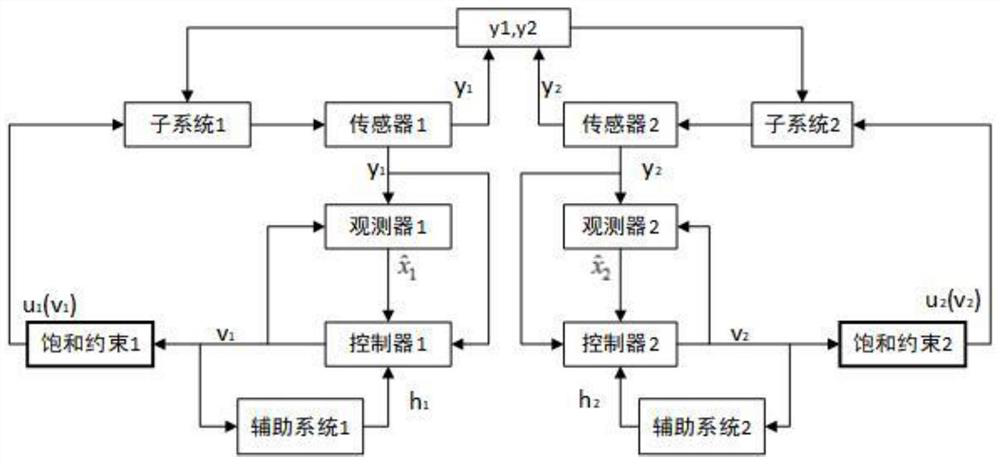
[0066] refer to figure 1 , which is the first embodiment of the present invention, provides a distributed adaptive tracking control method based on an extreme learning machine, specifically including:

[0067] S1: Based on the uncertain nonlinear interconnected system of N subsystems, the interconnected system model is established by using the approximation principle of the extreme learning machine. It should be noted that the uncertain nonlinear interconnection system of N subsystems includes:

[0068]

[0069]

[0070]

[0071] the y i =x i,1

[0072] Where, i=1,...,N, l=2,...,n i -1, respectively represent the state and output of the i-th subsystem, f i,l ( ) represents an unknown smooth nonlinear function, H i,l ( ) represents the unknown interconnection between subsystems, which is also a smooth function, v i is the controller input to be designed, u i ( ) represents the system control input affected by the saturation nonlinearity;

[0073]

[0074]...

Embodiment 2

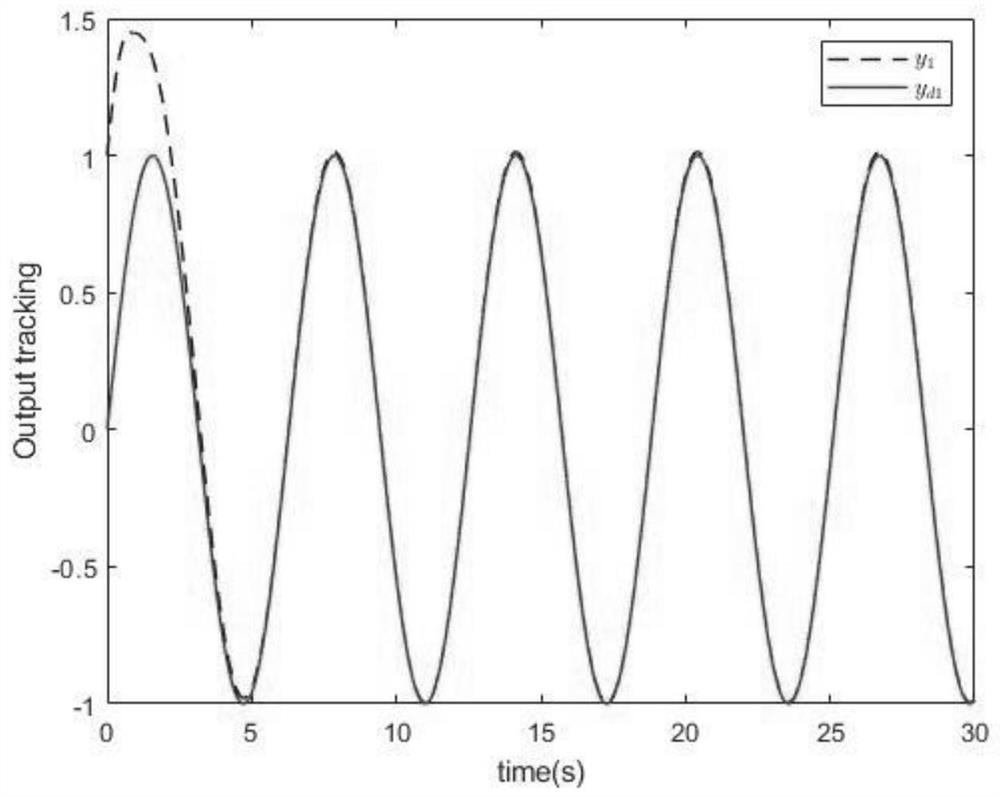
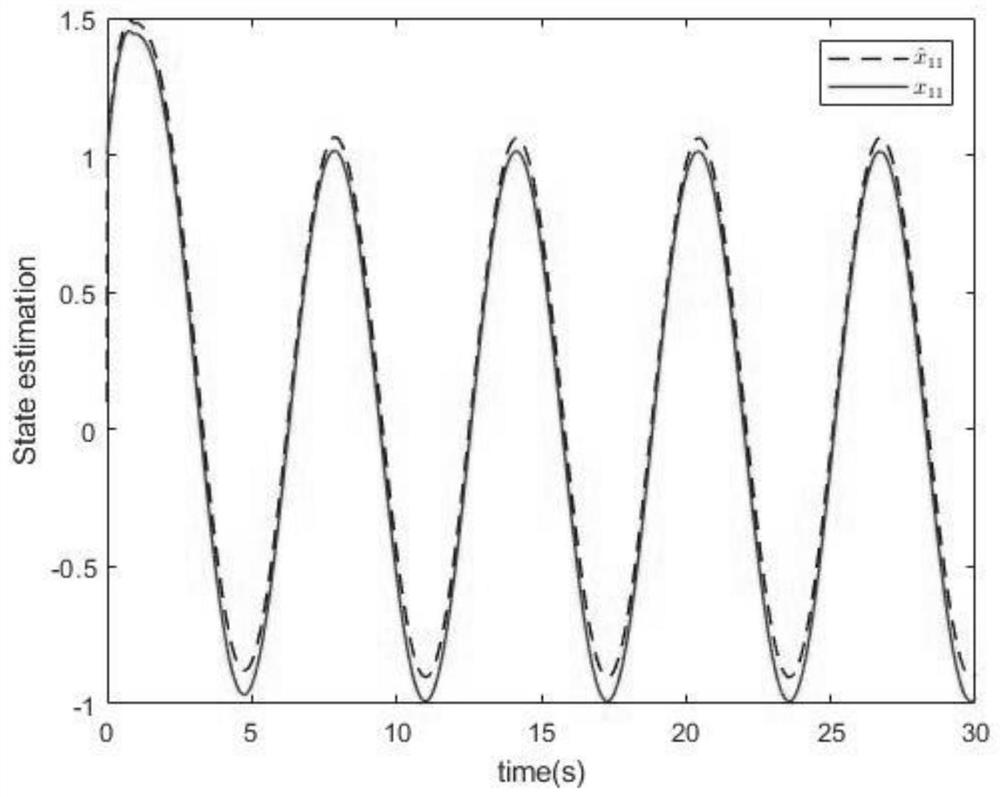
[0121] refer to Figure 1 to Figure 11 , which is the second embodiment of the present invention. What this embodiment is different from the first embodiment is that it provides an experimental test of a decentralized adaptive tracking control method based on extreme learning machines, which specifically includes:

[0122] In this embodiment, in order to verify the stability of the controller provided in Embodiment 1, this embodiment uses Lyapunov stability analysis theory to verify the effectiveness of the controller of the present invention, as follows,

[0123] For all interconnected systems, define the Lyapunov function:

[0124]

[0125] Derivation of the above formula can get

[0126]

[0127]

[0128] It can be obtained from the above formula that the defined Lyapunov function is eventually uniformly bounded, the system model used in the present invention is stable, and the controller designed in the present invention is effective.

[0129] In this embodiment...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a decentralized adaptive tracking control method based on an extreme learning machine, which includes, based on an uncertain nonlinear interconnection system of N subsystems, using the approximate principle of an extreme learning machine to establish an interconnection system model; according to the interconnection system The model construction is based on the state observer of the extreme learning machine to estimate the state and obtain the observation error; the auxiliary system is introduced to deal with input saturation, and the tracking error equation is established according to the dynamic surface control technology; the virtual controller and the adaptive rate are combined with the inversion control technology to obtain the actual controller. The present invention uses the dynamic surface control technology to design the tracking error, which avoids the complexity explosion problem caused by repeated differential operations on the virtual controller. On the other hand, the present invention utilizes the state observer based on the extreme learning machine, Extreme learning machine algorithms have better computational efficiency in terms of learning speed and generalization ability.

Description

technical field [0001] The invention relates to the technical field of distributed control of nonlinear interconnected systems, in particular to a distributed adaptive tracking control method based on an extreme learning machine. Background technique [0002] With the continuous expansion of the scale of modern control system, its structure is also more and more complex. For this kind of complex large-scale system with multi-subsystem interconnection characteristics, the single system modeling method is no longer applicable. Each subsystem has its own control requirements and dynamic characteristics, but they are mutually influential. Therefore, it is an effective method to use interconnected system modeling for such complex large systems. In the past, it was difficult to design reliable control schemes for high-dimensional nonlinear systems because interconnected subsystems lacked the computing power required by a single centralized controller. Under these constraints, d...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Patents(China)
IPC IPC(8): G05B13/04
CPCG05B13/042
Inventor 张忠洋高志峰王邢波赵静林金星
Owner NANJING UNIV OF POSTS & TELECOMM
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com