Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Approximate calculation method for weibull type spare part demanded quantity by means of characteristic numbers

A technology of Weibull type and characteristic number, which is applied in the field of calculation of spare parts demand, can solve the problems of complex multiple convolution form, complex calculation process, large error, etc., and achieve the effect of saving the iterative process

Active Publication Date: 2017-09-29
NAVAL UNIV OF ENG PLA
View PDF1 Cites 26 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

Because the multiple convolution form of the Weibull distribution is extremely complex, it is difficult to obtain the numerical integration result of its multiple convolution
Therefore, in engineering, approximate methods are generally used to calculate the demand for Weibull-type spare parts (such as exponential approximation, normal approximation), but the approximation methods currently used in engineering have large errors, and the exponential approximation is in the Weibull shape. The calculation effect is good when the parameter is close to 1. The normal approximation is only when the Weibull shape parameter is greater than 3, and the approximate calculation method must be reasonable to achieve a better calculation effect. The above calculation method is not only complicated in the calculation process, but also cannot effectively cover All cases in the range of possible values ​​of the shape parameters, which make it impossible to perform a valid calculation of spare part requirements

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Approximate calculation method for weibull type spare part demanded quantity by means of characteristic numbers
  • Approximate calculation method for weibull type spare part demanded quantity by means of characteristic numbers
  • Approximate calculation method for weibull type spare part demanded quantity by means of characteristic numbers

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

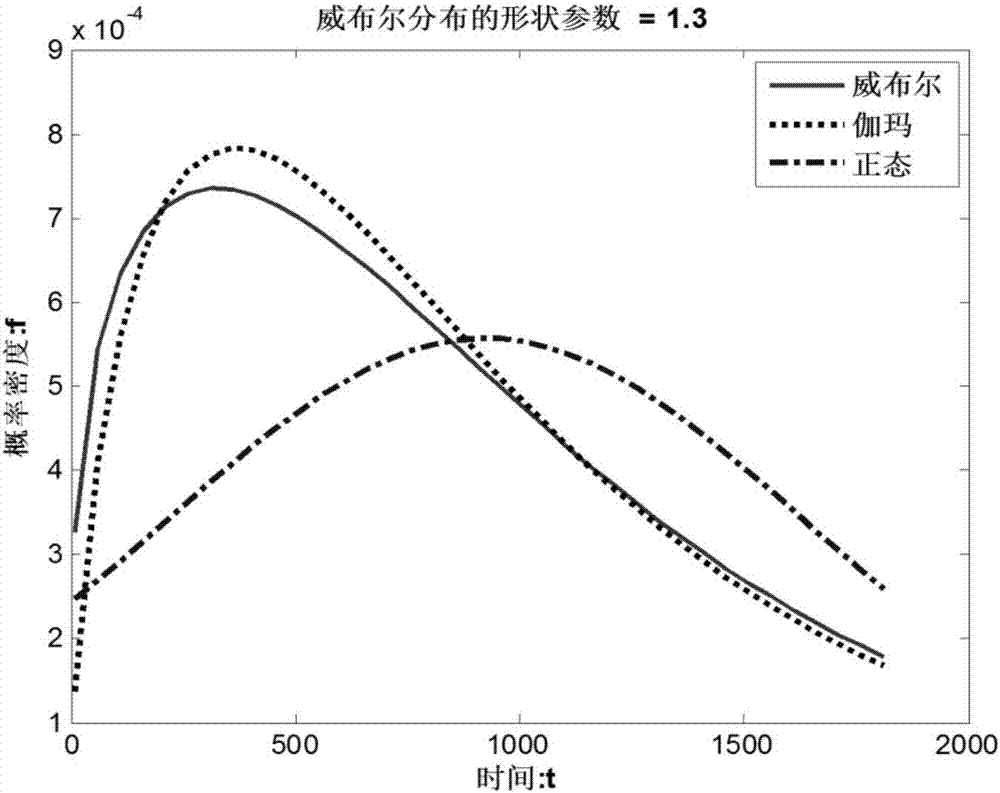
[0073] Example 1: The service life of a certain unit obeys the Weibull distribution W(200,1.3), the guarantee task time is 1000h, the guarantee probability of spare parts is required to be not less than 0.8, and the demand for spare parts is calculated.

[0074] 1) Calculate the parameter α of the gamma distribution g , lambda

[0075] According to the principle that the mean and variance of the gamma distribution are equal to the mean and variance of the Weibull distribution, calculate α g , lambda

[0076]

[0077] 2) Calculate the parameters μ and σ of the normal distribution

[0078] According to the principle that the mean and variance of the normal distribution are equal to the mean and variance of the Weibull distribution, calculate μ and σ

[0079]

[0080] 3) Calculate skewness and kurtosis

[0081] The skewness and kurtosis of the gamma distribution Ga(1.66,0.009) are 1.55 and 3.61;

[0082] Normal distribution N(184.7, 143.3 2 ) skewness and kurtosis are...

Embodiment 2

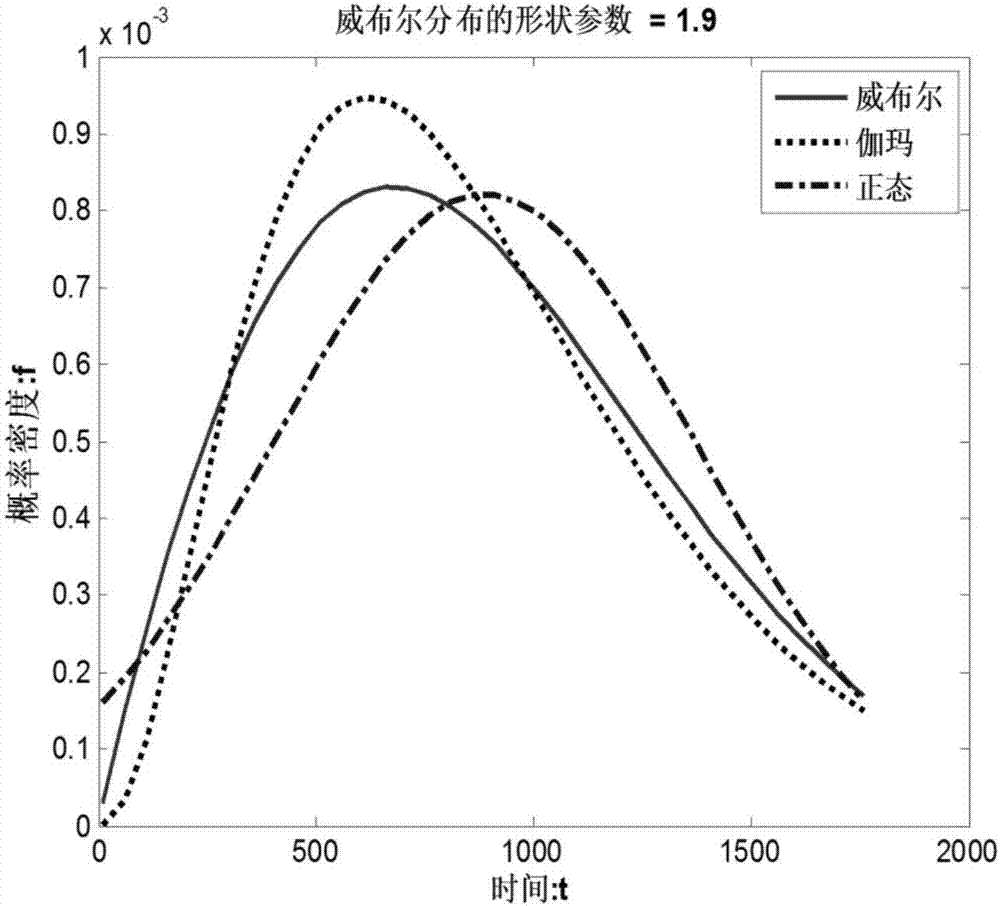
[0091] Example 2: The service life of a certain unit obeys the Weibull distribution W(200,2.9), the guarantee task time is 1000h, and the guarantee probability of spare parts is required to be not less than 0.8, and the demand for spare parts is calculated.

[0092] 1) Calculate the parameter α of the gamma distribution g , lambda

[0093] According to the principle that the mean and variance of the gamma distribution are equal to the mean and variance of the Weibull distribution, calculate α g , lambda

[0094]

[0095] 2) Calculate the parameters μ and σ of the normal distribution

[0096] According to the principle that the mean and variance of the normal distribution are equal to the mean and variance of the Weibull distribution, calculate μ and σ

[0097]

[0098] 3) Calculate skewness and kurtosis

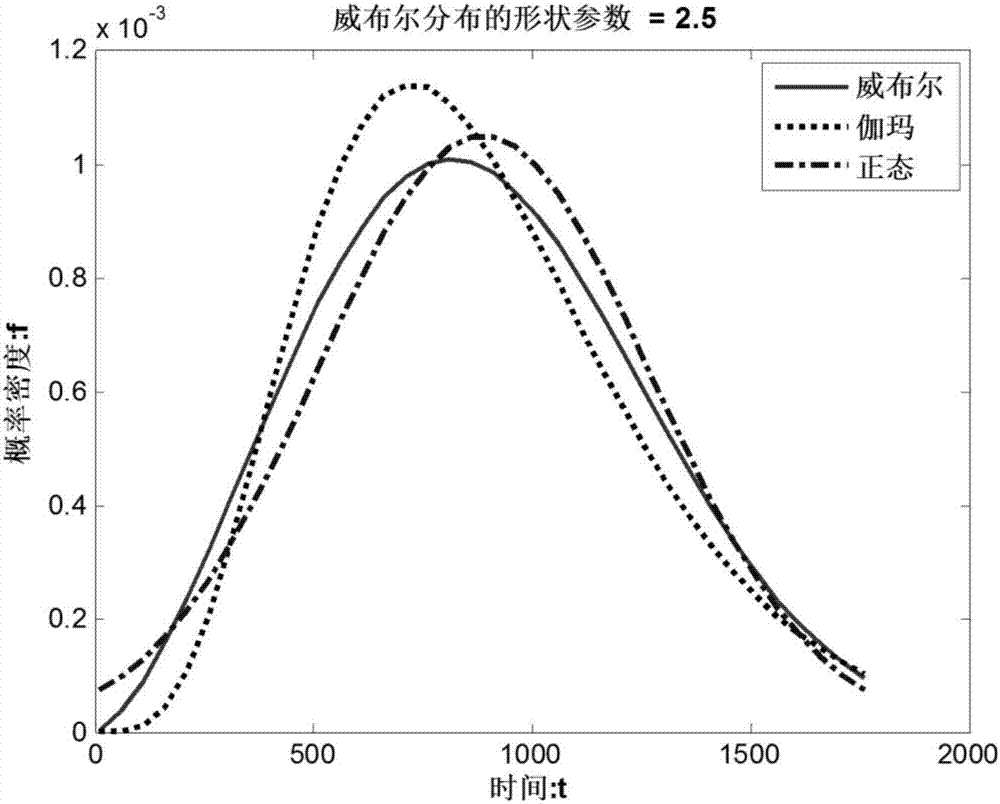
[0099] The skewness and kurtosis of the gamma distribution Ga(7.12,0.04) are 0.75, 0.84;

[0100] Normal distribution N(178.3, 66.8 2 ) skewness and kurtosis are ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses an approximate calculation method for a weibull type spare part demanded quantity by means of characteristic numbers. The approximate calculation method mainly comprises the following steps that 1, gamma distribution parameters alpha g and lambda by means of weibull distribution parameters, and normal distribution parameters mu and sigma are calculated; 2, the skewness and kurtosis are calculated, and the skewness and kurtosis of three distribution characteristic numbers are calculated according to the following formula on the basis of the gamma distribution parameters alpha g and lambda and the normal distribution parameters mu and sigma; 3, the skewness and kurtosis of three distribution characteristic numbers are compared, and if the skewness absolute difference between gamma distribution and weibull distribution is small, the spare part fault probability is calculated according to the method; otherwise, the spare part fault probability Ps is calculated according to other methods. According to the approximate calculation method for the spare part demanded quantity, the calculation process can be simplified, and the approximate calculation precision can be improved.

Description

technical field [0001] The invention belongs to the field of spare part demand calculation, in particular to an approximate calculation method of Weibull type spare part demand using characteristic numbers. Background technique [0002] Weibull distribution is often used to describe the life of components that fail due to gradual aging, and components with this Weibull distribution are Weibull-type units. Weibull type units are mainly suitable for electromechanical parts, such as: ball bearings, relays, switches, circuit breakers, certain capacitors, electron tubes, magnetrons, potentiometers, gyroscopes, electric motors, aircraft generators, batteries, hydraulic pumps, air turbines Engines, gears, valves, material fatigue parts, etc. [0003] When the above-mentioned units are used in various systems, it is necessary to pre-evaluate and calculate the demand for spare parts. Spare parts are the material conditions to ensure the sustainable operation of equipment in consider...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06F17/18
CPCG06F17/18
Inventor 邵松世翟亚利李华王俊龙
Owner NAVAL UNIV OF ENG PLA
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com