Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Linear fitting method and system and storage medium

A linear fitting and square technology, applied in the field of data fitting, can solve the problem of inaccurate data fitting results, achieve the effect of good robustness and improve efficiency

Inactive Publication Date: 2020-06-19
XI AN JIAOTONG UNIV
View PDF11 Cites 2 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0016] The purpose of the present invention is to solve the problem of inaccurate fitting results caused by noise in the data during linear fitting, and provide a linear fitting method, system and storage medium, which can significantly improve the accuracy of data fitting and the efficiency of data fitting

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Linear fitting method and system and storage medium
  • Linear fitting method and system and storage medium
  • Linear fitting method and system and storage medium

Examples

Experimental program
Comparison scheme
Effect test

Embodiment

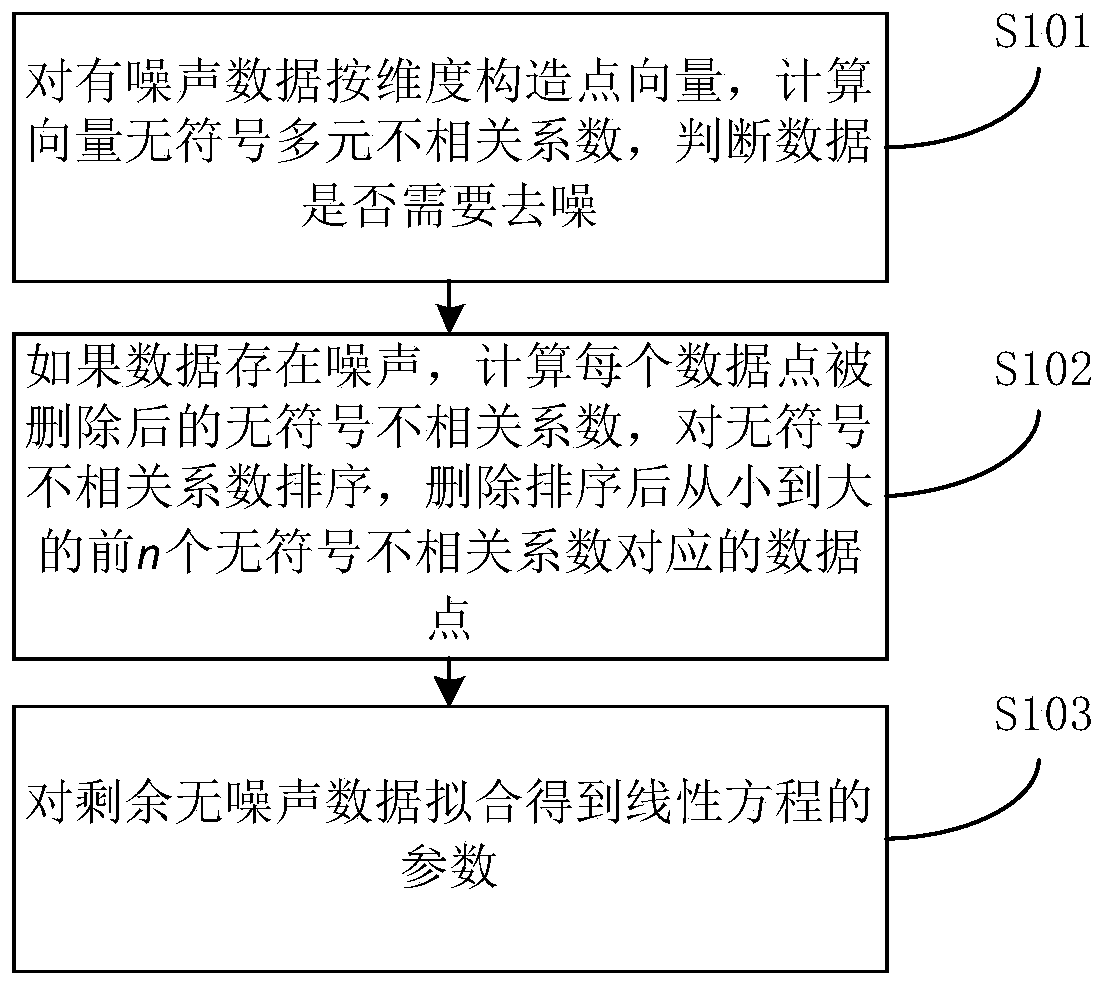
[0069] see figure 1 , the feasibility of the present invention will be described below by taking the three-dimensional data point when k=3 as an example, and an application example will be given.

[0070] Step 1. For a data point that needs to be fitted, input a 3D point such as figure 2 As shown, the number of data points m=1810;

[0071] Step 2: Construct the vector x according to the dimensions of the input data with m noises 0 ,x 1 ,x 2 ;

[0072] Step 3, calculate the vector x 0 ,x 1 ,x 2 The Pearson correlation coefficient between the two constitutes the correlation matrix R;

[0073] Step 4, calculate the square ω of the unsigned uncorrelated coefficient between vectors according to formula (3) 2 ;

[0074] Step 5, determine the square of the unsigned multivariate uncorrelated coefficient ω 2 Is it greater than the threshold ω t , if ω is less than the threshold δ, there is no noise in the data, and the data is directly fitted; if ω is greater than or equal...

Embodiment 2

[0097] The present invention uses the square of the uncorrelated coefficient of the attribute vector, and uses the square of the uncorrelated coefficient of the attribute vector to judge the strength of the noise. In fact, since minimizing the square of the unsigned uncorrelated coefficient is equivalent to maximizing the square of the unsigned uncorrelated coefficient, which is equivalent to minimizing the unsigned uncorrelated coefficient, which is equivalent to minimizing the unsigned uncorrelated coefficient plus an arbitrary constant, Equivalent to minimizing the unsigned uncorrelated coefficient plus any constant, equivalent to minimizing the unsigned uncorrelated coefficient multiplied by any constant greater than zero, etc., and also equivalent to maximizing the unsigned uncorrelated coefficient multiplied by any less than zero Constants, etc., so the corresponding equivalent expressions can be given with reference to the steps in the claims. For example, for the unsign...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a linear fitting method and system and a storage medium, and the method provided by the invention can delete noise points in batch, and greatly improves the denoising efficiency. When the data noise is removed, compared with a traditional method, the noise existing in the data can be rapidly deleted by utilizing the correlation of the data, and the fitting method still hasgood robustness on the premise that a large amount of noise exists in the data.

Description

technical field [0001] The invention belongs to the field of data fitting, and in particular relates to a linear fitting method and system and a storage medium. Background technique [0002] At present, data noise is widespread. For example, the data acquired by sensors, due to the acquisition accuracy of the sensors, or the external interference received during the interaction with the data acquisition equipment, the acquired data often contains noise, resulting in data analysis results inaccurate. [0003] In statistics, linear regression is a type of regression analysis that models the relationship between one or more independent and dependent variables using the least squares function known as the linear regression equation. Such functions are linear combinations of one or more model parameters that become regression coefficients. [0004] assuming x 1 ,x 2 ,...x d , d factors, the following linear relationship is considered: [0005] y=β 0 +β 1 x 1 +β 2 x 2 +...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G06F17/11G06F17/16
CPCG06F17/11G06F17/16
Inventor 汪建基丁健郑南宁
Owner XI AN JIAOTONG UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com