Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Characteristics description method for plane geometric shape

A technology of plane geometry and feature description, applied in the field of pattern recognition, can solve problems such as large amount of calculation, judgment error, and failure of judgment error, etc., and achieve the effect of simple calculation method, accurate description and fast speed

Active Publication Date: 2009-07-01
NEW FOUNDER HLDG DEV LLC +1
View PDF0 Cites 15 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0009] Aiming at the problems existing in the prior art, the first purpose of the present invention is to provide a method for describing the features of the plane geometry, which is used to solve the problem in the prior art that some local features of the plane geometry cannot be accurately described, and the amount of calculation is large. and the flaw of judgment error
[0010] The second purpose of the present invention is to provide another feature description method for plane geometric shapes, which is used to solve the problems in the prior art that cannot accurately describe a certain local feature of plane geometric shapes, has a large amount of calculation, has judgment errors, and cannot accurately describe a certain local feature of a plane geometric shape. Deficiencies in the description of common features of similar planar geometric shapes

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Characteristics description method for plane geometric shape
  • Characteristics description method for plane geometric shape
  • Characteristics description method for plane geometric shape

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

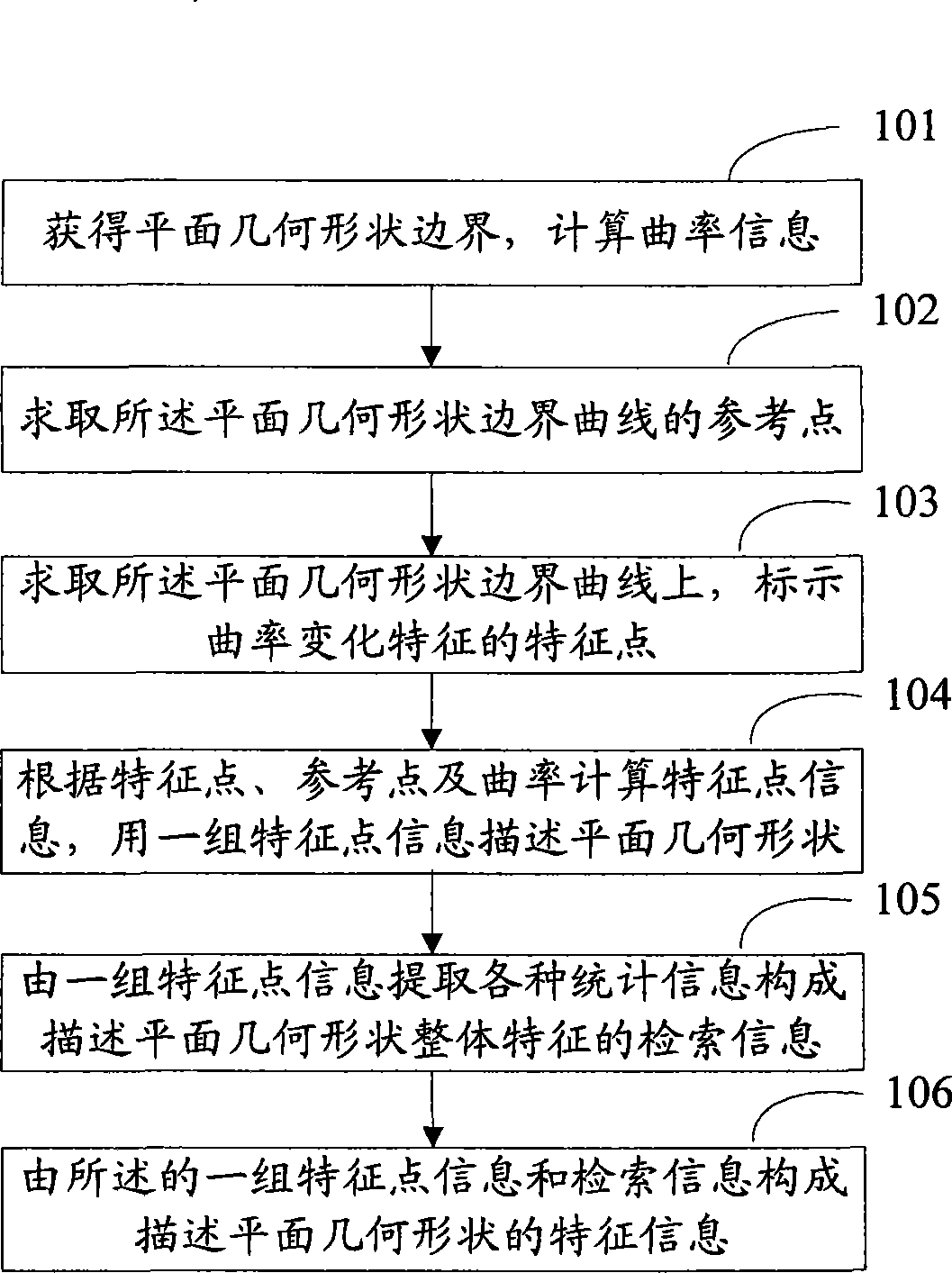
[0048] like figure 1 As shown, the present embodiment provides a feature description method of a plane geometry, comprising the following steps:

[0049] Step 101, calculate the boundary curve of the obtained plane geometry according to a certain direction, and obtain the curvature information of each point on the boundary curve;

[0050] Curvature can be calculated for boundary curves in a clockwise or counterclockwise direction, starting from any point. According to the definition of curvature, it can be known that the curvature of the boundary curve is calculated along different directions, and the sign of the result is different, but the absolute value is the same. After the calculation direction is selected, it cannot be changed; at the connection point of two piecewise continuous functions, when the two functions are at the connection point and the derivative is discontinuous, the curvature of the point is positive or negative infinity.

[0051] Step 102, obtaining the r...

Embodiment 2

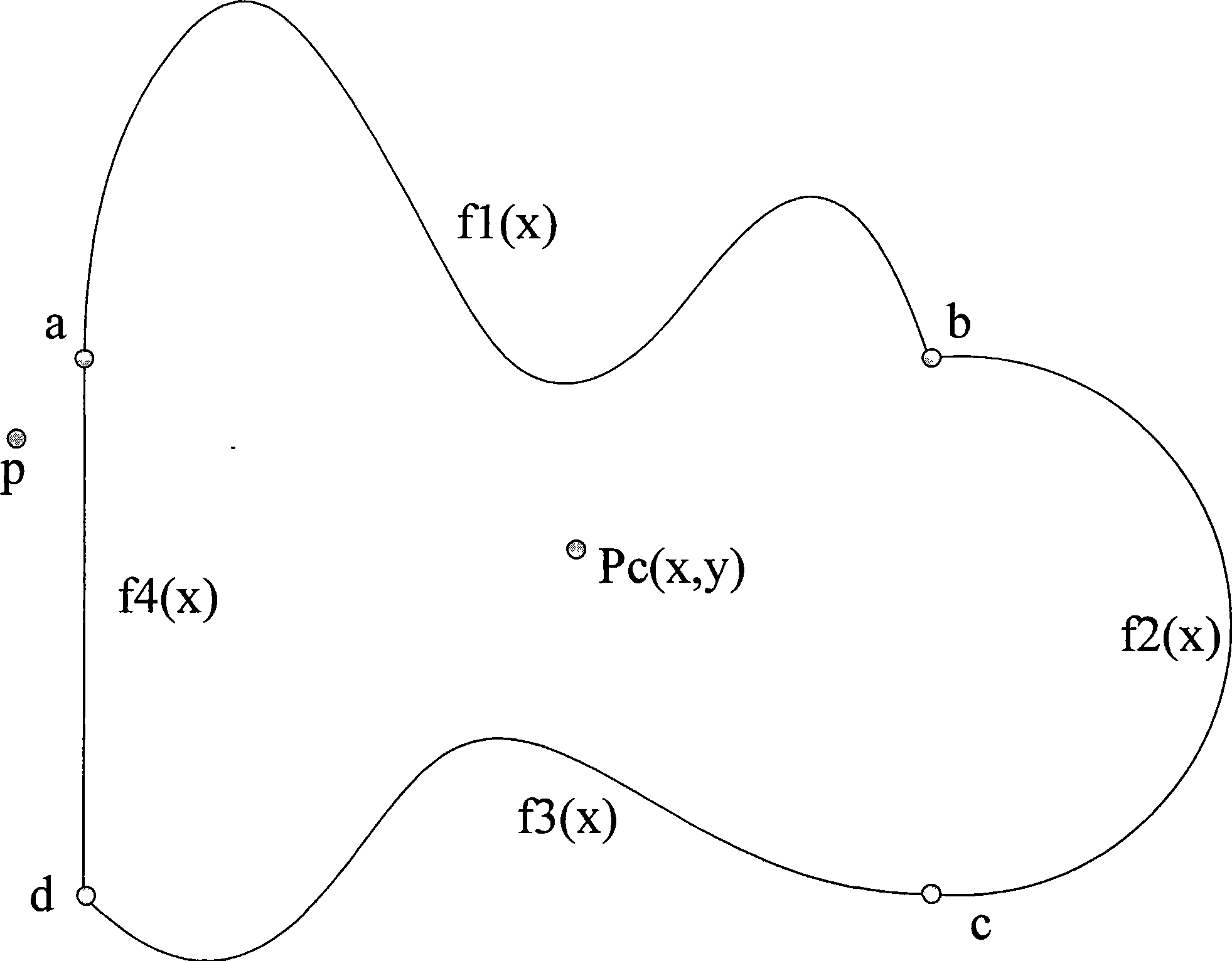
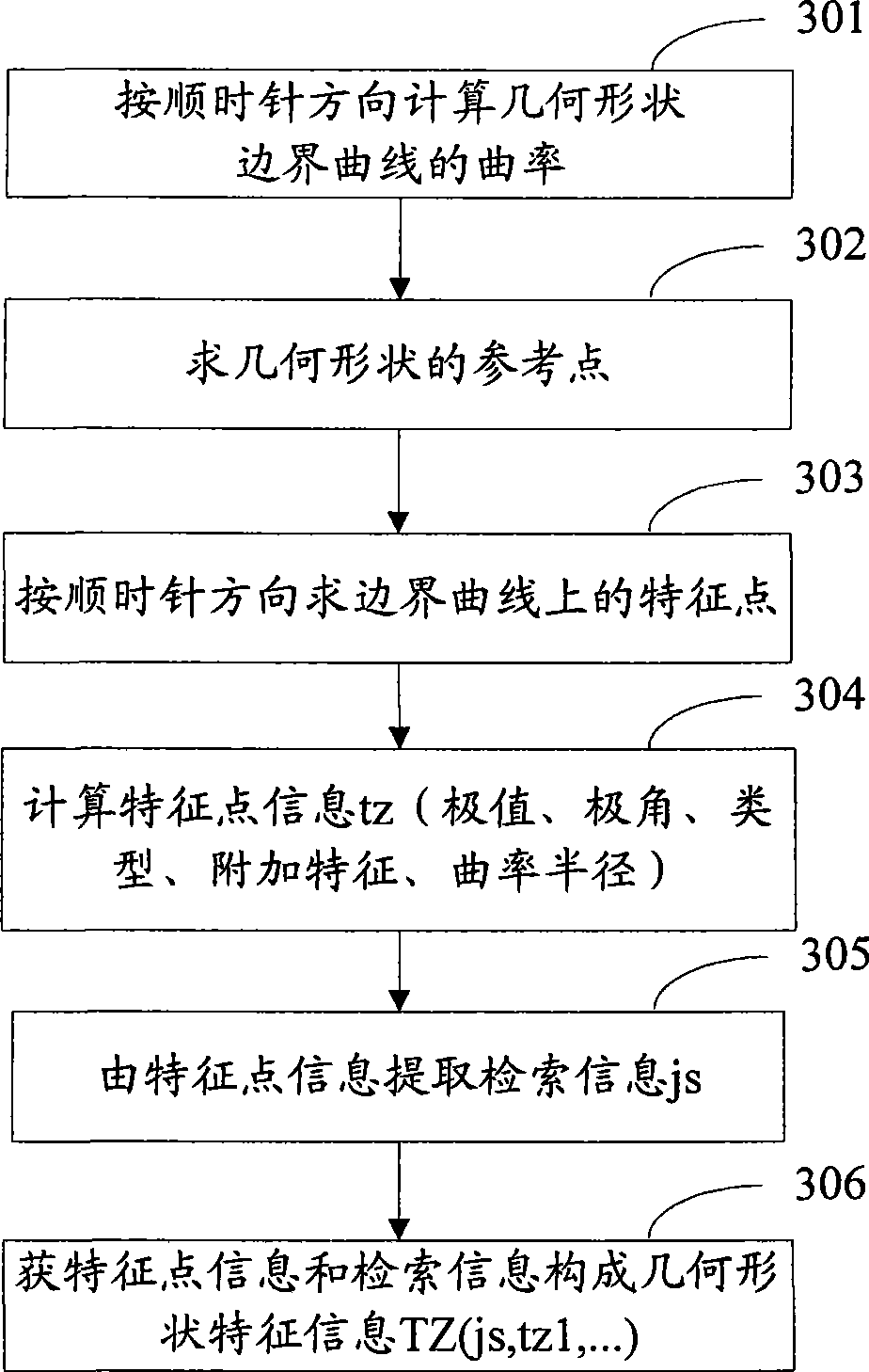
[0087] The embodiment of the present invention adopts the figure 2 The geometric shape shown obtains feature information to realize the feature description of this geometric shape. Mathematical description Y=F(X) of the boundary curve of any geometric shape. Without loss of generality, the boundary curve is composed of n piecewise continuous functions {y=f1(x), y=f2(x)...y=fn(x)}. The process of feature information acquisition method is as follows: image 3 shown, including the following steps:

[0088] Step 301: get figure 1 The mathematical description of the boundary curve of the shown geometric shape Y=F(X), such as figure 1 As shown, the boundary curve is composed of 4 piecewise continuous functions {y=f1(x), y=f2(x), y=f3(x), y=f4(x)}; where f2(x) is The arc of a semicircle, f4(x) is a straight line; starting from any point p on the boundary curve, calculate the curvature on the boundary curve clockwise, according to the definition of curvature, calculate the curva...

Embodiment 3

[0103] by right Figure 5 The characteristic information of the geometric shape formed by the shown unclosed curve is obtained, which shows that the plane geometric shape can be composed of any combination of closed curve and unclosed curve; the process of obtaining the characteristic information is completely consistent with the above steps, the only difference is whether Closed curve constitutes a mathematical description of geometric shape, let Figure 5 The mathematical description function of the shown closed curve is y=fa(x), and the function is defined in Figure 5 In (a, b) between two points. Define another function y=fb(x) between two points (a, b), and there is fb(x)=fa(x), so fa(x) and fb(x) constitute a closed curve, so , the feature information of this geometric shape can be obtained by the same method; similarly, the feature information of the geometric shape composed of any combination of closed curves and unclosed curves can also be obtained by the same meth...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a characteristic description method of a plane geometry plane, which belongs to the basic technique in the pattern recognition field. The method comprises extracting geometric figure outline characteristic points through the mode of simulating an image thinking memory geometric figure, utilizing a characteristic point polar coordinate vector and an additional curvature information as a core to form characteristic information for describing a plane geometry shape. The characteristic description method achieves the correct description to the main characteristics of plane geometry shapes. The calculating method of characteristic information is simple, and calculating quantity is greatly reduced. Characteristic information which is accurately described can be got under the complex circumstance of rotating, translating and scaling.

Description

technical field [0001] The invention relates to the field of pattern recognition, in particular to a feature description method of plane geometric shapes. Background technique [0002] Geometric shape recognition is the basic technology of graphics and image recognition in the field of pattern recognition. In artificial intelligence, computer vision, robotics, image target recognition, optical character reader (Optical Character Reader, OCR), military and other high-tech fields, geometric shape recognition technology plays a key role. Geometric shape recognition is realized based on the mathematical description method of shape features and feature information extraction technology. [0003] In the prior art, the mathematical description of graphics is mainly based on two theoretical systems. One is the Fourier descriptor theory based on integral transform, which transforms the image information from the time domain to the frequency domain, and uses the obtained frequency d...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G06K9/52G06K9/46
Inventor 曾培祥
Owner NEW FOUNDER HLDG DEV LLC
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com