Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Model building method and device for linear scanning polarographic wave

A technology of linear scanning and construction methods, applied in special data processing applications, instruments, electrical digital data processing, etc., can solve problems such as high computational complexity and complex model structure.

Active Publication Date: 2016-06-01
CHANGSHA HASKY ENVIRONMENTAL PROTECTION TECH DEV CO LTD
View PDF4 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0006] The purpose of the present invention is to solve the problem of high calculation complexity due to the complex model structure when the traditional linear scanning polarographic method is used to measure the ion concentration analysis and detection

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
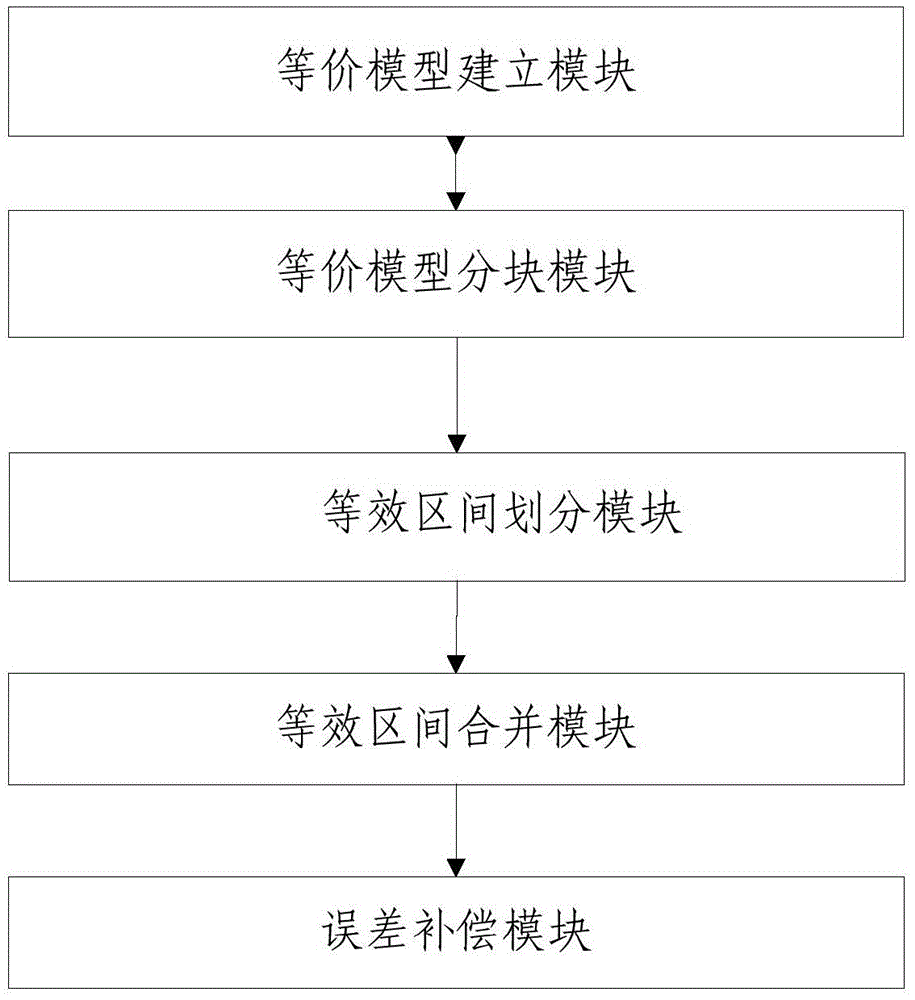
  • Model building method and device for linear scanning polarographic wave
  • Model building method and device for linear scanning polarographic wave
  • Model building method and device for linear scanning polarographic wave

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0053] The symbols and letters used in the formula are described as follows in this embodiment,

[0054] I 1 is the polarographic current; I 2 is the error compensation value; i 1 is the first sub-model, i 2 is the second sub-model; n is the number of electrons in the electrode reaction; F is the Faraday constant; E is the potential value at time t; 0 is the initial scan potential; is the half-wave potential; A is the mercury drop area; O is the depolarizer; C 0 is the initial concentration of depolarizer O in the solution; D O is the diffusion coefficient of the depolarizer O; the diffusion rate Where R represents the gas constant, T is the absolute temperature, v is the electrode potential change rate; t is the voltage sweep time, that is, the mercury drop time, Indicates the time when the scanning voltage reaches the half-wave potential; μ∈[0,1].

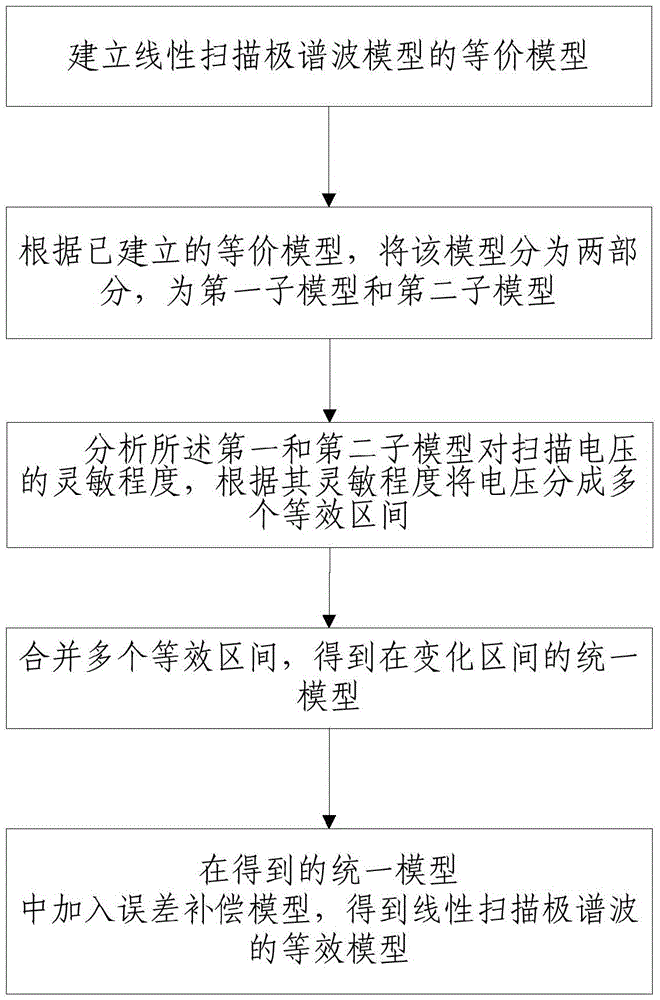
[0055] 1. Establish an equivalent model of the linear sweep polarographic wave model

[0056] The equivalent model es...

Embodiment 2

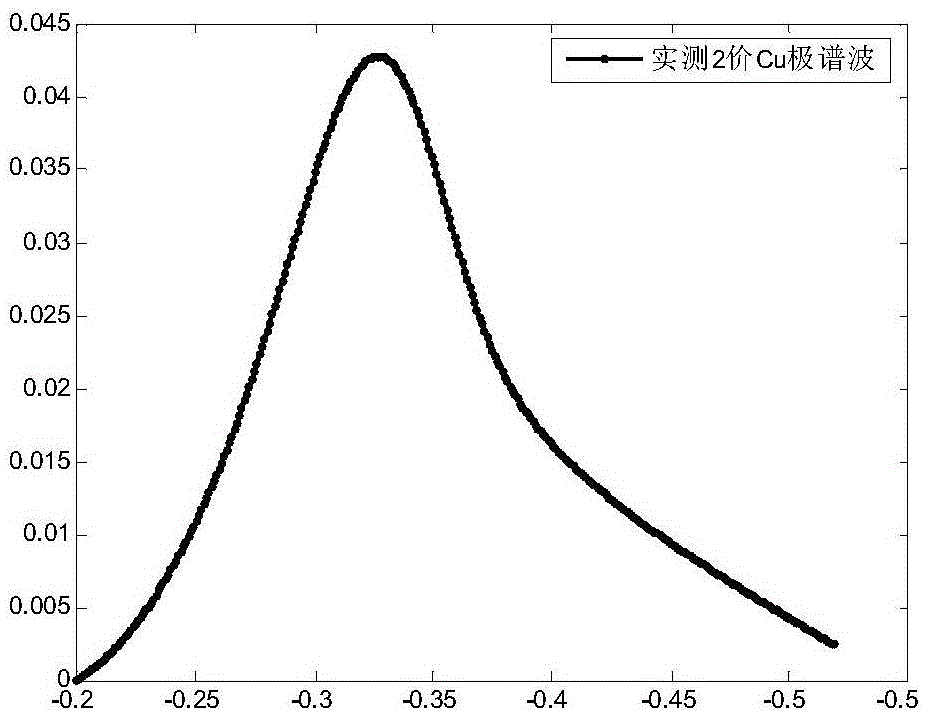
[0123] Using the formula used in Example 1, the specific application is described as follows. Take Cu 2+ 、Cd 2+ 、Co 2+ and Zn 2+ Efficient calculation of linear sweep polarographic wave model as an example, Cu obtained by linear sweep polarography 2+ 、Cd 2+ 、Co 2+ and Zn 2+ The polarographic waves of Figure 3 ~ Figure 6 As shown, the detection temperature is 20°C, and the specific and efficient calculation method for the linear scanning polarographic wave model of these four ions is implemented as follows:

[0124] 1. Determine the value of some fixed parameters

[0125] The linear sweep polarographic wave can be expressed by the Sevcik current formula as:

[0126] I 1 = 1 2 nFAC 0 D o 1 2 ∫ 0 t 1 ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a high-efficiency calculation method for a linear sweep polarography model. A mean value theorem of integrals and a Newton-Leibniz formula are applied to converting an integral part of the linear sweep polarography model, i.e., a Sevcik current formula, into an equivalent model containing no integral terms; the model is divided into three intervals to perform equivalent transformation in the entire change interval by analyzing the characteristics of the equivalent model, and the three intervals are combined by analyzing the characteristics of the equivalent models of the three intervals, so that the unification of the linear sweep polarography interval equivalent models in the entire change interval is realized; meanwhile, considering the error produced in the equivalent transformation process, the linear sweep polarography equivalent model which is formed through linear superposition of two sub-models is finally obtained by adding an error compensation model in the combined model. After testing, the linear sweep polarography model can be accurately calculated, and meanwhile, the parameter identification time is reduced by over 90 percent.

Description

technical field [0001] The invention relates to the field of ion concentration analysis and detection model construction, in particular to a model construction method and device for linear scanning polarographic waves. Background technique [0002] Linear scanning polarography is an important method for ion concentration analysis and detection. It is accurate and simple, and has been widely used, especially suitable for online analysis and detection of metal ions. However, when using this method to determine the concentration of metal ions online, it usually cannot meet the requirements of accuracy and real-time performance at the same time. In order to obtain high-precision analysis results, laboratory offline analysis is usually used, but the lag time is too long to provide real-time information for online analysis. [0003] Real-time analysis is to implant the analysis method into the automatic online analyzer based on the microprocessor to analyze the ion concentration,...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Patents(China)
IPC IPC(8): G06F19/00
Inventor 阳春华王国伟彭雄威王宏善尹睿朱红求李勇刚桂卫华
Owner CHANGSHA HASKY ENVIRONMENTAL PROTECTION TECH DEV CO LTD
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com