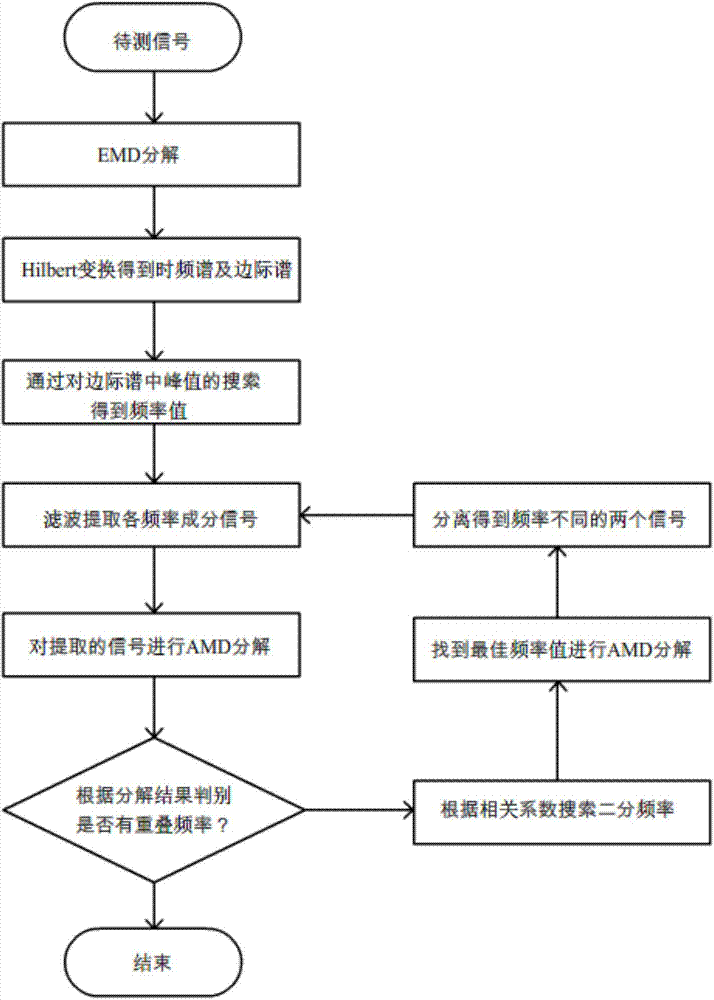
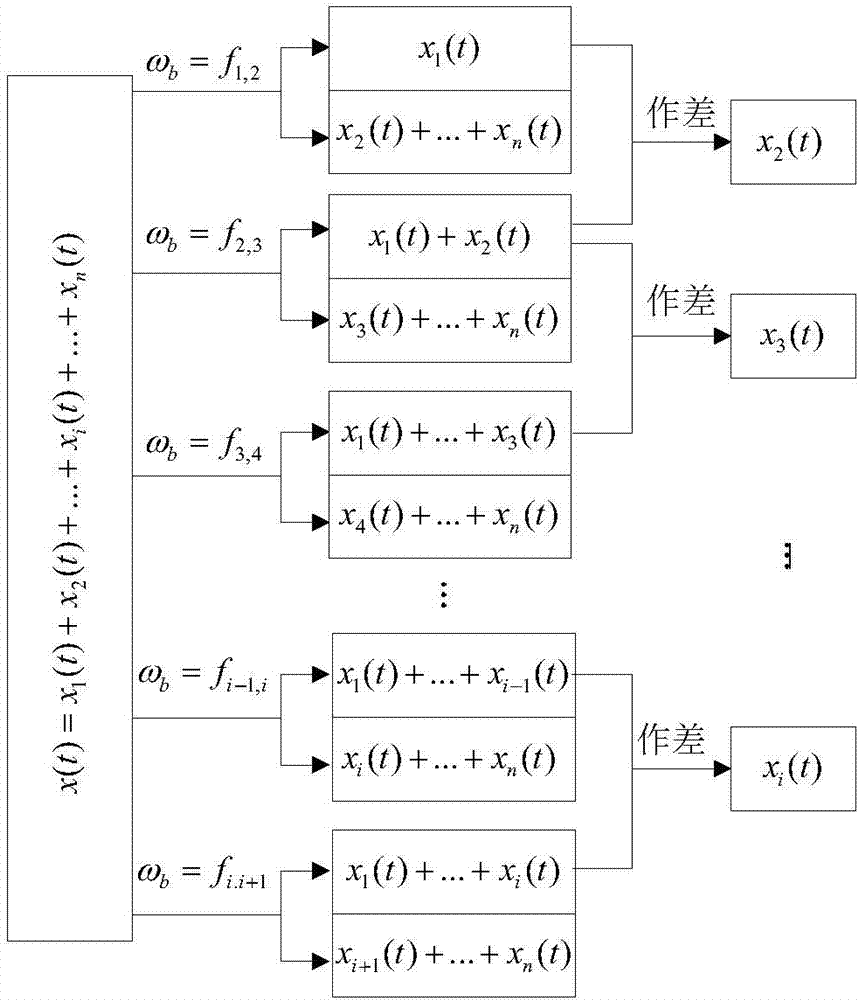
Method for detecting closely spaced frequency components of non-stationary signals
A non-stationary signal and spaced frequency technology, applied in the field of signal processing, can solve problems such as the inability to identify closely spaced frequency components and the difficulty of detecting non-stationary signal closely spaced frequency components
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment 1
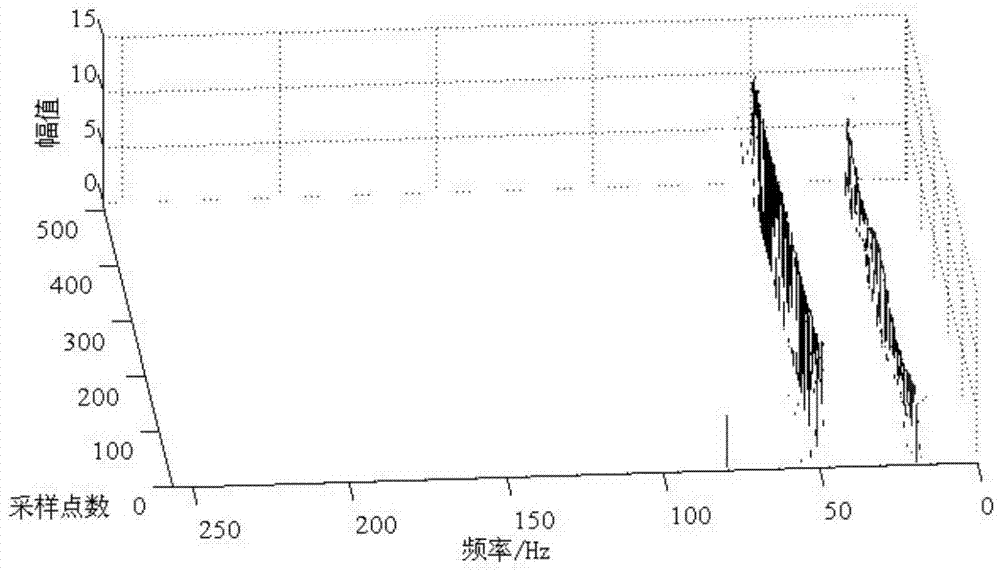
[0082] For the simulation signal x(t)=5sin(40πt)+2sin(44πt)+10sin(100πt), the time domain waveform is as follows image 3 shown.
[0083] After HHT processing the signal, its marginal spectrum is as follows: Figure 7 As shown, two frequency values can be obtained, which are 42π and 100π respectively.
[0084] AMD decomposes the signals with frequency components of 42π and 100π respectively, and the decomposed time domain waveforms are respectively Figure 8 and Figure 10 As shown, the decomposed spectrograms are respectively Figure 9 and Figure 11 shown. From the simulation diagram, it can be seen that the signal with frequency component 42π has frequency aliasing phenomenon, and the signal with frequency component 100π has no frequency aliasing phenomenon.
[0085] The values of the two cross-correlation coefficients obtained by frequency search on the signal with frequency component 42π are shown in Table 1, in the table a 1 Represents the cross-correlation co...
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com