Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

A steady fluctuating wind field simulation method based on root computing and decomposition and a spectral representation method

A simulation method and pulsating wind technology, applied in special data processing applications, instruments, electrical digital data processing, etc., can solve problems such as Cholesky decomposition of power spectrum matrix

Inactive Publication Date: 2017-09-15
HOHAI UNIV
View PDF2 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0004] Purpose of the invention: the purpose of the present invention is to solve the problem that the power spectrum matrix cannot be decomposed by Cholesky at local frequency points in the wind field simulation, and to provide a method to make the spectral representation applicable to Simulation Method of Power Spectrum Matrix Based on Square Root Decomposition Spectrum Representation for Stationary Fluctuating Wind Field in More Situations

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • A steady fluctuating wind field simulation method based on root computing and decomposition and a spectral representation method
  • A steady fluctuating wind field simulation method based on root computing and decomposition and a spectral representation method
  • A steady fluctuating wind field simulation method based on root computing and decomposition and a spectral representation method

Examples

Experimental program
Comparison scheme
Effect test

Embodiment Construction

[0027] The technical solution of the present invention will be further described below in conjunction with the accompanying drawings.

[0028] This implementation method is illustrated by simulating a one-dimensional three-variable random wind field as an example:

[0029] (1) The simulated points are three points distributed along the same vertical line, and their heights are 100m, 110m, and 120m respectively.

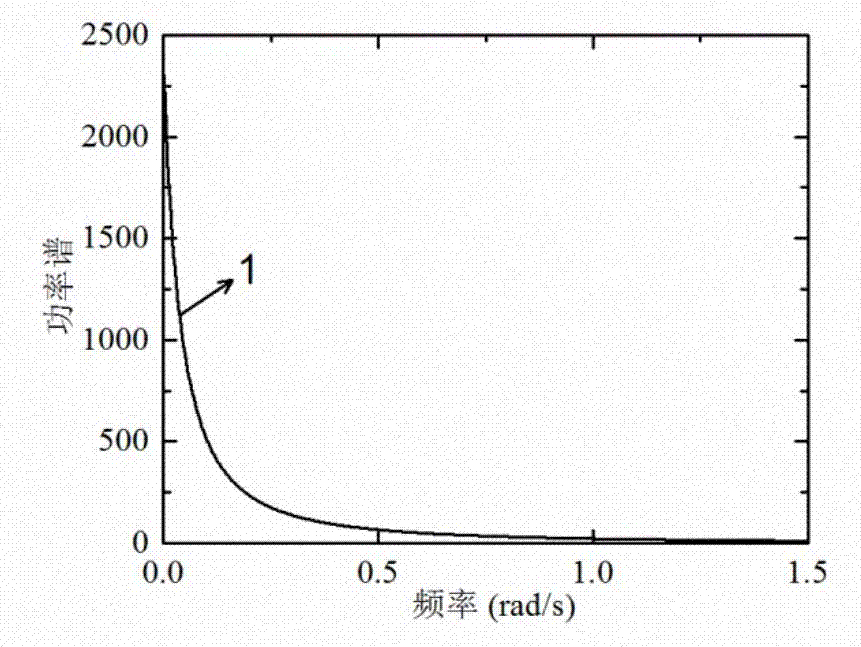
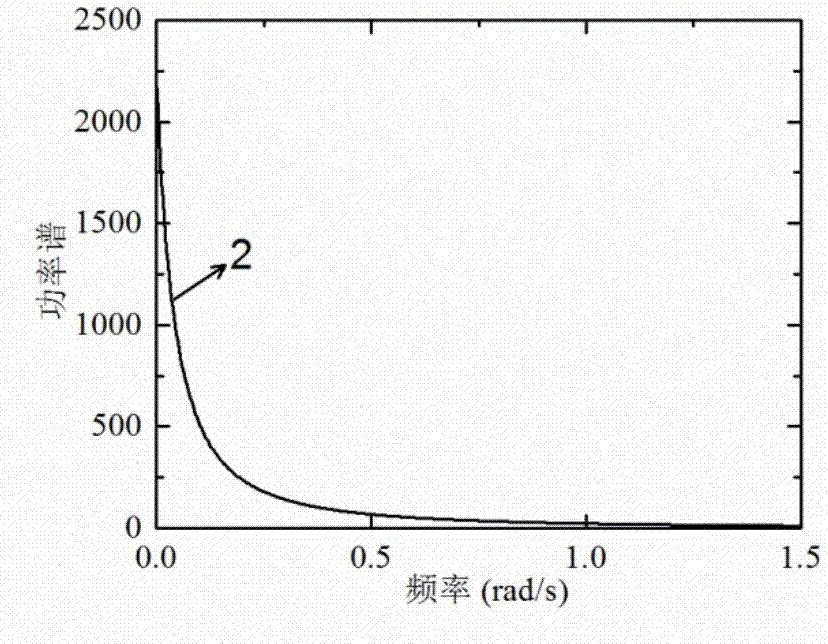
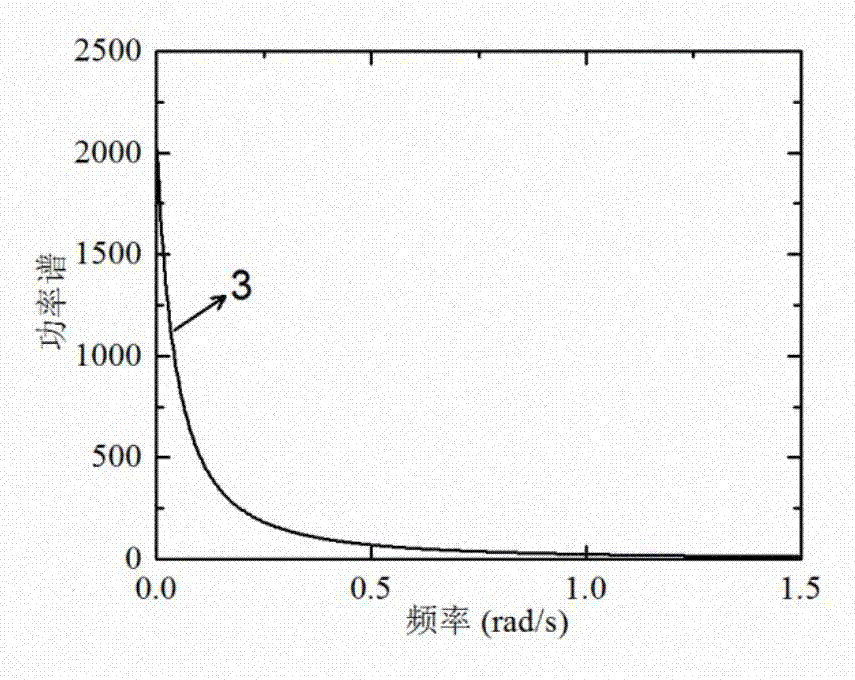
[0030] (2) Assume that the autopower spectrum of each point is:

[0031]

[0032] Among them, z j is the height of point j, u* is the shear wave velocity, U(z j ) is at height z j The average wind speed at , which can be obtained by the following empirical formula:

[0033]

[0034] where k is the von Karman constant and the roughness length z 0 Take 2.0m; get the average wind speed at each point height as:

[0035] U(z 1 =120m)=64.4m / s

[0036] U(z 2 =110m)=63.0m / s

[0037] U(z 3 =100m)=61.5m / s

[0038] Based on the above parameters, the obtained au...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention provides a steady fluctuating wind field simulation method based on root computing and decomposition and a spectral representation method. The method comprises the steps of: (1) discretizing a steady fluctuating wind field and determining the number N of discrete points needed for simulating a wind process, so that the random wind field simulation is converted to one-dimensional and multi-variable random process simulation; (2) determining a power spectrum matrix of the random wind field; (3) performing matrix root computing and decomposition on the power spectrum matrix to obtain a new multi-dimensional Gaussian power spectrum matrix; (4) randomly generating N2 random phase angles distributed uniformly in a [0, 2pi] range; (5) using a trigonometric function combination method based on a spectral representation method and generating a wind process time course of each point. The method uses an root computing and decomposition method to analyze power spectrum matrixes so that the problem that Cholesky decomposition cannot be implemented under some conditions is solved; after being decomposed by the decomposition method, the matrixes have better symmetry and the problem is solved that many zero elements emerge through Cholesky decomposition, so that the physical significance of trigonometric function combination item coefficients is more reasonable.

Description

technical field [0001] The invention relates to a wind-resistant design method for civil engineering structures, in particular to a method for simulating a steady fluctuating wind field based on a square root decomposition spectrum representation. Background technique [0002] In the wind-induced dynamic response analysis of long-span bridges and high-rise buildings, due to the need to consider the nonlinearity of structure and aerodynamics, the traditional frequency-domain analysis methods cannot meet the requirements, and time-history-based nonlinear dynamic response analysis is required. In the nonlinear time-history analysis, simulating the fluctuating wind field at the location of the structure is a basic link, and also the most important link. Among many simulation methods, the spectral representation method is relatively simple, that is, it is only obtained through the superposition simulation of trigonometric functions, and has rigorous derivation as a theoretical su...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G06F17/50
CPCG06F30/13G06F30/20
Inventor 吴勇信高玉峰李睿张宁张飞
Owner HOHAI UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com