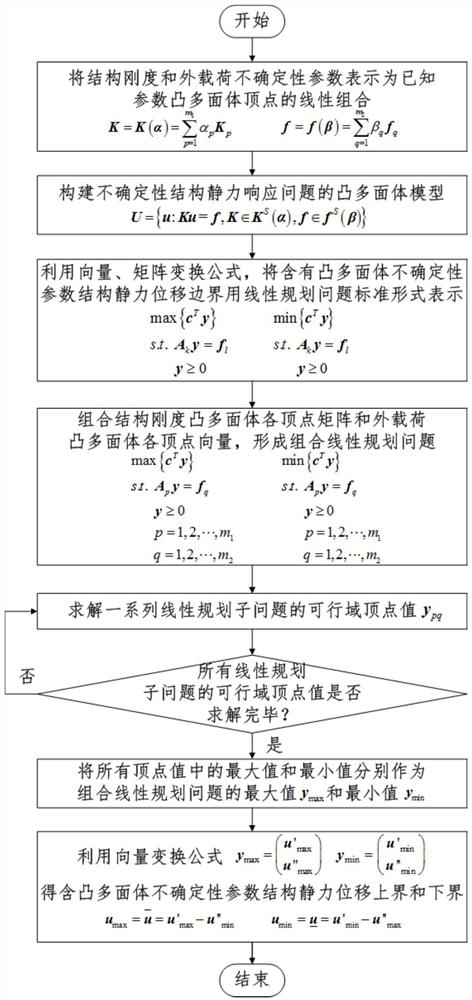
A Linear Programming-Based Method for Estimating the Static Displacement Boundary of Structures Containing Convex Polyhedral Uncertainty Parameters
A convex polyhedron and uncertainty technology, applied in the field of uncertainty propagation analysis technology under parameter conditions, can solve the problem of excessively wide boundaries, ignore the correlation between uncertain variables, and provide objective and effective methods for structural reliability assessment and design. Data and other issues
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment
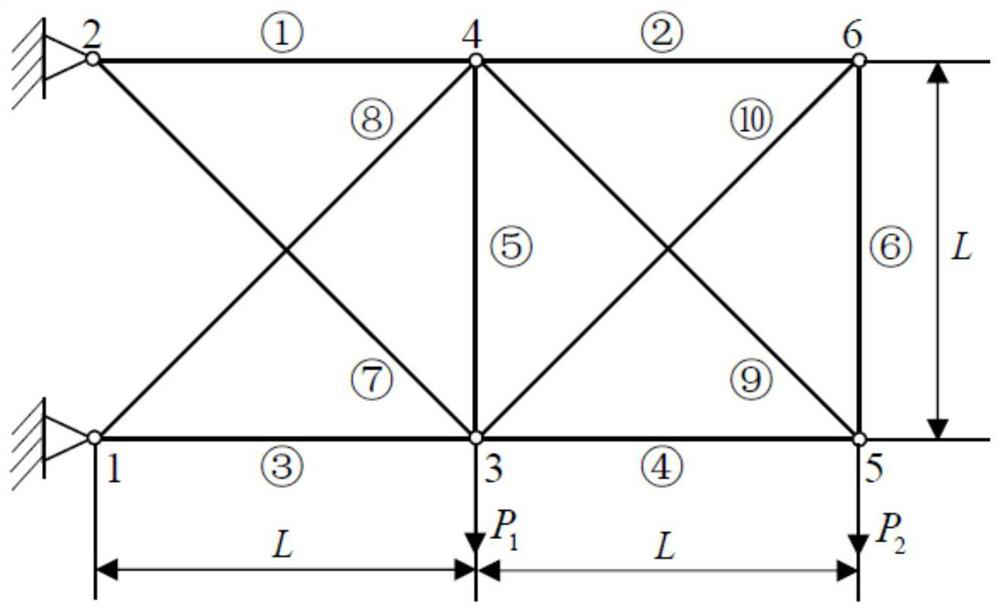
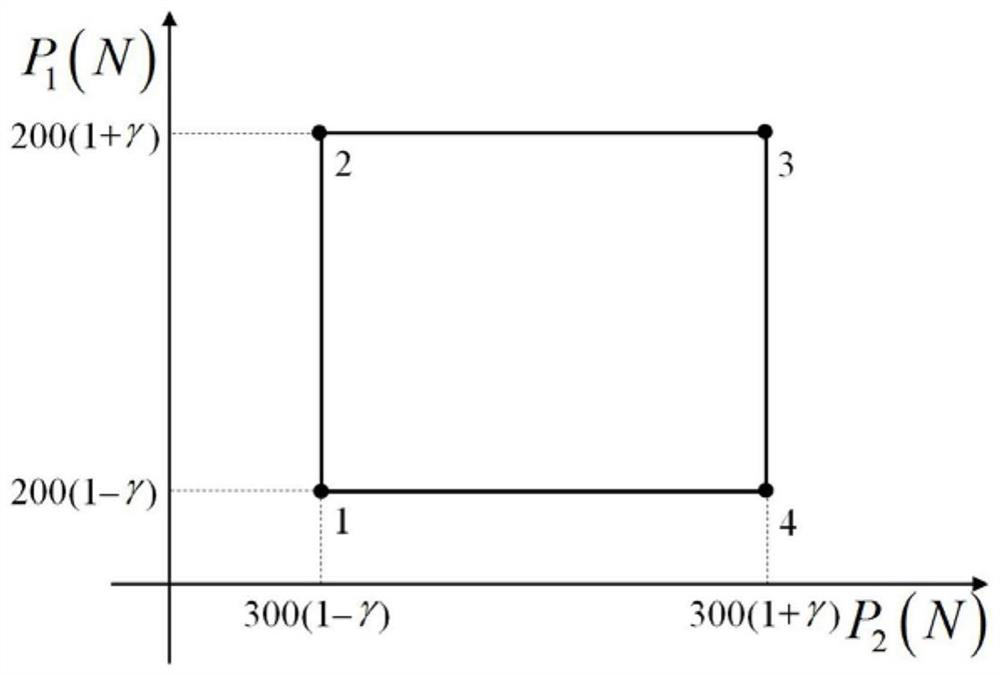
[0144] In order to understand more fully the characteristics of the invention and its applicability to engineering practice, the present invention aims at such as figure 2 The ten-bar truss shown is evaluated for the static displacement boundary of the structure containing convex polyhedral uncertain parameters. The length of each rod in the truss is L=1m, the elastic modulus of the material is E=200GPa, and the vertical load P acts on nodes 3 and 5 of the truss respectively 1 and P 2 , rods ①-⑥ have the same cross-sectional area and are equal to A 1, rods ⑦-⑩ have the same cross-sectional area and are equal to A 2 . Due to manufacturing and measurement errors, the external load vector P = (P 1 P 2 ) T and cross-sectional area vector A=(A 1 A 2 ) T are convex polyhedron uncertainty parameters, and satisfy the following constraints:
[0145] P∈P S (α), A ∈ A S (β)
[0146] Among them, P S and A S are respectively the vertex vector P of the convex polyhedron wi...
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com