Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

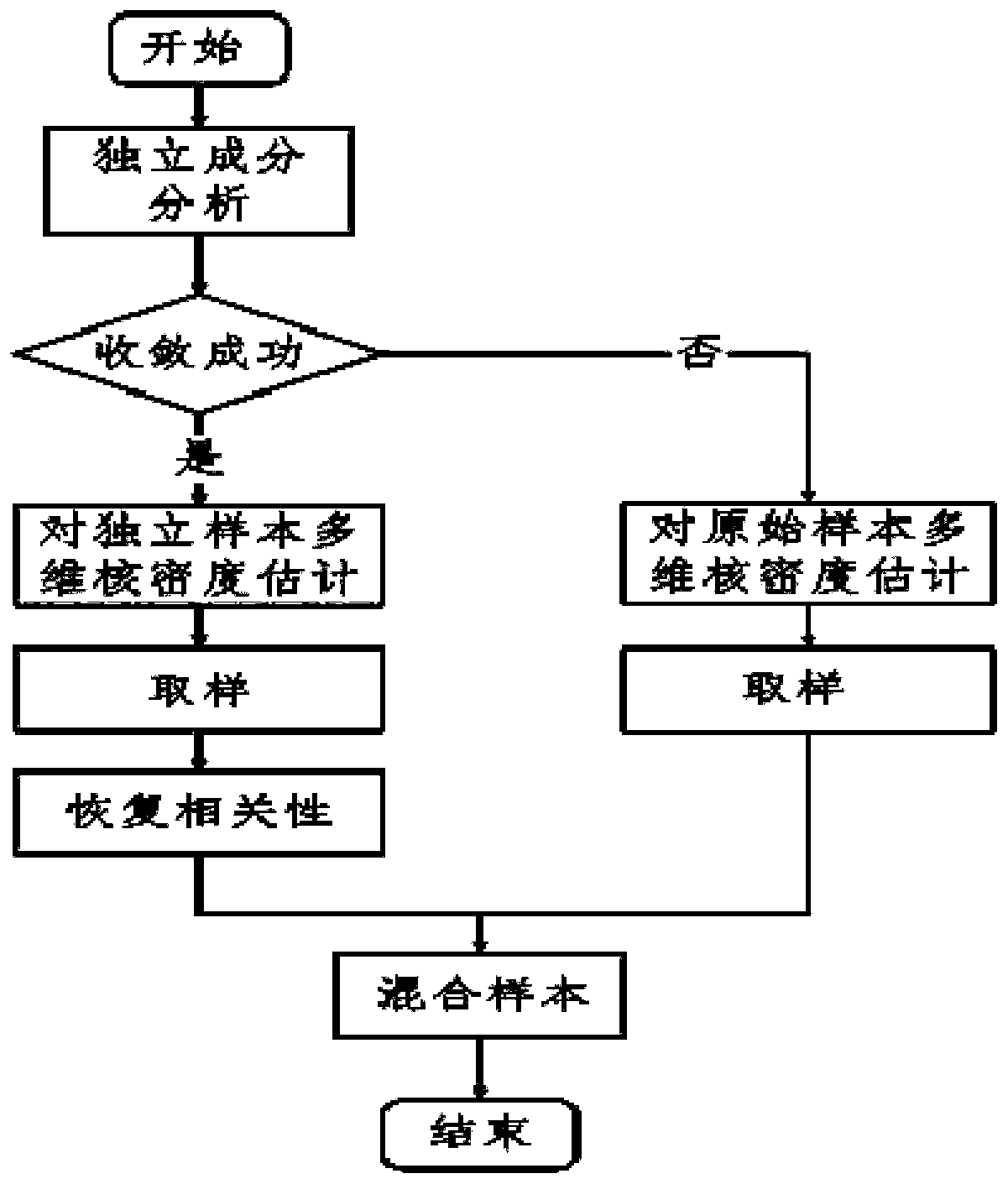
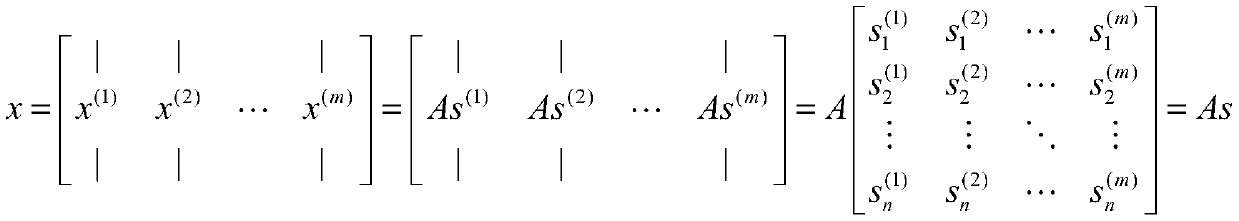Virtual sample generation method based on independent component analysis and kernel density estimation

A technology of independent component analysis and kernel density estimation, applied in the computer field, it can solve the problem of not considering the correlation between sample attributes, and achieve the effect of alleviating the shortage of training samples, improving the accuracy, and broadening the application area.

Inactive Publication Date: 2019-08-06
XI AN JIAOTONG UNIV
View PDF0 Cites 3 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0004] The existing virtual sample generation methods are mainly aimed at samples whose attributes are independent of each other, without considering the correlation between sample attributes

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Virtual sample generation method based on independent component analysis and kernel density estimation
  • Virtual sample generation method based on independent component analysis and kernel density estimation
  • Virtual sample generation method based on independent component analysis and kernel density estimation

Examples

Experimental program
Comparison scheme
Effect test

Embodiment

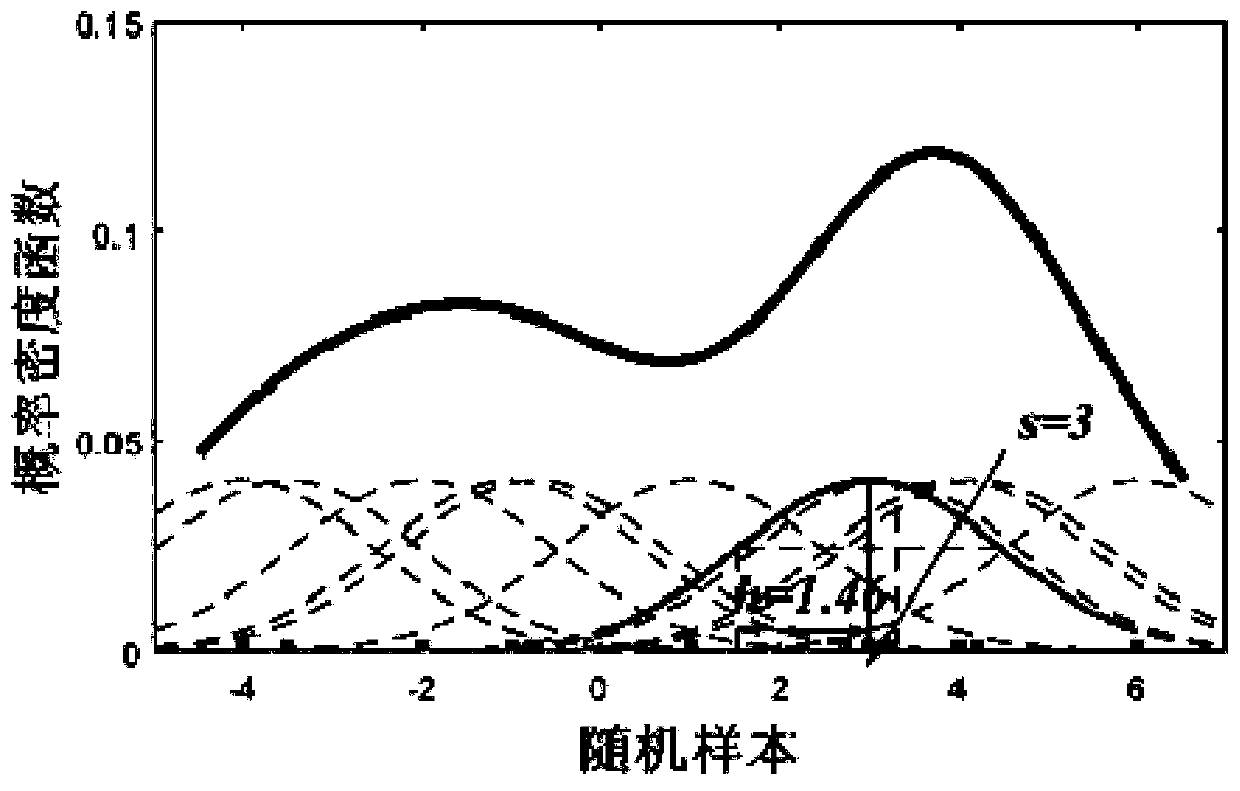
[0063] Let s=[-4,-3.5,-2,-1,-0.75,1,3,3.2,4,4.2,4,6], after kernel density estimation, figure 2 The solid line in the The dashed line depicts the Gaussian kernel function applied to each raw sample. When generating a dummy sample, first select an original sample, figure 2 is chosen as the original sample at s=3, and the blue curve depicts the Gaussian kernel function at s=3. Then generate a one-dimensional random number s that conforms to the normal distribution r , take s here r =0.29. Finally, the virtual independent sample s is obtained according to the h obtained by the kernel density estimation v. In this example s=3, s r =0.29, h=1.4614, according to the formula, get s v =3+0.29*1.461=3.4237.

[0064] Sampling according to the above steps until a satisfactory number of virtual independent samples s are obtained v , and finally according to formula (3), the independent virtual samples are mapped back to the original sample space to obtain virtual samples,

...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a virtual sample generation method based on independent component analysis and kernel density estimation. At a system operation initial stage, the number of training samples isinsufficient, a kernel density estimation method is used, the overall probability density function of a sample is estimated through the probability density function of a small number of samples, whenthe attributes of an original sample have the correlation, the correlation between the attributes of the original sample is removed through an independent component analysis method, then kernel density estimation is conducted, and a virtual sample is generated according to the probability density function obtained through estimation. The problem that training samples are insufficient when the machine learning model is trained can be relieved, and the accuracy of the machine learning model is improved. Compared with other virtual sample generation methods, an independent component analysis method is introduced to solve the problem that all attributes of the sample have correlation, so that the application range of the method is widened.

Description

technical field [0001] The invention belongs to the field of computers, in particular to a virtual sample generation method based on independent component analysis and kernel density estimation. Background technique [0002] At present, machine learning methods are more and more applied in various fields. For problems that cannot be solved by classical statistics, people hope that they can be solved by machine learning methods. The number of samples has a large impact on the accuracy of machine learning methods. However, in many cases, due to the limitation of sampling time and cost, there is often a problem of insufficient sample size. [0003] The virtual sample generation technology was first proposed by Niyogi et al. Wang Xu et al. divided the virtual sample generation methods into three categories, which are based on prior knowledge, based on disturbance and based on the distribution function of the research field. The virtual sample generation technology is applied...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06K9/62
CPCG06F18/2134G06F18/214
Inventor 董小社袁坤王龙翔张兴军王强王宇菲
Owner XI AN JIAOTONG UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com