Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Magnetic resonance image reconstruction method based on adaptive orthogonal basis

A technology of magnetic resonance imaging and orthonormal basis, which is used in image data processing, 2D image generation, instruments, etc.

Active Publication Date: 2015-09-09
SOUTHERN MEDICAL UNIVERSITY
View PDF4 Cites 15 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0006] The purpose of the present invention is to address the deficiencies of the existing MRI image reconstruction methods, and provide a MRI image reconstruction method based on an adaptive orthogonal basis that can simultaneously improve the quality of the reconstructed image and reduce the time-consuming calculation

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Magnetic resonance image reconstruction method based on adaptive orthogonal basis
  • Magnetic resonance image reconstruction method based on adaptive orthogonal basis
  • Magnetic resonance image reconstruction method based on adaptive orthogonal basis

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

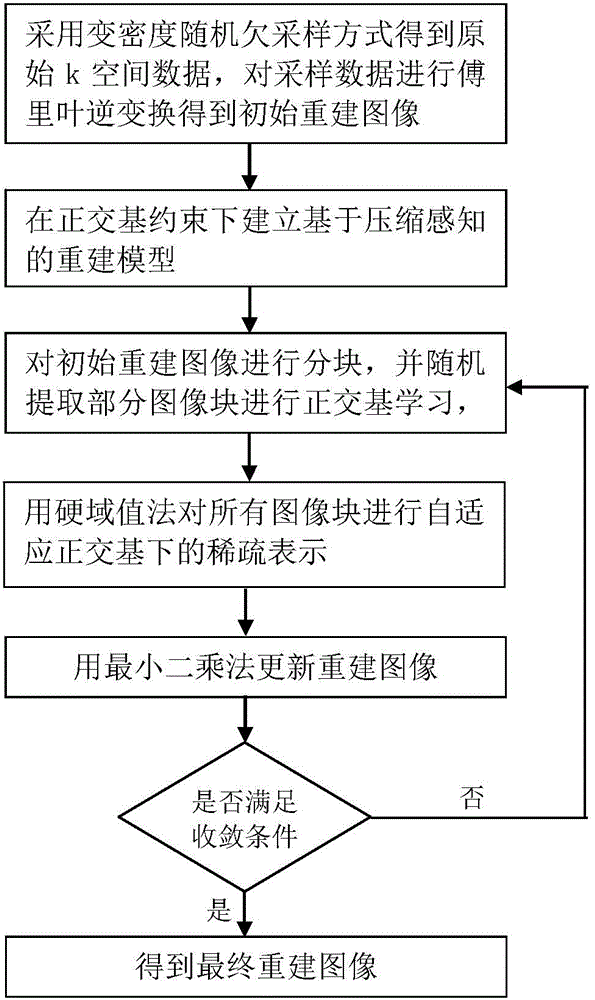
[0051] A MRI image reconstruction method based on an adaptive orthogonal basis, such as figure 1 shown, including the following steps:

[0052] (1) The original k-space data is obtained by random undersampling with variable density, and the inverse Fourier transform is performed on the sampled k-space data to obtain the initial reconstructed image;

[0053] (2) Establish a reconstruction model based on compressed sensing under the constraints of orthogonal bases;

[0054] (3) Blocking the initial reconstructed image, randomly extracting some image blocks to carry out orthogonal base learning, to obtain a group of adaptive orthogonal bases;

[0055] (4) carry out the sparse representation under the self-adaptive orthogonal base to all image blocks of step (3) subdividing with the hard domain value method;

[0056](5) update the reconstructed image with the least squares method to obtain the current reconstructed image;

[0057] (6) Judging whether the current reconstructed i...

Embodiment 2

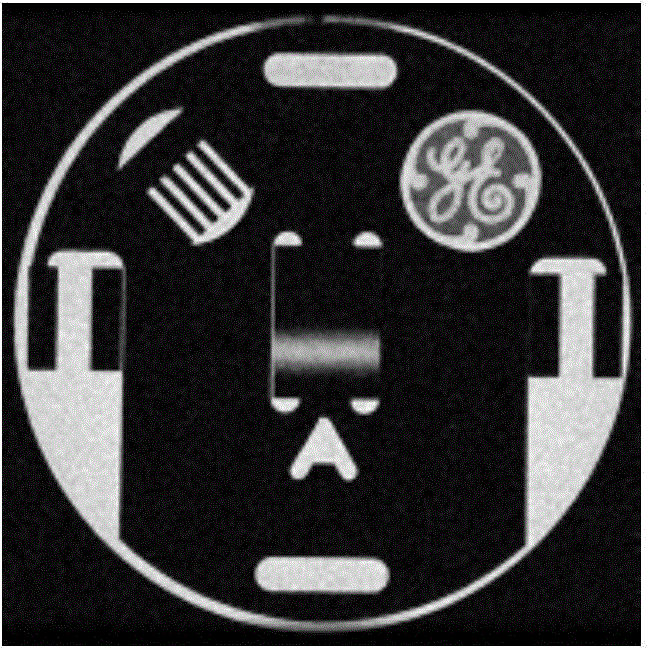
[0079] In order to verify the effect of the present invention, in this embodiment figure 2 The image reconstruction is performed based on the phantom data shown.

[0080] Under different subsampling factors for phantom data, the magnetic resonance image reconstruction method based on the adaptive orthogonal basis of the present invention includes the following steps:

[0081] (1) Obtain fully sampled original k-space data through magnetic resonance scanning, and perform retrospective undersampling on the original k-space data according to different given undersampling factors to obtain undersampled k-space data y;

[0082] Then perform zero-padded Fourier reconstruction on the k-space data y to obtain the initial value of the reconstructed image x, and let the initial value of Γ be a zero matrix.

[0083] (2) Establish a compressed sensing reconstruction model based on orthogonality constraints, as follows:

[0084] Apply orthogonality constraints to the base, and establish...

Embodiment 3

[0106] In order to verify the effect of the present invention, in this embodiment Figure 7 Image reconstruction is performed based on the brain simulation data shown.

[0107] (1) Perform Fourier transform on an ideal magnetic resonance image to obtain simulated full-sampled k-space data, and retrospectively under-sample the k-space data according to different given under-sampling factors to obtain under-sampling Sampling k-space data y;

[0108] The zero-filled Fourier reconstruction is performed on the k-space data y to obtain the initial value of the reconstructed image x, and the initial value of Γ is a zero matrix.

[0109] (2) Establish a compressed sensing reconstruction model based on orthogonality constraints, as follows:

[0110] Apply orthogonality constraints to the base, and establish a reconstruction model based on compressed sensing:

[0111]

[0112] Among them, y represents the undersampled k-space data, x represents the image to be reconstructed, and F...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a magnetic resonance image reconstruction method based on an adaptive orthogonal basis, and the method comprises the following steps: (1) obtaining original k-space data, carrying out the Fourier inversion of the sampled k-space data, and obtaining an original reconstruction image; (2) building a reconstruction model based on compression sensing under the constraint of the orthogonal basis; (3) partitioning the original reconstruction image, randomly extracting some image blocks for the learning of the orthogonal basis, and obtaining a group of adaptive orthogonal basis; (4) employing a hard threshold method to achieve the sparse representation of all image blocks based on the adaptive orthogonal basis; (5) employing a least square method to updating the reconstruction image, and obtaining a current reconstruction image; (6) judging whether the current reconstruction image meets the condition of convergence or not: if the current reconstruction image meets the condition of convergence, the current reconstruction image serves as a final reconstruction image; or else, going to step (7); (7) reducing the value of a regularization parameter, the current reconstruction image serves as the original reconstruction image, and returning to step (3). The method is high in reconstruction speed, and is good in image quality.

Description

technical field [0001] The invention relates to the technical field of magnetic resonance imaging, in particular to a magnetic resonance image reconstruction method based on an adaptive orthogonal basis under the compressed sensing theory. Background technique [0002] Compressed Sensing (CS) theory utilizes the sparsity of the signal under a set of bases or dictionaries, and only needs to collect some samples to reconstruct high-quality original signals under certain conditions. Compressed sensing theory is applied to fast magnetic resonance imaging, which can reconstruct the original image from partially sampled k-space, reduce the acquisition number of k-space, and achieve high-resolution magnetic resonance images under the condition of fast imaging speed this goal. [0003] In the application of compressed sensing, the method based on over-complete dictionary learning has great potential and is widely used in image denoising, image restoration, etc. In recent years, so...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06T11/00
Inventor 冯衍秋黄进红陈武凡
Owner SOUTHERN MEDICAL UNIVERSITY
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com