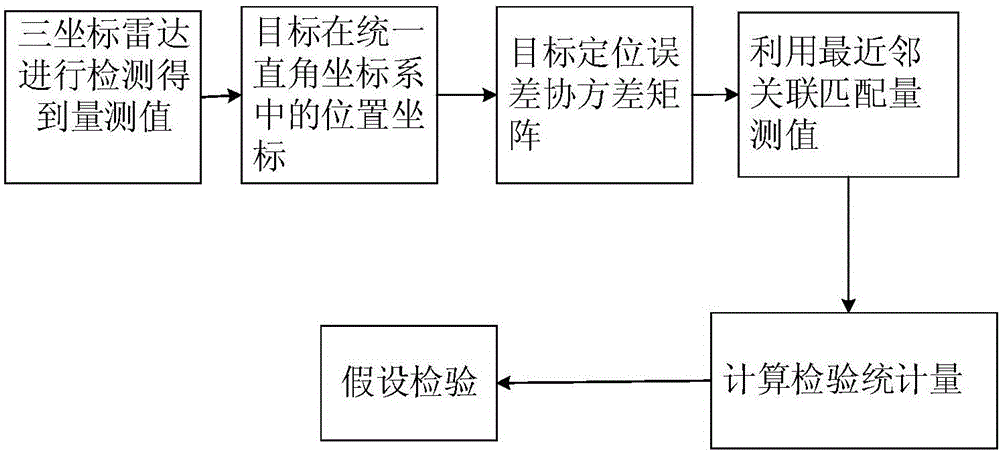
[0018] refer to figure 1 , the concrete realization steps of the present invention are as follows:
[0019] Step 1, the networked radar measures the target.
[0020] Each node radar in the networked radar system measures the target separately, and the measurement value of the i-th node radar for the k-th target is in, is the radial distance of the k-th target relative to the i-th node radar, is the azimuth of the k-th target relative to the i-th node radar, is the pitch angle of the k-th target relative to the i-th node radar;
[0021] Step 2, coordinate transformation
[0022] Perform coordinate transformation on the measured value of the target obtained in (1) to obtain the coordinates of the target in the unified rectangular coordinate system in:
[0023]
[0024] In the formula, are the radial distance, azimuth and pitch information of the target relative to the node radar, respectively, are the x-axis, y-axis, and z-axis coordinates of the i-th node radar in the unified coordinate system, respectively.
[0025] Step 3: Derive the positioning error covariance matrix.
[0026] 3a) For the position coordinate M of the target in the unified rectangular coordinate system i (k) Take the derivation to obtain the relationship expression between the target positioning position error, the radar measurement error, and the site error:
[0027]
[0028] in, are the radial distance, azimuth angle and pitch angle information of the target relative to the node radar, respectively;
[0029] 3b) The target positioning error Denoted as dX, the radar measurement error Denote it as dV, and denote the radar site error as dX s , the relationship between the target positioning position error, radar measurement error, and site error in 3a) is simplified as:
[0030] dX=CdV+dX s
[0031] in,
[0032]
[0033] From the above relationship, when the target position information is transformed into a unified rectangular coordinate system, the target positioning error dX, the radar measurement error dV and the radar site error dX s In a linear relationship, the Gaussian distribution is still valid, so the target positioning error dX is still a Gaussian distribution random variable with zero mean;
[0034] 3c) Calculate the target positioning error covariance matrix P:
[0035] P=E[dXdX T ]
[0036] =CE[dVdV T ]C T +E[dX s dX s T ]
[0037] in,
[0038]
[0039]
[0040] σ r , σ θ , are the ranging error, azimuth angle error and pitch angle error of the radar, respectively; σ xs , σ ys , σ zs are the site errors of the radar on the x-axis, y-axis and z-axis, respectively.
[0041] Step 4: Obtain an association measurement sequence through nearest neighbor association.
[0042] The data in the measurement set of each node radar is matched by the method of nearest neighbor correlation, and the measurement value with the smallest Euclidean distance is matched to the same correlation measurement sequence. One correlation measurement sequence corresponds to the amount of a target in different node radars. Measured value, its realization steps are as follows:
[0043] 4a) Find the node radar s with the least measured value as the reference radar;
[0044] 4b) Select a measurement value s of the reference radar s i , calculate its Euclidean distance from all measurements of the l-th radar:
[0045] in, are the x-axis, y-axis, and z-axis coordinates of the i-th measurement value of the reference radar s, is the x-axis, y-axis, and z-axis coordinates of the jth measurement value of node radar 1;
[0046] 4c) Select the measurement value of the lth radar corresponding to the minimum value of the Euclidean distance obtained in 4b) to match the measurement value of the reference radar s to the same associated measurement sequence.
[0047] Step 5, perform hypothesis testing.
[0048] 5a) Propose two mutually opposing first hypotheses H 0 and the second hypothesis H 1 :
[0049] The first hypothesis H 0 for the two measurements Z i and Z j Generated in different node radars for real targets;
[0050] Second Hypothesis H 1 for the two measurements Z i and Zj At least one of them is generated by deceptive false targets;
[0051] 5b) Calculate the difference between any two radar measurement errors ΔZ=dZ i -dZ j ~N(0,∑ ij ),in,
[0052] ∑ ij =E[(dZ i- dZ j )(dZ i- dZ j ) T ]=P i +P j
[0053] dZ i is the measurement error of the i-th measurement value, dZ j is the measurement error of the jth measurement value, then in H 0 Under the condition that is established, ΔZ also approximately obeys the zero-mean Gaussian distribution;
[0054] 5c) Calculate the Mahalanobis distance between the radar measurements of two nodes in an association sequence:
[0055]
[0056] Among them, Z i is the i-th measurement value, Z j For the jth measurement value, the Mahalanobis distance d ij As a statistical test quantity, in H 0 Under the condition that is established, the Mahalanobis distance d ij Obey the distribution of the other party, so according to the δ of the decision threshold given by the radar user, the Mahalanobis distance d ij Do the following hypothesis tests:
[0057] if d ij ≤δ is established, then accept the first hypothesis H 0 , that is, to determine the two measurement values Z i and Z j is the measurement value of the real target in different node radars;
[0058] if d ijδ is established, then accept the second hypothesis H 1 , that is, to determine the two measurement values Z i and Z j For the measurement values of false targets in different node radars, the identification of true and false targets is completed.
[0059] The effectiveness of the present invention against deceptive false targets can be verified by the following simulation experiments.
[0060] 1. Experimental scenario: three radars are used as the networking model of the node radar, and a real target is set, whose position is 50km in the x-dimension direction, 50km in the y-dimension direction, and 50km in the z-dimension direction. The coordinates are 1km, and a deceptive false target is set for each radar station. The radar information of each node is shown in Table 1. Among them, the positioning error of the node radar to itself is the same in three dimensions, namely In the hypothesis testing model, the significance level α=0.01, and the detection threshold δ=9.21.
[0061] Table 1 Radar parameter information table of each node
[0062]
[0063] 2. Experiment content and result analysis
[0064] Experiment 1: Change the deception distance of the false target, and the variation range is 0m to 1000m. Use the present invention and the existing networked radar anti-deceptive false target jamming algorithm to distinguish the true and false targets respectively, and obtain the correct identification probability and networking of the true target. The probability of radar being deceived, the results are as follows figure 2 shown. in figure 2 (a) is the variation curve of the discrimination probability of the true target, figure 2 (b) is the change curve of the probability of being deceived by the networked radar.
[0065] Depend on figure 2 (a) it can be seen: the correct identification probability of the present invention to the true target is higher than the correct identification probability of the existing algorithm to the true target, and along with the increase of the false target deception distance, the correct identification probability of the two algorithms to the true target is basically unchanged; by figure 2 (b) It can be seen that with the increase of the false target deception distance, the deception probability of the networked radar obtained by the two algorithms gradually decreases, and the deception probability of the networked radar obtained by the original algorithm is slightly lower than that in the present invention. The probability of being deceived by the network radar obtained by the algorithm.
[0066] Experiment 2: Change the deception distance of false targets, ranging from 0m to 1000m, use the invention to identify true and false targets, set three groups of different radar site errors, 0m, 30m, 60m, respectively, and count the real target identification Changes in the probability and the probability of being deceived by the networked radar, the results are as follows image 3 shown, where image 3 (a) is the variation curve of the discrimination probability of the true target, image 3 (b) is the change curve of the probability of being deceived by the networked radar.
[0067] from image 3 (a) It can be seen that when using the present invention to identify true and false targets, the size of the radar site error affects the real target identification probability and the probability of being deceived by the networked radar. With the increase of the site error, the real target identification probability gradually decreases. The probability of being deceived by the networked radar is gradually reduced. Therefore, after considering the site error factor, the detection probability and the deception probability obtained by the original algorithm can be corrected, bringing more accurate data to real applications and avoiding unnecessary resource consumption.
