Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

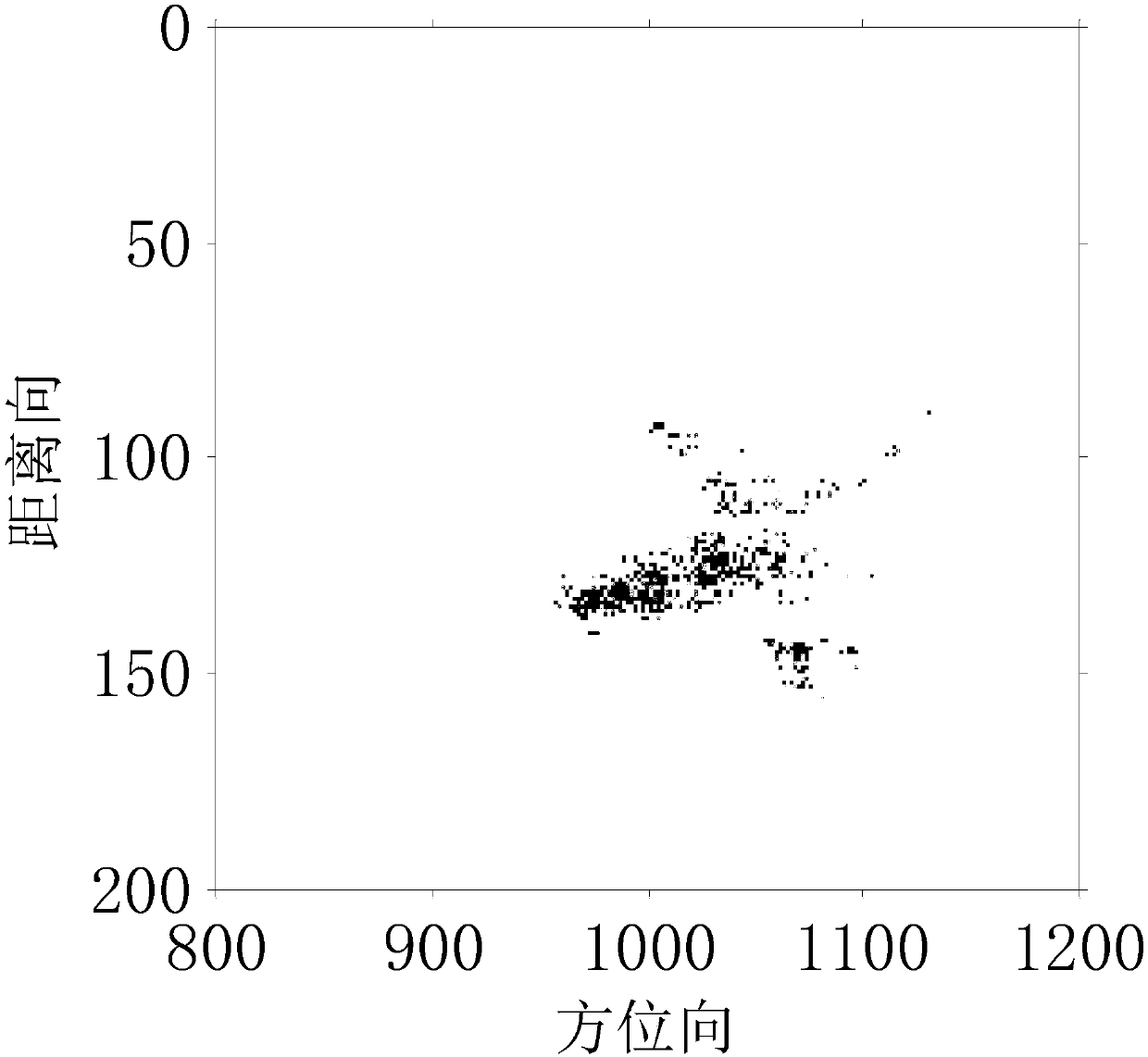
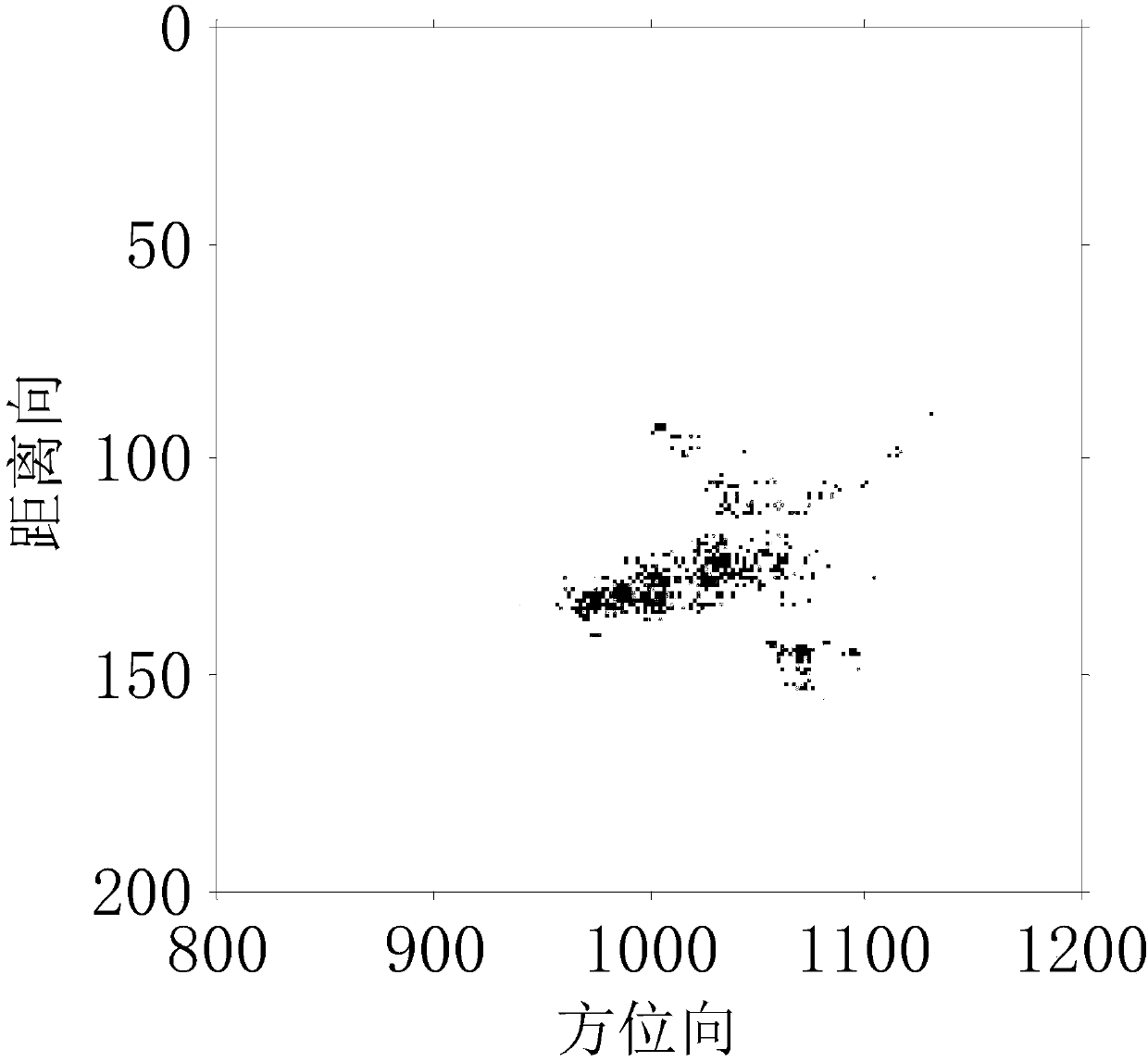
Characteristic vector phase compensation method based on Arnoldi

A phase compensation and eigenvector technology, applied in the field of inverse synthetic aperture radar, can solve the problem of large amount of calculation, achieve the effect of reducing the amount of calculation, ensuring the estimation performance, and reducing the amount of calculation

Active Publication Date: 2018-08-24
HARBIN INST OF TECH
View PDF7 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0003] The purpose of the present invention is to solve the problem that the existing eigenvector-based phase compensation method has a large amount of calculation, thus proposing an Arnoldi-based eigenvector phase compensation method

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Characteristic vector phase compensation method based on Arnoldi
  • Characteristic vector phase compensation method based on Arnoldi
  • Characteristic vector phase compensation method based on Arnoldi

Examples

Experimental program
Comparison scheme
Effect test

specific Embodiment approach 1

[0016] Specific implementation mode one: combine figure 1 To illustrate this embodiment, the specific process of an Arnoldi-based eigenvector phase compensation method in this embodiment is as follows:

[0017] Step 1: Take M target echoes, the complex range image of the mth target echo distributed along the distance n after range dimension compression is s(m,n), and take a modulo of the complex range image to obtain a target echo dimensional distance image, and use the cumulative cross-correlation method for envelope alignment to obtain the distance dimension compressed data after envelope alignment;

[0018] Among them, 0≤m≤(M-1), 0≤n≤(N-1), M is the number of points in the azimuth direction (that is, the number of target echoes), and N is the number of points in the distance direction;

[0019] Step 2, obtain the sampling covariance matrix according to the distance dimension compressed data after envelope alignment;

[0020] Step 3. Use Arnoldi to iteratively solve the e...

specific Embodiment approach 2

[0024] Specific embodiment 2: The difference between this embodiment and specific embodiment 1 is that in the first step, M target echoes are taken, and the complex range image of the mth target echo distributed along the distance n after distance dimension compression is s(m,n), take the modulus of the complex range image to obtain the one-dimensional range image of the target echo, and use the cumulative cross-correlation method to perform envelope alignment to obtain the range dimension compressed data after envelope alignment; the specific process is:

[0025] Step 11, the complex range image of the m-th target echo distributed along the distance n after the distance dimension compression is expressed as s(m,n);

[0026] Among them, 0≤m≤(M-1), 0≤n≤(N-1), M is the number of points in the azimuth direction (that is, the number of target echoes), N is the number of points in the distance direction, and the values ​​of N and M are positive integers ;

[0027] Steps 1 and 2, t...

specific Embodiment approach 3

[0036] Specific embodiment three: the difference between this embodiment and specific embodiment one or two is: in the step two, the sampling covariance matrix is ​​obtained according to the distance dimension compressed data after the envelope alignment; the specific process is:

[0037] Step 21, the complex envelope s of the distance dimension compressed data of the dth distance unit d Expressed as:

[0038]

[0039] Among them, a d is the scattering rate of the strong scattering point in the dth distance unit, d=1,2,...,N-1, v is the phase error vector, n d is Gaussian white noise, is the phase difference between the e+1th azimuth unit and the first azimuth unit, e=1,2,...,M-1, is an imaginary unit;

[0040] s(m,n) is the complex range image expression of the m-th echo distributed along the distance n after distance dimension compression, 0≤m≤(M-1), 0≤n≤(N-1), so after The echo data compressed in the range dimension is an M×N matrix, each row of the matrix is ​​a ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

PropertyMeasurementUnit
Bandwidthaaaaaaaaaa
Pulse repetition frequencyaaaaaaaaaa
Login to View More

Abstract

The invention relates to a characteristic vector phase compensation method based on Arnoldi. By using an existing phase compensation method based on a characteristic vector, a calculated amount is large. By using the method of the invention, the above problem is solved. The method is characterized by 1, taking M target echoes, acquiring the one-dimensional distance image of the target echoes by modeling a complex distance image, using an accumulation cross correlation method to carry out envelope alignment and acquiring distance dimension compression data after envelope alignment; 2, calculating a sampling covariance matrix; 3, using Arnoldi iteration to solve a characteristic vector corresponding to the maximum characteristic value of the sampling covariance matrix, extracting phase information of each element in the characteristic vector corresponding to the maximum characteristic value, carrying out phase compensation on the distance dimension compression data after the envelope alignment in the step1, and acquiring the data after phase compensation; and 4, using a range Doppler method to carry out ISAR imaging on the data after the phase compensation. The method is used for theinverse synthetic aperture radar field.

Description

technical field [0001] The invention belongs to the field of inverse synthetic aperture radar (ISAR), and relates to an Arnoldi-based eigenvector phase compensation method. Background technique [0002] Inverse Synthetic Aperture Radar (ISAR) uses the relative motion of the radar and the target to achieve high-resolution imaging of space targets, and has been widely used in military and civilian fields. Motion compensation is an important step in ISAR imaging, which is divided into two parts: envelope alignment and phase compensation. Envelope alignment is the basis for phase compensation. At present, the method of envelope alignment using the similarity of adjacent one-dimensional images is the most commonly used method, so the method of accumulating cross-correlation is used to perform envelope alignment of one-dimensional range images. As a global phase compensation method, the eigenvector-based phase compensation method can be applied to both full aperture and sparse a...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G01S7/41
CPCG01S7/41
Inventor 刘秋晨王勇
Owner HARBIN INST OF TECH
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com