Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

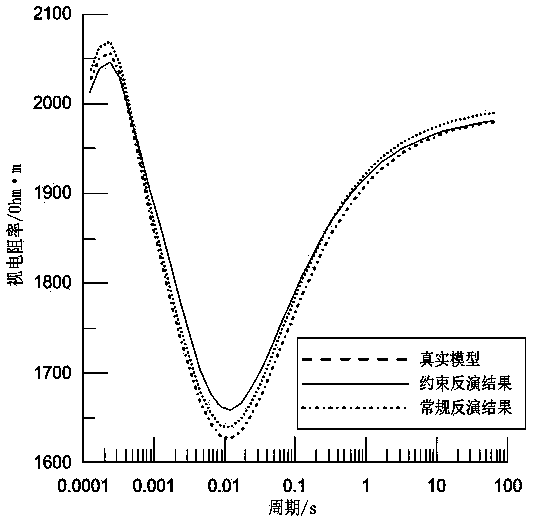
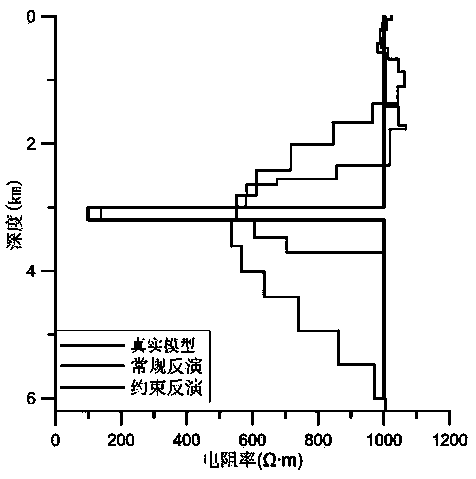
Electromagnetic sounding constraint inversion methods based on resistivity equivalence principle

An electromagnetic sounding and constrained inversion technology, applied in the field of geophysical exploration, can solve problems such as poor adaptability, difficulty in obtaining inversion results, and slow calculation speed, so as to simplify the working process, highlight formation information, and reduce work intensity Effect

Active Publication Date: 2019-07-26
CHENGDU UNIVERSITY OF TECHNOLOGY
View PDF2 Cites 6 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0007] The existing technology represented by the above two papers obtains the depth and burial depth of the high-resistivity thin layer by restricting the initial model when it is known that there is a high-resistance thin layer underground; The response of the layer on the apparent resistivity curve to determine its resolution; it is difficult to obtain more realistic inversion results, and there are problems of slow calculation speed and poor adaptability

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Electromagnetic sounding constraint inversion methods based on resistivity equivalence principle
  • Electromagnetic sounding constraint inversion methods based on resistivity equivalence principle
  • Electromagnetic sounding constraint inversion methods based on resistivity equivalence principle

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0050] An electromagnetic sounding constrained inversion method based on the principle of resistivity equivalence, comprising the following steps:

[0051] a. Assuming that the result of an inversion is m, calculate the derivative of the layer thickness for the forward modeling response, normalize the negative derivative value, and take the positive derivative value as 0 to obtain Δm;

[0052] b. Use Δm as the coefficient corresponding to ε in the S equivalence convergence algorithm of each layer;

[0053] c. Use S equivalence to converge low-resistance thin layers, and iteratively obtain Equation 2 through Equation 1; solve the m-th layer z through the inversion data m 、h m and ρ m , gradually decrease h at the speed of Δ m The value of h is reduced every time m The value of ρ will iteratively calculate the unique corresponding ρ m , until h m and ρ m The value of satisfies formula 1, the convergence ends, and the sheet resistivity and sheet thickness after convergence...

Embodiment 2

[0061] An electromagnetic sounding constrained inversion method based on the principle of resistivity equivalence, comprising the following steps:

[0062] a. Assuming that the result of an inversion is m, calculate the derivative of the layer thickness for the forward modeling response, normalize the negative derivative value, and take the positive derivative value as 0 to obtain Δm;

[0063] b. Use Δm as the coefficient corresponding to ε in the S equivalence convergence algorithm of each layer;

[0064] c. Use S equivalence to converge low-resistance thin layers, and iteratively obtain Equation 2 through Equation 1; solve the m-th layer z through the inversion data m 、h m and ρ m , gradually decrease h at the speed of Δ m The value of h is reduced every time m The value of ρ will iteratively calculate the unique corresponding ρ m , until h m and ρ m The value of satisfies formula 1, the convergence ends, and the sheet resistivity and sheet thickness after convergence...

Embodiment 3

[0073] An electromagnetic sounding constrained inversion method based on the principle of resistivity equivalence, comprising the following steps:

[0074] a. Assuming that the result of an inversion is m, calculate the derivative of the layer thickness for the forward modeling response, normalize the negative derivative value, and take the positive derivative value as 0 to obtain Δm;

[0075] b. Use Δm as the coefficient corresponding to ε in the S equivalence convergence algorithm of each layer;

[0076] c. Use S equivalence to converge low-resistance thin layers, and iteratively obtain Equation 2 through Equation 1; solve the m-th layer z through the inversion data m 、h m and ρ m , gradually decrease h at the speed of Δ m The value of h is reduced every time m The value of ρ will iteratively calculate the unique corresponding ρ m , until h m and ρ m The value of satisfies formula 1, the convergence ends, and the sheet resistivity and sheet thickness after convergence...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

PropertyMeasurementUnit
Resistivityaaaaaaaaaa
Login to View More

Abstract

The invention discloses electromagnetic sounding constraint inversion methods based on a resistivity equivalence principle and belongs to the field of geophysical exploration. A method is characterized by comprising the following steps of a, assuming that a one-time inversion result is m, and carrying out a forward action on the m to obtain the derivative of response with respect to layer thickness, thereby obtaining deltam; b, taking the deltam as a coefficient corresponding to epsilon in an S equivalence convergence algorithm of each layer; c, carrying out low resistivity thin layer convergence through utilization of S equivalence, thereby obtaining converged thin layer resistivity and thin layer thickness; and d, carrying out high resistivity thin layer thickness compensation through utilization of H equivalence, adding 1 / 2 of low resistivity thin layer reduced thickness to an upper high resistivity thin layer, and solving resistivity of the high resistivity thin layer. According tothe method, under the condition that no any known condition exists, relatively accurate high resistivity thin layer and low resistivity thin layer information can be obtained at the same time, so inversion explanation precision is improved; computing speed is fast; and adaptability is high.

Description

technical field [0001] The invention relates to the technical field of geophysical exploration, in particular to an electromagnetic sounding constrained inversion method based on the principle of resistivity equivalence. Background technique [0002] Magnetotelluric sounding MT is a geophysical exploration method that uses natural alternating electromagnetic fields to study the electrical structure of the earth. Based on the principle that the penetration depth of high-frequency electromagnetic waves is small to the ground and the penetration depth of low-frequency electromagnetic waves is large, through the main observation of the magnetotelluric pulse signal with a frequency range of 10-4102Hz at a point on the ground, and after data processing and analysis, it is obtained to reflect the point The apparent resistivity curves of the magnetotelluric bathymetric sounding at different depths are used to make geological interpretations. [0003] The multi-solution nature of in...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G01V3/38
CPCG01V3/38
Inventor 王绪本唐荣江郭家松乃国茹
Owner CHENGDU UNIVERSITY OF TECHNOLOGY
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com