Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

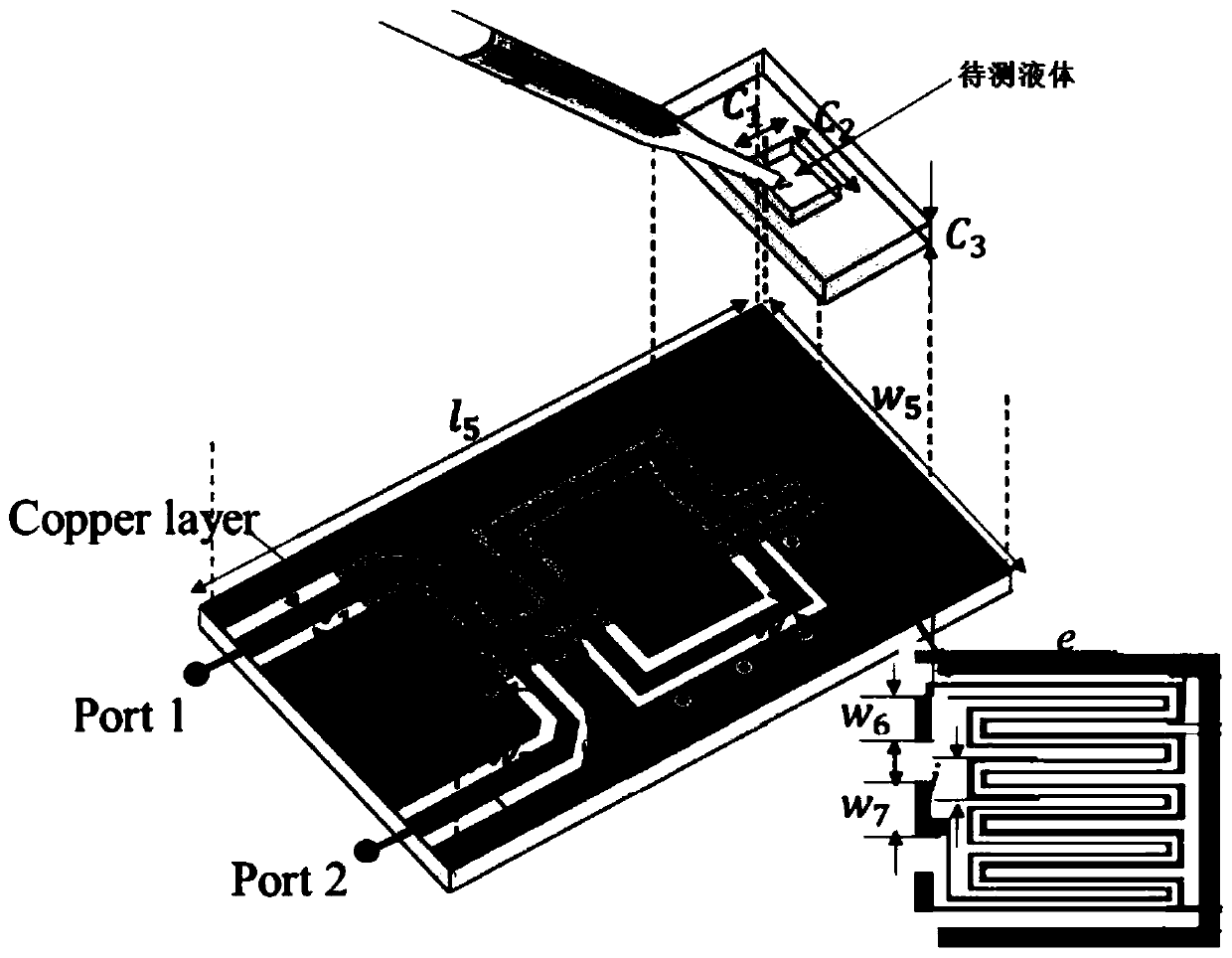
Planar microwave cavity drip-in type liquid dielectric substance value detection sensor

A microwave cavity and dielectric technology, which is applied in the direction of material analysis, instruments, and measurement devices using microwave means to achieve the effect of simple processing circuits

Active Publication Date: 2019-11-26
DALIAN UNIV OF TECH
View PDF5 Cites 4 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

However, this requires a certain volume of liquid to analyze the dielectric properties
Especially in the detection of biological and chemical solvents, the limited volume of liquid samples is a practical problem that limits the analysis of liquid materials

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Planar microwave cavity drip-in type liquid dielectric substance value detection sensor
  • Planar microwave cavity drip-in type liquid dielectric substance value detection sensor
  • Planar microwave cavity drip-in type liquid dielectric substance value detection sensor

Examples

Experimental program
Comparison scheme
Effect test

Embodiment Construction

[0028] The inner dimension of the FR4 container that the present invention adopts is 7.5 * 7.5mm 2 , to cover the sensor detection area. The height of the container is 1.6 mm, which provides enough space for adjusting the volume of the liquid to be measured. The width and length of the container (ie c1 and c2) are respectively larger than the width of the detection area sensor (4.1×4.6mm 2 ) 3.4 and 2.9mm larger to ensure that the liquid to be tested is exposed to the electric field on the side of the container.

[0029] A parametric study of the influence of the volume of the liquid to be measured on the reflected notch frequency and decibels is carried out. different volumes of liquid to be tested for the evaluation of the sensor, Figure 5 Displays the measurement results. S11 was evaluated for different volumes of 0.02, 0.04, 0.06, 0.08 and 0.1 mL of deionized (DI) water placed on the detection area. Deionized water was chosen because it has a high dielectric constant...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention belongs to the technical field of microwave sensing and relates to a planar microwave cavity drip-in type liquid dielectric substance value detection sensor. A square OCSRR of the detection sensor is fixed on the top surface of a single-sided FR4 substrate; a rectangular detection area exists at the top of the square OCSRR; the rectangular detection area is of a rectangular structure; two output ends of the square OCSRR are connected with two input ends in the rectangular detection area; a three-sided metal rectangular frame is arranged outside the rectangular structure; two endsof the metal rectangular frame are suspended; two metal wires in the rectangular detection area are located in the metal frame; one end of each of the two metal wires is suspended; the metal wires are arranged in the metal frame so as to form a rectangular double-loop form; the two metal wires are parallel to each other; and the direction of the inner metal wire is opposite to that of the external metal wire. According to the invention, a drip-in mode is adopted; a to-be-detected liquid is directly dripped to a sensing area; a high-precision micro-channel does not need to be additionally manufactured; the sensing end is suitable for mass production; and a back-end processing circuit is simple.

Description

technical field [0001] The invention belongs to the technical field of microwave sensing, and relates to a drop-in liquid dielectric value detection sensor of a planar microwave cavity. Background technique [0002] Microwave sensing is a reliable method for liquid characterization that has been applied over the past decade. Split-ring resonators (SRRs), complementary SRRs (CSRRs), open CSRRs (OCSRRs) and other cavity resonator particles, isolated or coupled to transmission lines, have become the focus of considerable attention for sensing applications. The electromagnetic properties of these particles depend on the resonant frequency shift and quality factor change of the liquid variables. By measuring the reflection coefficient and transmission coefficient, the complex dielectric parameters of the liquid sample can be obtained. This provides a new sensing platform for the bio, pharmaceutical and fuel industries. Most microwave sensors are placed in supercylindrical duct...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G01N22/00
CPCG01N22/00
Inventor 李杰森王志强李国锋
Owner DALIAN UNIV OF TECH
Features
  • Generate Ideas
  • Intellectual Property
  • Life Sciences
  • Materials
  • Tech Scout
Why Patsnap Eureka
  • Unparalleled Data Quality
  • Higher Quality Content
  • 60% Fewer Hallucinations
Social media
Patsnap Eureka Blog
Learn More

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com