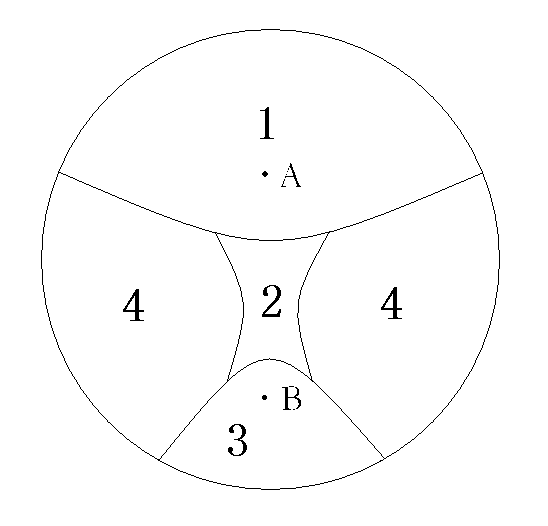Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Design method of progressive addition ophthalmic lens

A technology for progressive multi-focal ophthalmic lenses, which is applied in glasses/goggles, optics, instruments, etc., and can solve the problem of insufficient flexibility of progressive multi-focal ophthalmic lenses

Active Publication Date: 2013-08-14
SUZHOU UNIV OF SCI & TECH +2
View PDF5 Cites 12 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0005] The contour line clusters involved in the above-mentioned prior art only have several fixed forms, which is not flexible enough when designing progressive multifocal ophthalmic lenses for different purposes

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Design method of progressive addition ophthalmic lens
  • Design method of progressive addition ophthalmic lens
  • Design method of progressive addition ophthalmic lens

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0073] In this embodiment, the lens radius of the lens to be processed is R=36mm, and the refractive power of the far zone is 4 diopters, near vision It is 6 diopters, and the focal power of the lens is 2 diopters. Set the lens gradient channel length h=36mm, and the distance L from A to the lens center point O=18mm. The lens material has a refractive index of 1.523.

[0074] The lens design steps are as follows:

[0075] 1. The value of u at point A is , at point B is , the change form of u on the meridian is a straight line. The boundary around the square u is in the form of a straight line boundary, where the sides are straight lines with a certain slope: .

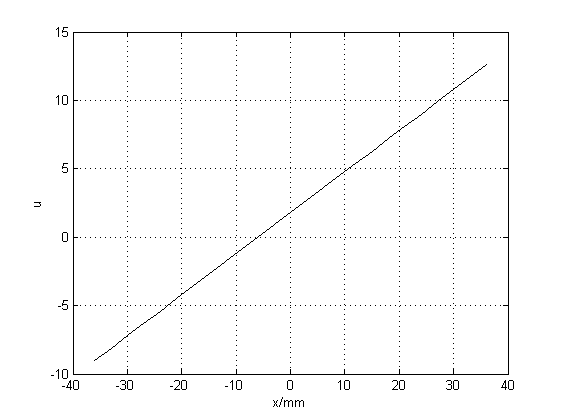
[0076] Such as figure 2 As shown in the figure, the abscissa is the longitudinal coordinate of the lens, and the value of the ordinate is u. The top and bottom connect the two sides with a straight line, and the contour cluster is obtained by numerically solving the Laplace equation Contours such as ...

Embodiment 2

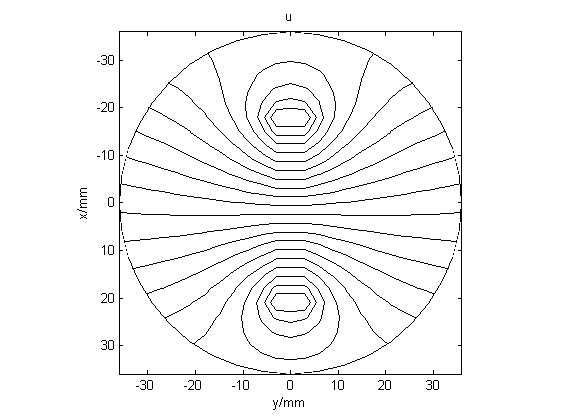
[0091] In this embodiment, lens parameters and design steps are the same as those in Embodiment 1. The difference is that in step 2, the boundary conditions around the u square are in the form of curved boundaries, and the four sides are all polynomials. Figure 8 are the boundary curves for the top, bottom and side edges, Figure 9 Contour plot of u obtained for solving Laplace's equation. Figure 10 is the three-dimensional grid map of the focal power distribution of the lens, Figure 11 , Figure 12 Respectively, the focal power distribution and astigmatism distribution diagram of the lens.

[0092] From Figure 9 It can be seen from the u contour line in the figure that the u contour line in the far-sighted area is flatter and wider than the u contour line in the near-sighted area. From this embodiment, the lens focal power three-dimensional grid map ( Figure 10 ) and focal power contour map ( Figure 11 ), it can be seen that the focal power of the far viewing ar...

Embodiment 3

[0094] In this embodiment, lens parameters and design steps are the same as those in Embodiment 1. The difference is that the change form of u between the reference point A of the far-sighted area and the reference point B of the near-sighted area on the meridian is a Gaussian curve, such as Figure 13 shown. The boundary conditions around the u square are in the form of curved boundaries, and the four sides are polynomials.

[0095] Figure 14 are the boundary curves for the top, bottom and side edges, Figure 15 Contour plot of u obtained for solving Laplace's equation. Figure 16 is the three-dimensional grid map of the focal power distribution of the lens, Figure 17 , Figure 18 Respectively, the focal power distribution and astigmatism distribution diagram of the lens.

[0096] From Figure 13 The change form of u between the reference point A of the far-sighted area and the reference point B of the near-sighted area on the neutron meridian is a Gaussian curve. T...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

PropertyMeasurementUnit
Lengthaaaaaaaaaa
Login to View More

Abstract

The invention relates to a design method of a progressive addition ophthalmic lens. According to the invention, a contour line is designed by using a numerical method to dissolve Laplace's equation, so as to design a method of the progressive addition ophthalmic lens. The boundary of the Laplace's equation is set to a square boundary; the boundaries of four edges of the square are linear form or a curve form; and the curve form may be a polynomial function, a trigonometric polynomial function, an exponential polynomial function, etc. A contour line cluster is obtained by dissolving the Laplace's equation with the numerical method; and the curvature radius distribution of the progressive addition ophthalmic lens can be obtained in combination with the focal power distribution on the meridian line so as to determine the surface shape of the progressive addition ophthalmic lens. The design method provided by the invention can be used for conveniently and flexibly designing multi-purpose progressive addition ophthalmic lenses to meet the requirement of different wearers.

Description

technical field [0001] The invention relates to a design method of an ophthalmic lens, in particular to a method for designing a progressive multifocal ophthalmic lens by using a numerical method to solve the Laplace equation to design a contour line cluster. Background technique [0002] Progressive multifocal ophthalmic lenses can meet the needs of distance vision and near vision at the same time, and avoid the defects of bifocal lenses such as vision breakage when switching from distance vision to near vision. Progressive multifocal ophthalmic lenses are attracting more and more people's attention, and their application range is becoming wider and wider, and their application prospects are very broad. See attached figure 1 , The surface of progressive multifocal ophthalmic lenses is divided into 1 far zone, 2 gradient channels (or intermediate transition zone), 3 near zone and 4 astigmatism zone. figure 1 A is the reference point for the far vision area, and B is the re...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G02C7/06G02C7/02
Inventor 吴泉英唐运海陈晓翌余浩墨陈芒保吴晓旭
Owner SUZHOU UNIV OF SCI & TECH
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com