Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Fast simulation method for non-stationary random process

A stochastic process and simulation method technology, applied in special data processing applications, instruments, electrical digital data processing, etc., can solve the problems that two-dimensional FFT cannot be used, and non-stationary stochastic process simulation cannot be directly applied

Inactive Publication Date: 2017-05-17
SOUTHWEST JIAOTONG UNIV
View PDF0 Cites 11 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

However, due to the need to transform multi-point non-stationary random processes into non-stationary random waves, this simulation method cannot be directly applied to the simulation of non-stationary random processes
Also, since the 2D EPSD varies with time and distance, the above simulation method cannot use 2D FFT

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Fast simulation method for non-stationary random process
  • Fast simulation method for non-stationary random process
  • Fast simulation method for non-stationary random process

Examples

Experimental program
Comparison scheme
Effect test

example 1

[0167] Example 1: Simulation of non-stationary and heterogeneous ground motion

[0168] Assuming that the distance between two earthquake simulation points is 10m, and a total of 401 points are simulated. The evolution power spectrum of an earthquake adopts an inseparable form, and its formula is as follows:

[0169]

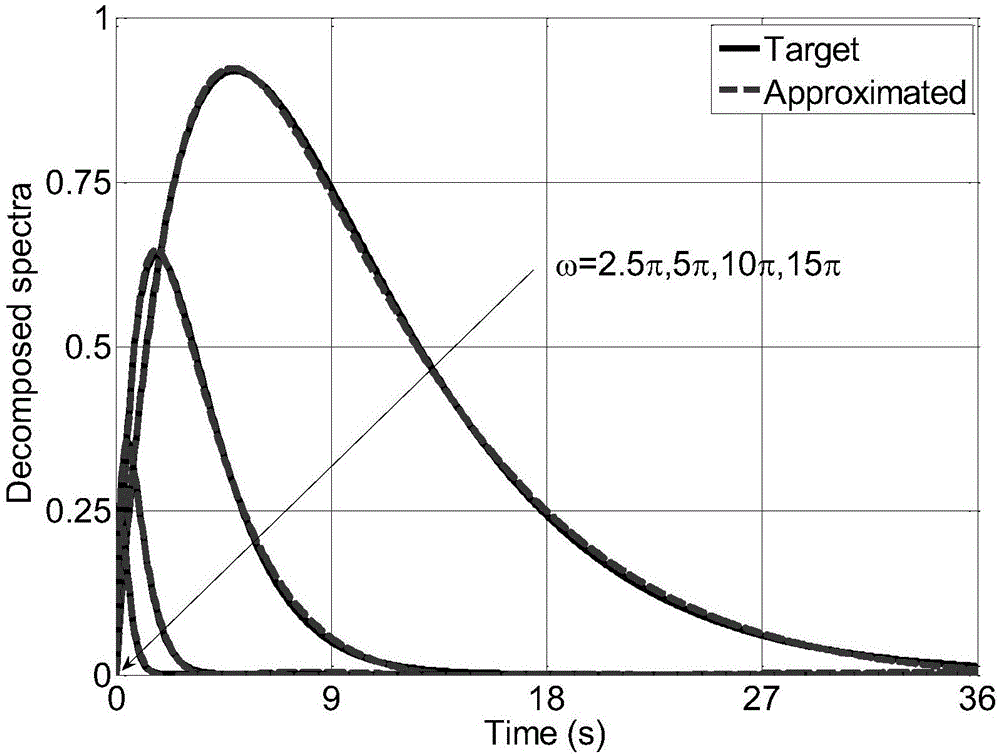
[0170] In the formula: S 0 =1. For simulation points j=1,2…,200, parameter C j = 2.5 and D j = 0.15. For simulation points j=201,202…,401, parameter C j = 4 and D j = 0.2. figure 1 Decomposed spectrum G at four selected frequencies is shown 0 (x 200 ,ω,t) and G 0 (x 201 ,ω,t) fluctuation curve. It can be seen that there is a relatively obvious difference between the evolution spectra of the simulation points 200 and 201 . Based on the POD method in the present invention, the decoupling and reconstruction of the evolutionary power spectrum are performed, when N q = 6, the comparison between the reconstructed evolution spectrum and the target evol...

example 2

[0181] Example 2: Simulation Efficiency Comparison

[0182] In 2015, Huang applied POD to the spectral representation method of non-stationary random processes, and proposed a fast simulation method for non-stationary random processes that can use FFT. In addition, the method is highly accurate and easy to implement. For convenience of comparison, this method is defined as a POD-based method. In this embodiment, the differences in efficiency and accuracy between the present invention and the POD-based method will be compared.

[0183] For comparison, these two methods are used simultaneously to simulate the random process of earthquake motion in Example 1. In the simulation, the number of discrete frequencies in both methods is set to 1024, while the number of simulation points varies from 32 to 512. The simulations of the two methods are calculated by Matlab programming, and run on a 64-bit computer with Intel(R) Xeon(R) E5-2609 v2 processor (frequency 2.50GHz) and 32GB me...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a fast simulation method for a non-stationary random process. The fast simulation method includes the steps: firstly, establishing the relationship between the multi-point non-stationary random process and a one-dimensional non-stationary random wave, namely, acquiring the two-dimensional evolutionary power spectrum density of a transformation random wave according to the evolutionary power spectrum and the coherence function of a given target random process; secondly, decoupling 2D EPSD by a proper orthogonal decomposition method; finally, simulating the transformation random wave by two-dimensional FFT (fast Fourier transform) to obtain the corresponding target non-stationary random process. Cholesky decomposition is omitted, decoupling time based on POD (proper orthogonal decomposition) is shortened, and the two-dimensional FFT technology is used, so that simulation efficiency is greatly improved. In addition, when parameters are properly selected, precision is still is high. In conclusion, the fast simulation method has the advantages of easiness in use and high precision and simulation efficiency, and can effectively solve the problem that the simulation efficiency of a spectral representation method is low when non-stationary random process simulation points are large.

Description

technical field [0001] The invention belongs to the field of random signal simulation, and in particular relates to a fast simulation method for a non-stationary random process by mixing random waves and intrinsic orthogonal decomposition. Background technique [0002] Fluctuating wind speed and ground motion usually exhibit non-stationary characteristics, so they can be described by non-stationary stochastic processes. The evolutionary power spectrum concept (evolutionary PSD or EPSD) proposed by Priestley in 1965 is widely used to describe these stochastic excitations (removal of the mean component). Compared with the time series model and the time-varying spectrum based on wavelet transform, EPSD reflects the change of spectral energy with time, and more importantly, it has obvious physical meaning. In addition, EPSD makes frequency domain response analysis and simulation of non-stationary random processes easier. [0003] Compared with time-domain methods such as time-...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06F17/50
CPCG06F30/20
Inventor 彭留留黄国庆刘瑞莉赵宁姜言
Owner SOUTHWEST JIAOTONG UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com