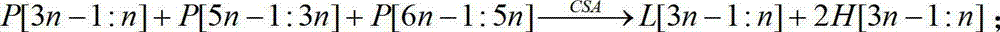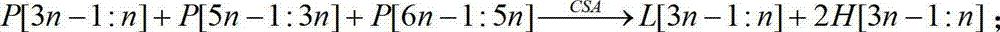Modular multiplier
A technology of multiplier and binary multiplier, which is applied in the direction of instruments, electrical digital data processing, digital data processing components, etc., can solve the problems of resource consumption and low speed, and achieve the effect of improving the operation speed
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment Construction
[0015] The present invention will be further elaborated below in conjunction with the accompanying drawings and specific embodiments.
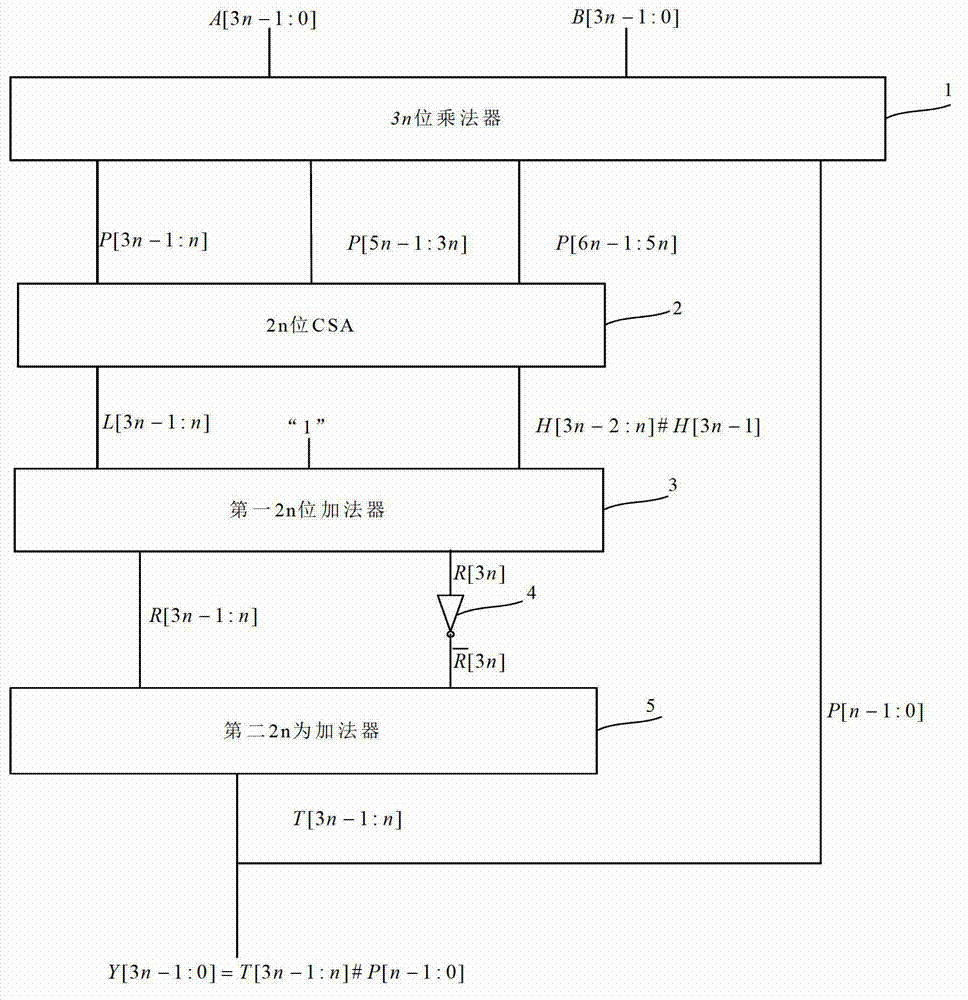
[0016] The mold of the present invention (2 3n -2 n ) multiplier structure such as figure 1 As shown, wherein, 1 is a 3n-bit binary multiplier, 2 is a 2n-bit CSA compressor array, 3 is the first 2n-bit binary adder, 4 is a 1-bit inverter, and 5 is the second 2n-bit binary adder, A[3n-1:0] and B[3n-1:0] are the input of 1, P[6n-1:0] is the output of 1; P[6n-1:5n], P[5n-1: 3n] and P[3n-1:n] are the input of 2, L[3n-1:n] and H[3n-1:n] are the output of 2; L[3n-1:n] and H[3n -2:n]#H[3n-1] is the input of 3, R[3n:n] is the output of 3; R[3n] is the input of 4, is the output of 4; R[3n-1:n] and is the input of 5, and T[3n-1:n] is the output of 5.
[0017] For the specific connection relationship, please refer to the summary of the invention. It should be noted that: # is a connection symbol, for example, in H[3n-2:n]#H[3n-1], H[3n-1] is the...
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com