Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Non-orthogonal Joint Diagonalized Instantaneous Blind Source Separation Method Based on Double Iteration

A technology of joint diagonalization and blind source separation, applied in the field of blind source signal separation, non-orthogonal joint diagonalization instantaneous blind source separation based on double iteration, can solve singular solutions, high computational complexity and computational complexity Low-level problems, to achieve the effect of simplifying modeling difficulty, good convergence performance, and fast convergence speed

Inactive Publication Date: 2017-05-17
XIDIAN UNIV
View PDF2 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0007] Aiming at the disadvantages of the existing joint diagonalization algorithm, such as easy to generate singular solutions and high computational complexity, a non-orthogonal joint pair based on double iterations with fast convergence speed, good convergence performance and relatively low computational complexity is provided. Keratinized Transient Blind Source Separation Method

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Non-orthogonal Joint Diagonalized Instantaneous Blind Source Separation Method Based on Double Iteration
  • Non-orthogonal Joint Diagonalized Instantaneous Blind Source Separation Method Based on Double Iteration
  • Non-orthogonal Joint Diagonalized Instantaneous Blind Source Separation Method Based on Double Iteration

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0061] The present invention has carried out innovation and research on the problems of unavoidable trivial solutions and degenerate solutions and high computational complexity existing in blind source separation in the prior art. The present invention is a non-orthogonal joint pair based on double iteration Keratinized Transient Blind Source Separation Method, see figure 2 , the instantaneous blind source signal separation process of the present invention comprises:

[0062] Step 1. First, record multiple observation signal data for the observation target, and divide them into L different time points t 1 ,...,t L or L different time delays τ 1 ,…,τ L The corresponding observation signal data is recorded.

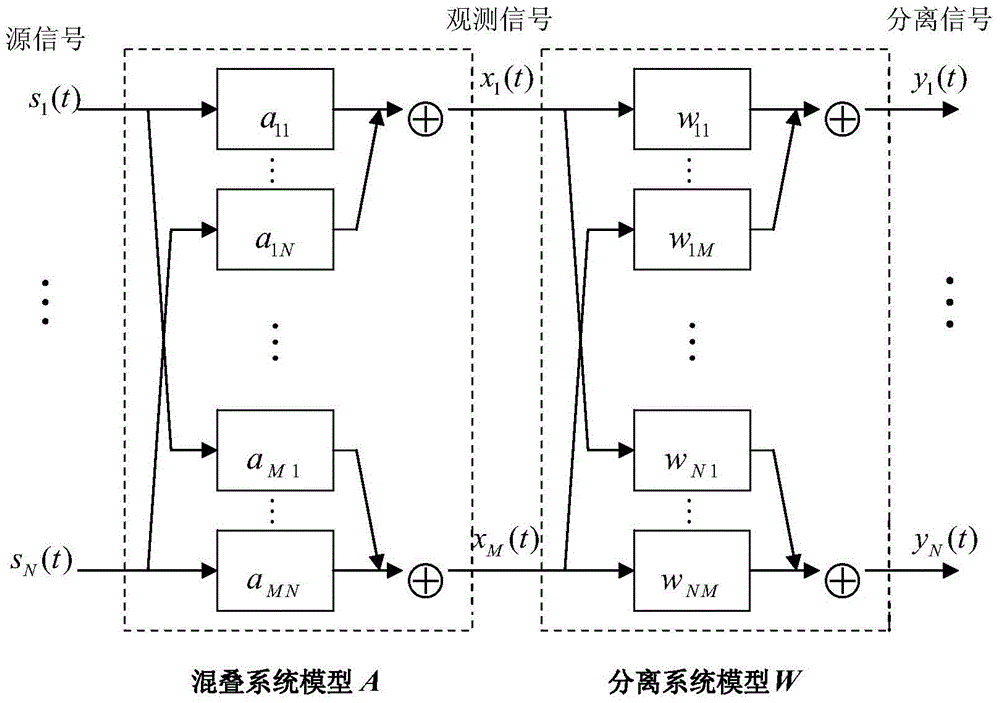
[0063] A linear aliasing and separation signal model for blind source separation see figure 1 , assuming there are N mutually statistically independent source signals s j (t),j=1,2,...,N, through the linear instantaneous aliasing system A, the array composed of M se...

Embodiment 2

[0104] The non-orthogonal joint diagonalized instantaneous blind source separation method based on double iteration is the same as that in embodiment 1, assuming that a blind source separation system applied to noise separation inputs 3 mutually uncorrelated noise source signals as source signals, and outputs 5 Observation signal, in the case of additive noise, the aliasing model of the aliasing system of the real observation signal after linearization Through the double iterative algorithm in the present invention, the optimal left aliasing matrix is ​​calculated as by formula The optimal estimated aliasing model of the observed signal is obtained as In order to prevent the occurrence of algorithm divergence or singular solutions, both the left and right aliasing matrices are normalized in the double iteration process, so the (|·|represents the modulus value of the matrix), where The reason why A / 3 is not a strict identity matrix is ​​affected by additive noise and...

Embodiment 3

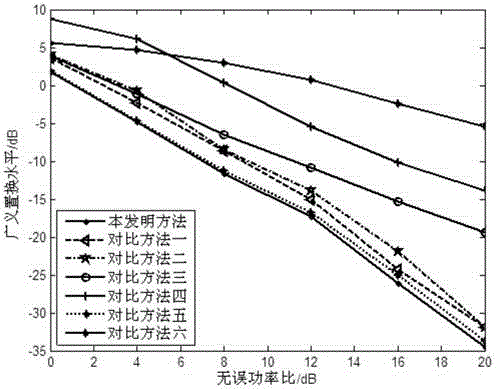
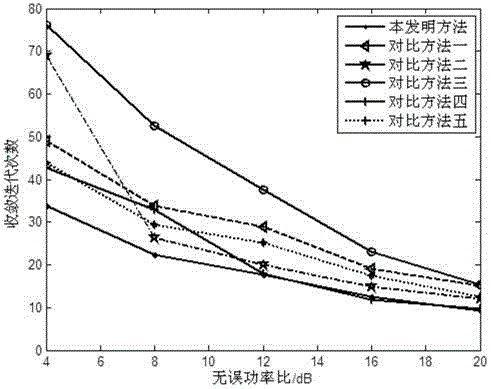
[0106] Non-orthogonal joint diagonalization instantaneous blind source separation method based on double iteration is the same as embodiment 1-2, see Figure 4 , when the double iterative algorithm in the present invention iterates one step, the value of the left aliasing matrix U is first given as U(i), so that the right aliasing matrix V(i)=U(i), by calculating and yields a diagonal matrix set with the autocorrelation coefficients of the source signals as diagonal elements via calculations and The value of the left aliasing matrix U after the single-step iteration is U(i+1)=P(i)Q -1 (i), and normalize it. Referring to Table 1, it is the comparison of the computational complexity of the double iterative algorithm of the present invention and the existing multiple non-orthogonal JD algorithms during the single-step iteration described above, represented by the number of multiplication and division operations, referred to as NMD, if the number of observation signals T...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a non-orthogonal joint diagonalization instantaneous blind source separation method based on double iteration. The method comprises the steps that signal data of repeated observation are recorded; a target matrix group based on observation signals is calculated; an observation signal aliasing model is divided into left and right aliasing matrixes; a blind source separation cost function is established; under the condition of equal left and right aliasing matrix nature, the cost function is reconstructed into a symmetric fitting function; a double iteration method is used to estimate left and right aliasing matrix optimal values required by non-orthogonal diagonalization; and an optimal aliasing model is derived to acquire an isolation signal, and instantaneous aliasing blind separation is realized. High demands of the existing non-orthogonal JD method on a target matrix are avoided, and the application scope of the observation signals is expanded. Compared with the prior art, the method provided by the invention has the advantages that the calculation complexity and the iteration number are significantly reduced; the least time is consumed; and when the same SNR is input, the shortest convergence time, the maximum output SINR and the minimum generalized replacement level are realized, and better separation performance and convergence are realized.

Description

technical field [0001] The invention belongs to the technical field of signal processing, and mainly relates to non-orthogonal joint diagonalization processing of blind signals, specifically a non-orthogonal joint diagonalization instantaneous blind source separation method based on double iteration, which is applied in the aliasing mode as Blind source signal separation during instantaneous aliasing has applications in the fields of radar signal processing and speech recognition. Background technique [0002] In many fields such as radio communication and medical imaging, it is necessary to analyze the various performance characteristics of the source signal through the received observation signal. How to separate or restore the unknown source signal in the aliasing system has gradually become a major issue in the field of signal processing technology. Research hotspots, such as when processing radar signals, do not know the transmitted signal and transmission channel, but ...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Patents(China)
IPC IPC(8): H04L25/03G10L21/0272
Inventor 冯大政刘玉胡张华杨振伟袁海璐陈跃维
Owner XIDIAN UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com