Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Sparse representation and parameterization-based curved surface fitting method

A sparse representation and surface fitting technology, applied in the field of surface fitting based on sparse representation and parameterization, can solve the problems that cannot be embedded in two-dimensional Euclidean space

Inactive Publication Date: 2017-03-08
合肥阿巴赛信息科技有限公司
View PDF2 Cites 4 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

Moreover, for geometric objects in three-dimensional space, except for special signals, they generally cannot be embedded in a regular two-dimensional Euclidean space

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Sparse representation and parameterization-based curved surface fitting method
  • Sparse representation and parameterization-based curved surface fitting method
  • Sparse representation and parameterization-based curved surface fitting method

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

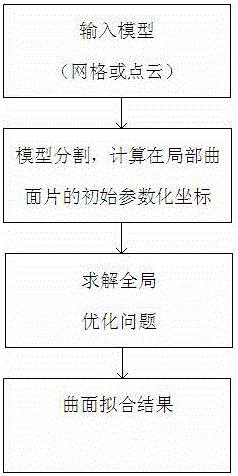
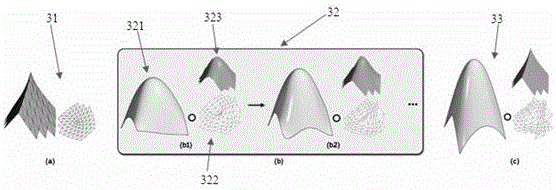
[0051] figure 2 Some monomial basis functions are shown; image 3 It is an overview diagram of the iterative solution of the method algorithm of the present invention, and the basic iterative steps are as follows:

[0052] Step 31: Read the input model, and use the LSCM method to calculate the initial parameterized coordinates.

[0053]Step 32: iterative optimization solution. Fix the initial parameterized coordinates, optimize the linear combination coefficient of the sparse representation, and the combination effect of the basis functions is shown in 321; after fixing the sparse representation, optimize the parameter domain 322; after completing one iteration, the composite representation of the sparse representation and the parameterized coordinates is as follows 323 shown.

[0054] Step 33: The optimization is completed, and the final surface fitting result 33 is obtained.

[0055] The present invention provides a simple interactive interface, such as Figure 4 As sh...

Embodiment 2

[0062] An immediate application of the present invention is point cloud reconstruction.

[0063] Such as Figure 7 As shown, the steps are as follows:

[0064] Step 71: Read the input point cloud model.

[0065] Step 72: Through the existing reconstruction method, the output is a discrete grid to obtain a simple triangular structure 72; project the point cloud to the structure 72 to obtain the local center of gravity coordinates of each point in the projected triangle; as in the complex model of embodiment 1 The processing method is to segment the structure 72 and perform parameterization, obtain the initial value of the parameterized coordinates of the point cloud according to the barycenter coordinates of the point cloud, and then use all fixed points of the point cloud as input signals to solve the problem.

[0066] 73: The optimization is completed, and the final surface fitting result 73 is obtained.

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a sparse representation and parameterization-based curved surface fitting method. The method comprises the following steps of inputting a model; segmenting the model, calculating initial parameterization coordinates of a local curved surface segment, optimizing a linear combination coefficient of sparse representation, and performing parameter optimization to obtain combined function optimization; solving a global optimization problem; and obtaining a curved surface fitting result. According to the sparse representation and parameterization-based curved surface fitting method, a simple monomial function is used as a basis function, good approximation for different geometric characteristics is realized through introduction of parameter optimization, and the input model can be a three-dimensional mesh model or point cloud; and the combined function optimization model is obtained in combination with the optimization of the linear combination coefficient of sparse representation and the parameter optimization, and the solving is performed in a cyclical iteration manner by introducing an auxiliary quantity.

Description

technical field [0001] The invention belongs to the technical field of machine learning and optimization, in particular to a surface fitting method based on sparse representation and parameterization. Background technique [0002] Sparse representation assumes that the input signal can be represented by a set of redundant base signals, and requires this representation to be sparse, that is, the input signal is expressed by a very small number of base signals. This representation is widely used in machine learning, computer vision, and pattern recognition, and is the basis of many learning algorithms, such as dictionary learning, deep learning, neural networks, object recognition, image denoising, image upsampling, etc. [0003] Given the input signal, how to select a very small number of base signals to represent is an integer optimization problem, which is an NP-hard problem, and the optimal algorithm cannot be realized in polynomial time. Naturally, many approximate algori...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06T17/00G06T17/20
CPCG06T17/00G06T17/20
Inventor 张朋
Owner 合肥阿巴赛信息科技有限公司
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com