Nonlinear manifold learning dimension reduction method based on adaptive density clustering

A density clustering algorithm and density clustering technology, applied in the fields of instruments, character and pattern recognition, computer parts, etc., can solve the problems of poor reliability, low accuracy, distortion, etc., to overcome the inability to automatically determine and correct High rate and good reliability

Inactive Publication Date: 2017-03-22
ZHEJIANG UNIV OF TECH
View PDF0 Cites 7 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0005] In order to overcome the deficiencies of ordinary dimensionality reduction methods, such as distorting the popularity after dimensionality reduction, "deformation" in the structure after unfolding, low accuracy rate, and poor credibility, the present invention provides a method with high accuracy rate and credibility A better nonlinear popular learning di

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Nonlinear manifold learning dimension reduction method based on adaptive density clustering
  • Nonlinear manifold learning dimension reduction method based on adaptive density clustering
  • Nonlinear manifold learning dimension reduction method based on adaptive density clustering

Examples

Experimental program
Comparison scheme
Effect test

Embodiment Construction

[0036] The present invention will be further described below in conjunction with the accompanying drawings.

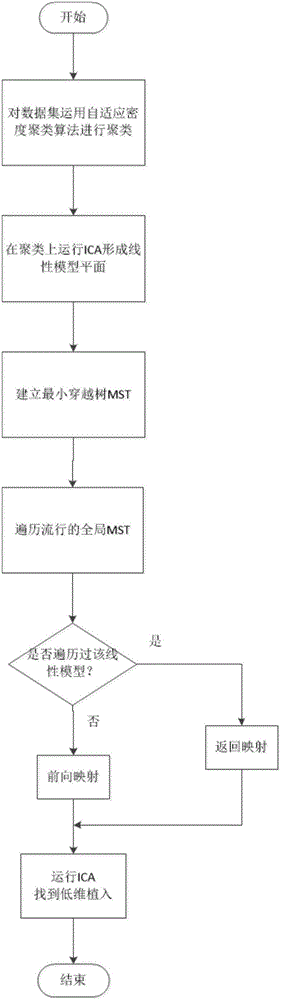
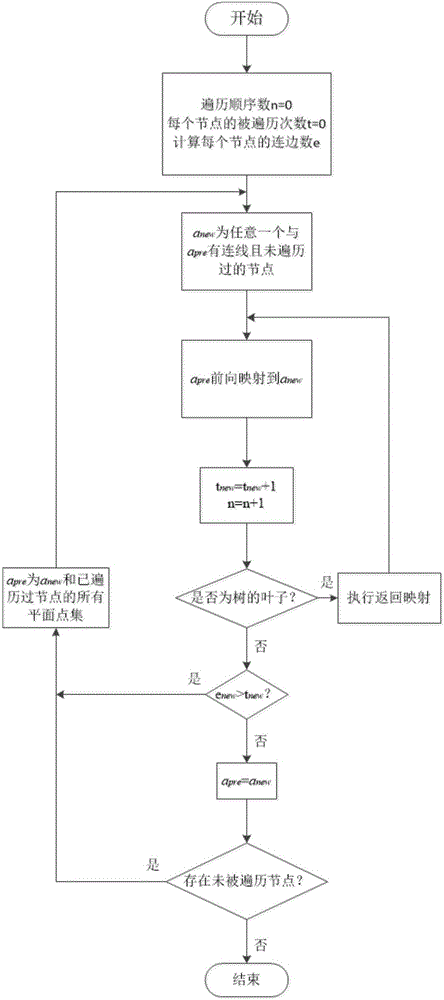
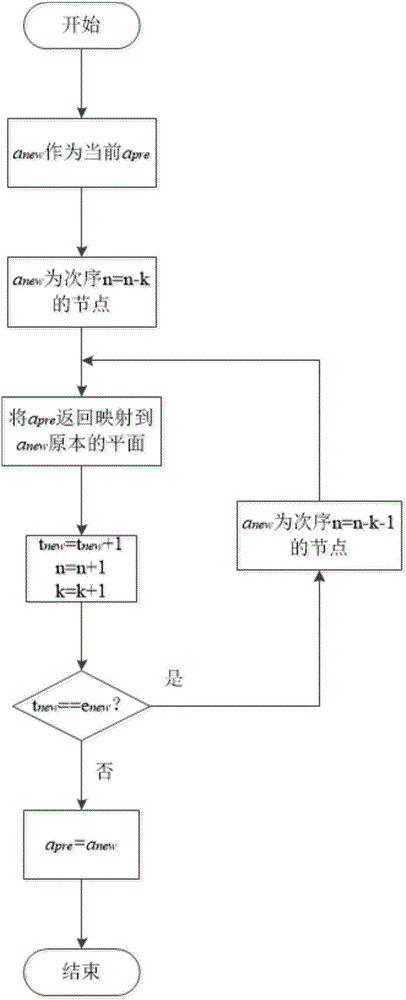
[0037] refer to Figure 1 to Figure 5 , a nonlinear popular learning dimensionality reduction method based on adaptive density clustering, has high-dimensional samples M, and each sample of M is a point x, which is usually an object of mathematical representation. Sampling from the embedded low-dimensional fashion (n<

[0038] refer to figure 1 As shown, the nonlinear popular learning dimensionality reduction method based on parallel mapping, the dimensionality reduction method includes the following steps:

[0039] 1) Use the clustering method to form a linear model plane, the process is as follows:

[0040] 1.1 Use the adaptive density clustering algorithm to cluster the data objects in the popular M, automatically dete...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to view more

PUM

No PUM Login to view more

Abstract

The invention discloses a nonlinear manifold learning dimension reduction method based on adaptive density clustering. The method comprises the following steps: 1) after the adaptive density clustering algorithm is used for clustering, ICA (Independent Component Analysis) is used for carrying out dimension reduction on each cluster to form a linear model plane; 2) the minimum spanning tree (MST) between local linear models is built; 3) manifold global MSTs are transversed; and 4) through operating the ICA on a global hyperplane, low dimensional implantation is found out. According to the nonlinear manifold learning dimension reduction method based on adaptive density clustering, the parallel mapping of the plane is used for overcoming the distortion generated by dimension reduction of the original data set, the accuracy is high, and the credibility is good.

Description

technical field [0001] The present invention belongs to a nonlinear data dimensionality reduction method. Aiming at the difficulties in directly carrying out data mining and analysis on high-dimensional data in current big data applications, a nonlinear popular method based on adaptive density clustering is proposed by using a parallel mapping method. Learning the dimensionality reduction method and using the parallel mapping of the plane can overcome the distortion of the original data set due to dimensionality reduction. Background technique [0002] With the development of science and technology and the era of big data, data information is rapidly changing to high-dimensional. The data information generated by people in the course of behavior is no longer a simple small amount of data, but high-dimensional data containing a large amount of information, but the huge The amount of data and the high-dimensional eigenvalues ​​of each data sample bring difficulties to data pro...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to view more

Application Information

Patent Timeline
no application Login to view more
IPC IPC(8): G06K9/62
CPCG06F18/23211
Inventor 陈晋音保星彤陈心怡郑海斌
Owner ZHEJIANG UNIV OF TECH
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Try Eureka
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap