Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Joint inversion method of airborne transient electromagnetic data and airborne magnetotelluric data

A transient electromagnetic and magnetotelluric technology, applied in the field of geophysical exploration, can solve the problems of low aerial MT resolution, shallow aerial TEM exploration depth, and inability to obtain deep electrical distribution of underground media, so as to overcome low depth and improve accuracy Effect

Active Publication Date: 2020-09-08
CHENGDU UNIVERSITY OF TECHNOLOGY
View PDF6 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0008] In order to overcome the defects of the above-mentioned prior art, the present invention provides a joint inversion method of airborne transient electromagnetic data and airborne magnetotelluric data. The present invention can not only solve the problem that the aerial TEM exploration depth is shallow and the electrical distribution in the deep part of the underground medium cannot be obtained. In addition, it can solve the problem of low resolving power of aerial MT in the shallow part, so as to provide more accurate inversion and interpretation results for airborne geophysical exploration in large areas, so as to improve the comprehensive exploration efficiency

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Joint inversion method of airborne transient electromagnetic data and airborne magnetotelluric data
  • Joint inversion method of airborne transient electromagnetic data and airborne magnetotelluric data
  • Joint inversion method of airborne transient electromagnetic data and airborne magnetotelluric data

Examples

Experimental program
Comparison scheme
Effect test

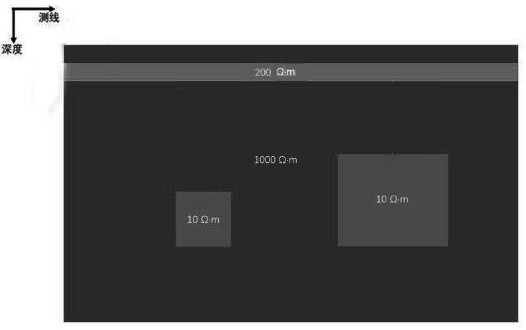
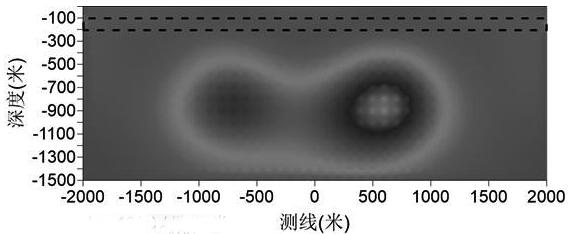
Embodiment 1

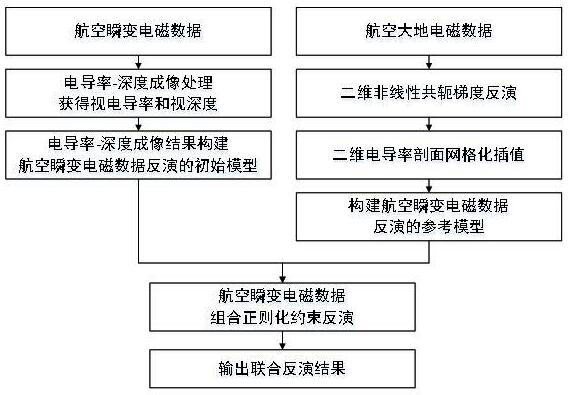
[0037] The joint inversion method of airborne transient electromagnetic data and airborne magnetotelluric data includes the following steps:

[0038] a. Use the nonlinear conjugate gradient inversion method to perform two-dimensional inversion processing on the airborne magnetotelluric data, and obtain a two-dimensional conductivity profile result. The objective function of the inversion is obtained by formula 1;

[0039] Φ(m)=(d-F(m)) T V -1 (d-F(m))+λm T LT Lm formula 1

[0040] Among them, λ is the regularization factor; V is the covariance matrix related to the error vector e; L is the two-dimensional differential matrix related to the model parameter m; d is the observed data; F(m) is the forward numerical simulation value; m is the model parameter vector; T is the transpose of the matrix;

[0041] b. Convert the reciprocal of the resistivity in the two-dimensional conductivity profile results into conductivity, and perform gridding to form regular conductivity grid d...

Embodiment 2

[0051] The joint inversion method of airborne transient electromagnetic data and airborne magnetotelluric data includes the following steps:

[0052] a. Use the nonlinear conjugate gradient inversion method to perform two-dimensional inversion processing on the airborne magnetotelluric data, and obtain a two-dimensional conductivity profile result. The objective function of the inversion is obtained by formula 1;

[0053] Φ(m)=(d-F(m)) T V -1 (d-F(m))+λm T L T Lm formula 1

[0054] Among them, λ is the regularization factor; V is the covariance matrix related to the error vector e; L is the two-dimensional differential matrix related to the model parameter m; d is the observed data; F(m) is the forward numerical simulation value; m is the model parameter vector; T is the transpose of the matrix;

[0055] b. Convert the reciprocal of the resistivity in the two-dimensional conductivity profile results into conductivity, and perform gridding to form regular conductivity grid...

Embodiment 3

[0066] The joint inversion method of airborne transient electromagnetic data and airborne magnetotelluric data includes the following steps:

[0067] a. Use the nonlinear conjugate gradient inversion method to perform two-dimensional inversion processing on the airborne magnetotelluric data, and obtain a two-dimensional conductivity profile result. The objective function of the inversion is obtained by formula 1;

[0068] Φ(m)=(d-F(m)) T V -1 (d-F(m))+λm T L T Lm formula 1

[0069] Among them, λ is the regularization factor; V is the covariance matrix related to the error vector e; L is the two-dimensional differential matrix related to the model parameter m; d is the observed data; F(m) is the forward numerical simulation value; m is the model parameter vector; T is the transpose of the matrix;

[0070] b. Convert the reciprocal of the resistivity in the two-dimensional conductivity profile results into conductivity, and perform gridding to form regular conductivity grid...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a joint inversion method for aviation transient electromagnetic data and aviation magnetotelluric data, and belongs to the technical field of geophysical exploration. The jointinversion method comprises the following steps of step a, performing two-dimensional inversion processing on the aeromagnetotelluric data by adopting a nonlinear conjugate gradient inversion method;step b, taking a reciprocal of the resistivity to convert the reciprocal into the conductivity, and meshing to form regular conductivity grid data; step c, constructing a reference model for the one-dimensional inversion of the aviation transient electromagnetic data; step d, obtaining the apparent conductivity and the apparent depth of an underground medium; step e, constructing an initial modelfor the one-dimensional inversion of the aviation transient electromagnetic data; step f, restraining and completing the one-dimensional combined regularization constrained inversion of the aviation transient electromagnetic data; and step g, outputting an inversion result of an aviation transient electromagnetic and aerospace magnetotelluric joint interpretation. According to the joint inversionmethod for the aviation transient electromagnetic data and the aviation magnetotelluric data provided by the invention, an inversion interpretation result with higher precision can be provided for theaviation geophysical exploration in large-area regions, so that the comprehensive exploration efficiency is improved.

Description

technical field [0001] The invention relates to the technical field of geophysical exploration, in particular to a joint inversion method of aerial transient electromagnetic data and aerial magnetotelluric data. Background technique [0002] Aeronautical geophysics is a geophysical exploration technology that uses aircraft platforms to carry geophysical exploration equipment and conduct observations in the air. It has the advantages of large detection area, fast speed, and relatively low cost. In complex areas such as Gobi, sea area, high mountains and forest cover, where it is difficult for ground personnel to carry out exploration operations, airborne geophysical exploration can achieve better exploration results. [0003] Airborne transient electromagnetic method (TEM) is widely used in airborne geophysics, and it is also a fast-growing exploration method. In the past ten years since entering the 21st century, airborne TEM has been used in observational instruments, data ...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Patents(China)
IPC IPC(8): G01V3/38
CPCG01V3/38
Inventor 王绪本赵宁余小东杨聪
Owner CHENGDU UNIVERSITY OF TECHNOLOGY
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com