Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

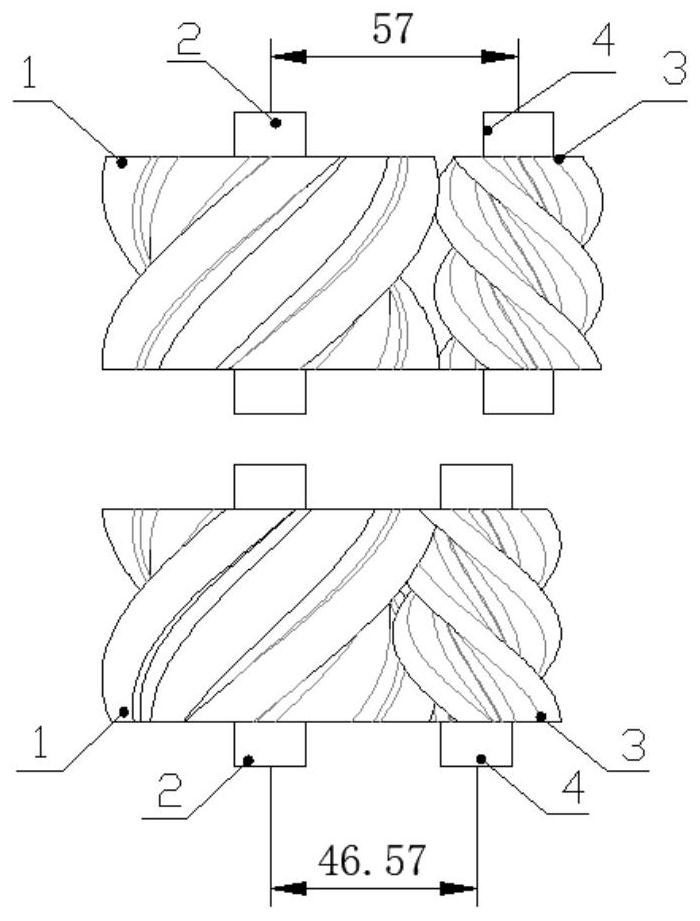
Non-sliding parallel shaft line gear mechanism with separable center distance

A technology of gear mechanism and center distance, applied in gear transmission, belt/chain/gear, mechanical equipment, etc., can solve the problems of lack of center distance separability, center distance error affecting the performance of line gear transmission, etc.

Pending Publication Date: 2021-08-20
SOUTH CHINA UNIV OF TECH
View PDF0 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

All the previously designed wire gear pairs do not have the separability of the center distance, and the center distance error affects the transmission performance of the wire gear

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Non-sliding parallel shaft line gear mechanism with separable center distance
  • Non-sliding parallel shaft line gear mechanism with separable center distance
  • Non-sliding parallel shaft line gear mechanism with separable center distance

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

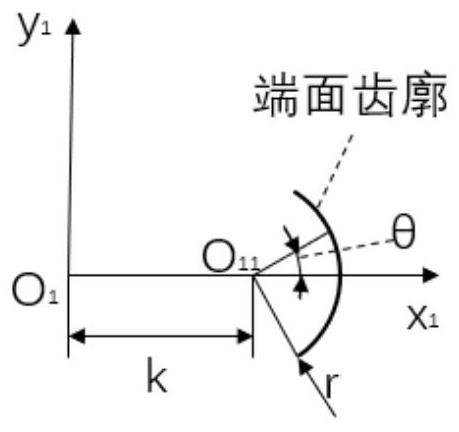
[0064] Given that the eccentricity k of the eccentric arc of the tooth profile of the driving wheel is 30, the radius r of the eccentric arc of the driving wheel is 8, and the variation range of the variable parameter of the eccentric arc of the driving wheel is 0°≤θ≤90°, the determined eccentricity of the driving wheel is obtained Arc tooth profile.

[0065] Further, given that the contact line pitch parameter is 50.92, it can be determined that when θ is 0, the corresponding The contact line is

[0066]

[0067] Further, the eccentric arc tooth profile of the driving wheel extends along the Sweep the contact line to get the tooth surface of the driving wheel tooth 1, and the equation of the tooth surface of the driving wheel tooth 1 in its own coordinate system is:

[0068]

[0069] -90°≤θ≤90°

[0070] 0≤t≤1.2π

[0071] Further, given that the transmission ratio is 0.5, the eccentricity of the eccentric arc of the driven wheel tooth profile and the radius of the ...

Embodiment 2

[0080] Given that the eccentricity k of the eccentric arc of the tooth profile of the driving wheel is 50, the radius r of the eccentric arc of the driving wheel is 8, and the variable parameter range of the eccentric arc of the driving wheel is 0°≤θ≤90°, the determined eccentricity of the driving wheel is obtained Arc tooth profile.

[0081] Further, given that the contact line pitch parameter is 100.46, it can be determined that when θ is 0, the corresponding The contact line is

[0082]

[0083] Further, the eccentric arc tooth profile of the driving wheel extends along the Sweep the contact line to get the tooth surface of the driving wheel tooth 1. Given the change range of the contact line length parameter is 0≤t≤0.4π, the equation of the driving wheel tooth 1 tooth surface in its own coordinate system is:

[0084]

[0085] -90°≤θ≤90°

[0086] 0≤t≤0.4π

[0087] Further, given that the transmission ratio is 0.5, the eccentricity of the eccentric arc of the dri...

Embodiment 3

[0096] Given that the eccentricity k of the eccentric arc of the tooth profile of the driving wheel is 40, the radius r of the eccentric arc of the driving wheel is 5, and the variation range of the variable parameter of the eccentric arc of the driving wheel is 0°≤θ≤90°, the determined eccentricity of the driving wheel is obtained Arc tooth profile.

[0097] Further, given that the contact line pitch parameter is 77.94, it can be determined that when θ is 0, the corresponding The contact line is

[0098]

[0099] Further, the eccentric arc tooth profile of the driving wheel extends along the Sweep the contact line to get the tooth surface of the driving wheel tooth 1. Given the change range of the contact line length parameter is 0≤t≤0.4π, the equation of the driving wheel tooth 1 tooth surface in its own coordinate system is:

[0100]

[0101] -90°≤θ≤90°

[0102] 0≤t≤0.4π

[0103] Further, given that the transmission ratio is 2, the eccentric distance of the ecce...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a non-sliding parallel shaft line gear mechanism with separable center distance. The mechanism comprises a transmission pair composed of a pair of parallel shaft line gears, including a driving wheel and a driven wheel, wherein the driving wheel and the driven wheel are each composed of a wheel body and line teeth, and the tooth profile of the end face of the line teeth is an eccentric arc tooth profile designed in a parameterized mode. The line gear mechanism can realize continuous and stable non-sliding transmission under different center distance conditions within a certain range, so that the transmission ratio of line gear transmission is irrelevant to the center distance of the two gears, the transmission ratio is not affected by errors of the center distance, the influence of the errors of the center distance on transmission performance is reduced, and the mechanism is particularly suitable for occasions such as micro-transmission in which the assembly precision is difficult to ensure and the requirements on the transmission performance are high.

Description

technical field [0001] The invention relates to the field of mechanical transmission, in particular to a parallel axis gear mechanism with no sliding and separable center distance. Background technique [0002] The transmission ratio of the gear transmission has nothing to do with the center distance of the two gears, and the transmission ratio is not affected by the error of the center distance. This is the separability of the center distance of the gear transmission. At present, only involute gears are separable gears, and the rest of the gears are not separable. For involute gear pairs, increasing the center distance will lead to a decrease in the degree of coincidence. For arc gears, the center distance error greatly affects the transmission performance of the arc gear; for the gear pair with cycloidal profile, the center distance error greatly affects the transmission performance of the gear. All in all, for the gear pair whose center distance is inseparable, the cent...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): F16H1/06F16H1/24F16H55/17F16H55/08
CPCF16H1/06F16H1/24F16H55/17F16H55/0826
Inventor 陈扬枝叶长坤何超刘雾杨辅标
Owner SOUTH CHINA UNIV OF TECH
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com