Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

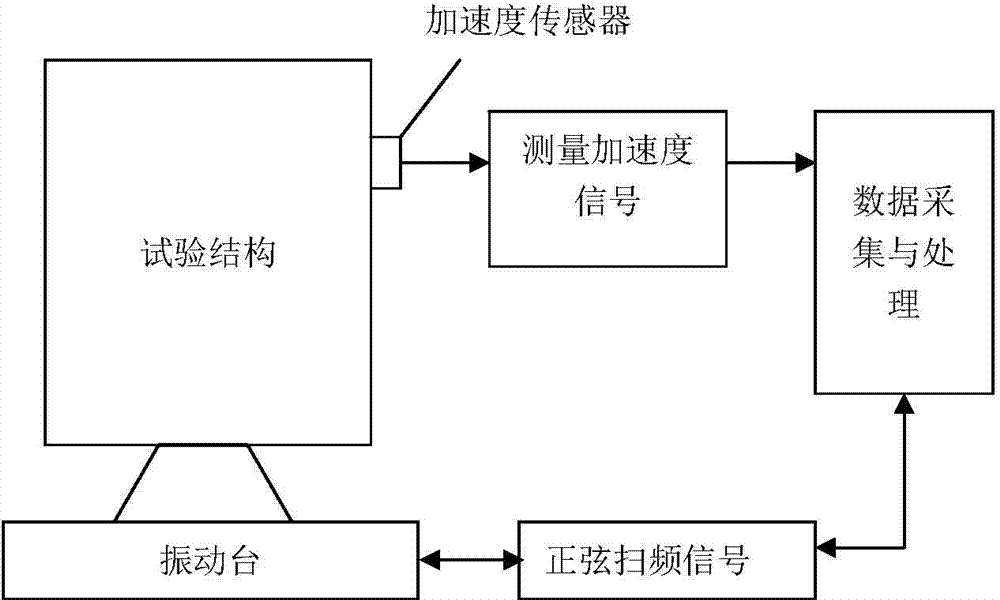
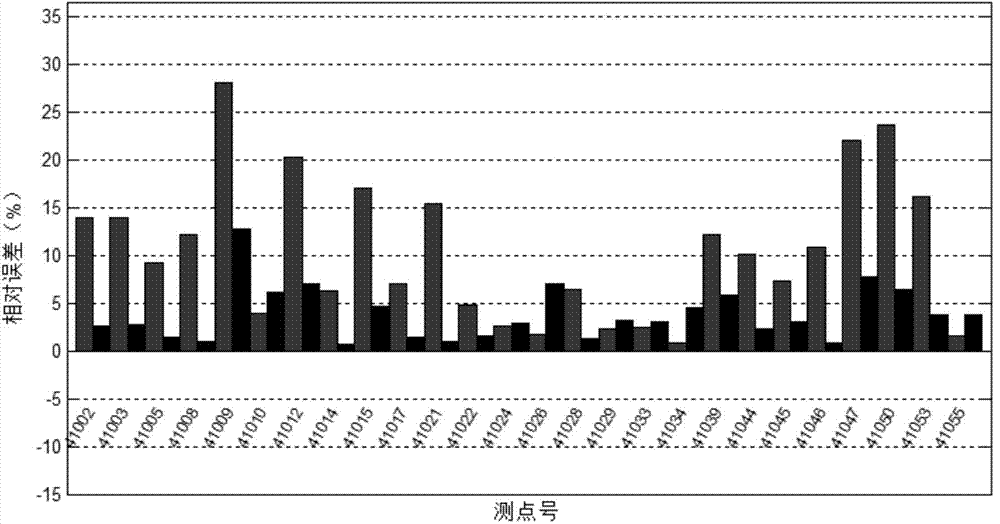
Model modification method with damp considered based on basic stimulation response data

A technology of stimulus response and model correction, applied in the direction of electrical digital data processing, special data processing applications, instruments, etc., can solve problems such as increasing test costs, reducing product design efficiency, and reducing the accuracy of dynamic response predictions

Inactive Publication Date: 2014-06-25
HARBIN INST OF TECH
View PDF3 Cites 13 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0005] The present invention is to solve the problem that the damping correction is not considered in the existing response-based model correction method, which leads to a decrease in the prediction accuracy of the model for the dynamic response, a reduction in the design efficiency of the product, and an increase in the cost of the test, and further provides a A Model Modification Method Considering Damping Based on Base Excitation Response Data

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Model modification method with damp considered based on basic stimulation response data
  • Model modification method with damp considered based on basic stimulation response data
  • Model modification method with damp considered based on basic stimulation response data

Examples

Experimental program
Comparison scheme
Effect test

specific Embodiment approach 1

[0033] Specific implementation mode 1: The damping-based model correction method based on the basic excitation response data of this implementation mode is implemented in the following steps:

[0034] 1. First, use the finite element software to establish the finite element simulation model of the system. After the finite element simulation model is established, the stiffness K, structural damping G, viscous damping C and mass matrix M in the physical matrix are exported to obtain a system considering damping Kinetic equations:

[0035] ([K]+i[G]+iω[C]-ω 2 [M]){u}={f}

[0036] Among them, the stiffness K, structural damping G, viscous damping C, and mass matrix M are partitioned according to the connection with the shaking table in the finite element simulation model, and the sub-matrix is ​​obtained:

[0037] [ K ] = [ ...

specific Embodiment approach 2

[0053] Embodiment 2: This embodiment is different from Embodiment 1 in that: the finite element software in the step 1 is MSC.Nastran.

[0054] Other steps and parameters are the same as those in Embodiment 1.

specific Embodiment approach 3

[0055] Specific embodiment three: the difference between this embodiment and specific embodiment one or two is:

[0056] In the first step, for the viscous damping C, the Rayleigh damping assumption is used to fit the viscous damping coefficient:

[0057] The damping matrix is ​​Rayleigh damping, and Rayleigh damping assumes that the damping is linearly related to the mass matrix and stiffness matrix;

[0058] C=αM+βK

[0059] where α, β are viscous damping coefficients, same as modal damping ξ i (Can be determined by modal test or identified by telemetry signal)

[0060] The relationship is as follows

[0061] ξ i = 1 2 ω i ( α + β ω i 2 )

[0062] The constants α, β were det...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a model modification method with damp considered based on basic stimulation response data and relates to model modification methods with damp considered. The model modification method with the damp considered based on the basic stimulation response data aims to solve the problem that damp modification is not considered in the prior art and comprises the steps that (1) a finite element model is established, and partitioning is conducted on a physic matrix of the model; (2) a transfer function based on basic stimulation is obtained by means of matrix transformation; (3) test data are introduced, and a deviation function of the test data and simulation data is established; (4) a model modification equation is established according to the relation between the deviation function and physic parameter deviation, and required modification can be obtained by solving the equation; (5) a modification parameter obtained in the step (4) is plugged into the finite element simulation model, and then a modified finite element simulation model is obtained. The model modification method with the damp considered based on the basic stimulation response data is applied to the field of finite element simulation of structures as well as the relevant fields such as the test field and the design field.

Description

technical field [0001] The present invention relates to a method of model revision considering damping. Background technique [0002] With the continuous development of industrial technology, the traditional method of product design relying on experimental technology is far from meeting the needs of designers. Utilizing the modern high-speed development of computer technology, it has been widely used by designers to realize the prediction of products through computer simulation. Finite element technology is a computer simulation technology with strong engineering practical application value. The use of finite element model can quickly realize the prediction of various mechanical environments and characteristics of products. [0003] Finite element model correction is an important research content in the field of modern mechanical simulation, especially for large and complex structures (satellites, rockets, spacecraft, etc.), model correction can effectively improve the pred...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06F17/50
Inventor 袁昭旭于开平郑重韩增尧邹元杰
Owner HARBIN INST OF TECH
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com