Polynomial Method of Unscented Kalman Filter Based on Higher Order Moment Matching
A technology of unscented Kalman and high-order moments, which is applied in impedance networks, digital technology networks, electrical components, etc., can solve problems such as black box packaging, large system errors, and limited precision.
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment Construction
[0064] The preferred embodiments of the present invention will be described in detail below with reference to the accompanying drawings.
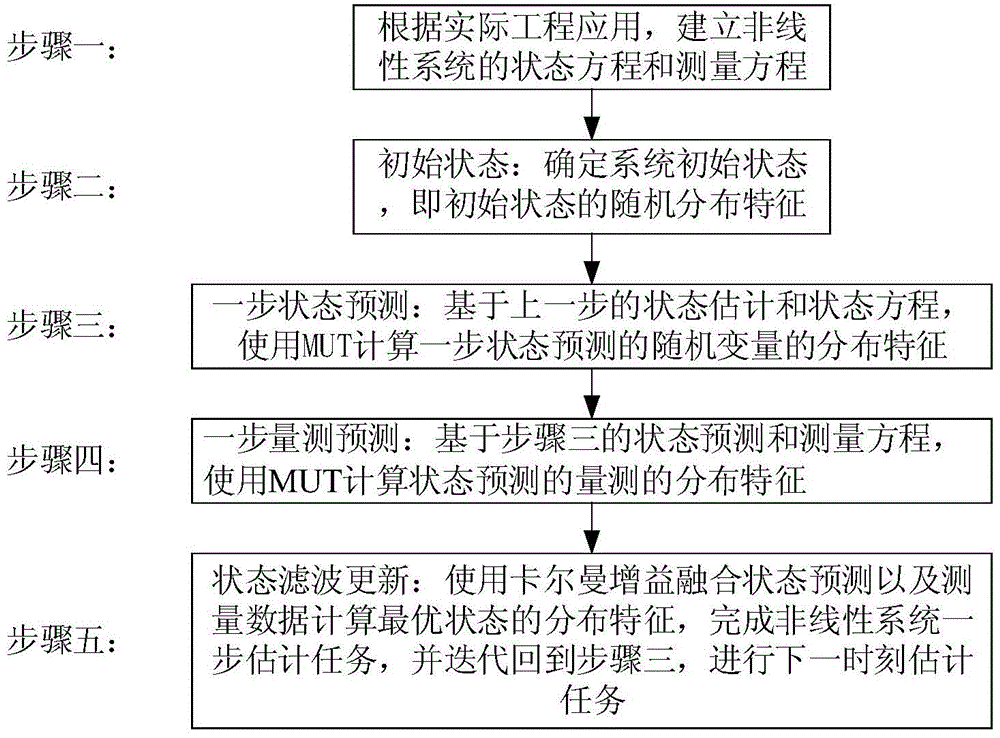
[0065] figure 1 It is a schematic flow chart of the method of the present invention, figure 2 It is a schematic diagram of the concrete steps of the method of the present invention, as shown in the figure, the concrete steps of the method are as follows:
[0066] Step 1: According to the problem of azimuth-only target tracking, the state equation and observation equation describing the target tracking system are established as follows:
[0067] x k 0.9 0 0 1 x k - 1 + w k - ...
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com