Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

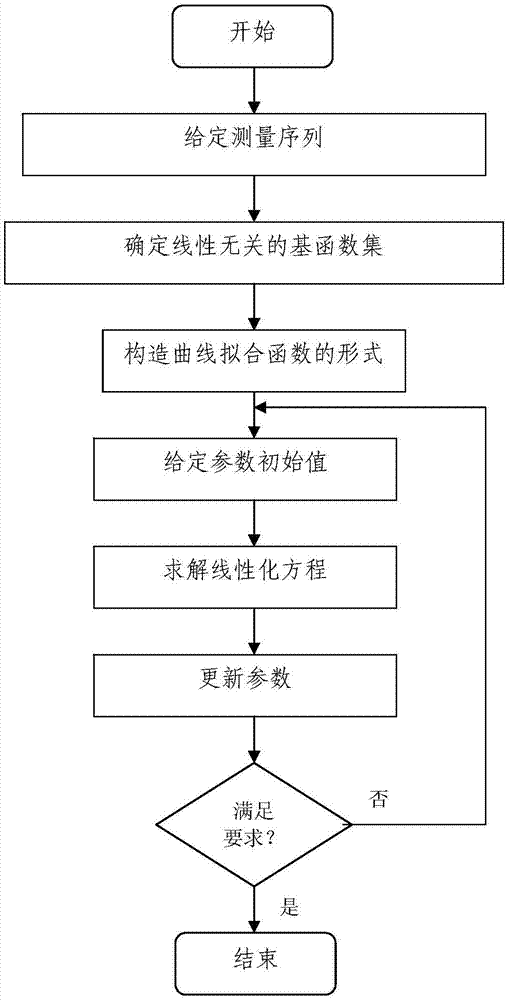
Least square method-based curve fitting method

A technology of least squares and curve fitting, applied in complex mathematical operations, etc., can solve problems such as inability to guarantee, and achieve the effect of simple method, avoiding minimal changes, and strong logic.

Inactive Publication Date: 2017-10-20
BEIJING INST OF AEROSPACE CONTROL DEVICES
View PDF0 Cites 5 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0023] Analyzing the reasons, the main reason is that the least square method can only guarantee minimum, but not guaranteed the smallest

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Least square method-based curve fitting method
  • Least square method-based curve fitting method
  • Least square method-based curve fitting method

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

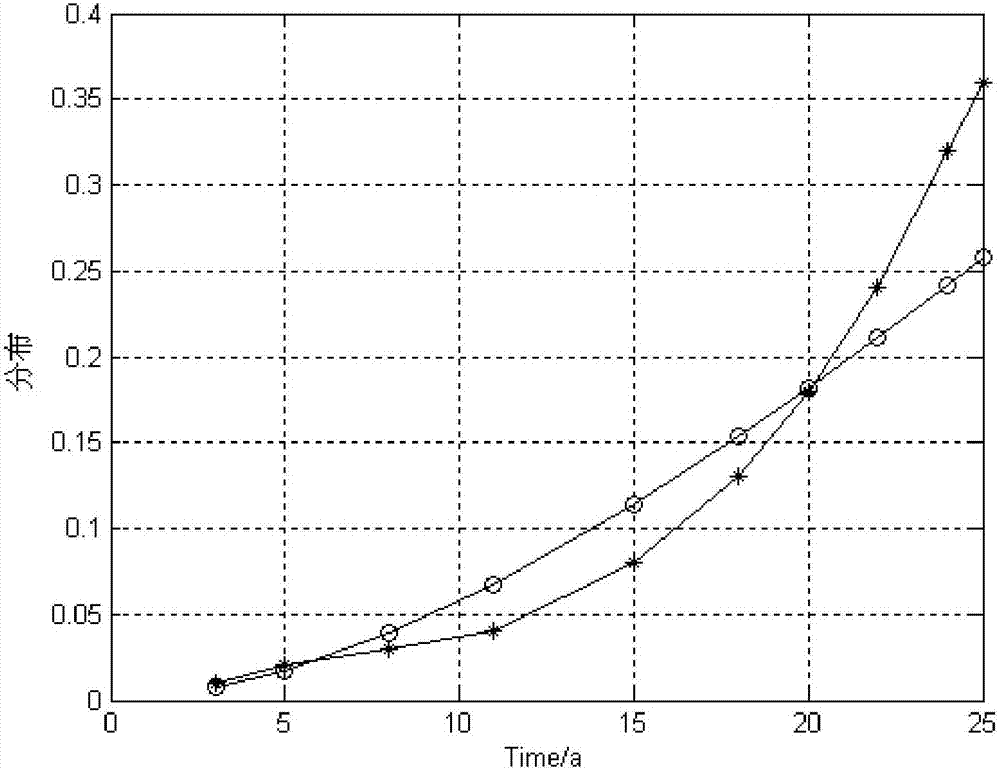
[0091] Taking Table 1 in the reference ("Assessment of accelerometer storage life under temperature stress", "Journal of Armored Forces Engineering College", Vol.28 No.3, 2014, P28) as an example, the inertial instrument—accelerometer is set at The failure data of the natural storage environment test under the condition of 25°C (the sample size of each test is 100), the test result is

[0092] Table 1 Statistical situation of test failure data

[0093]

[0094]

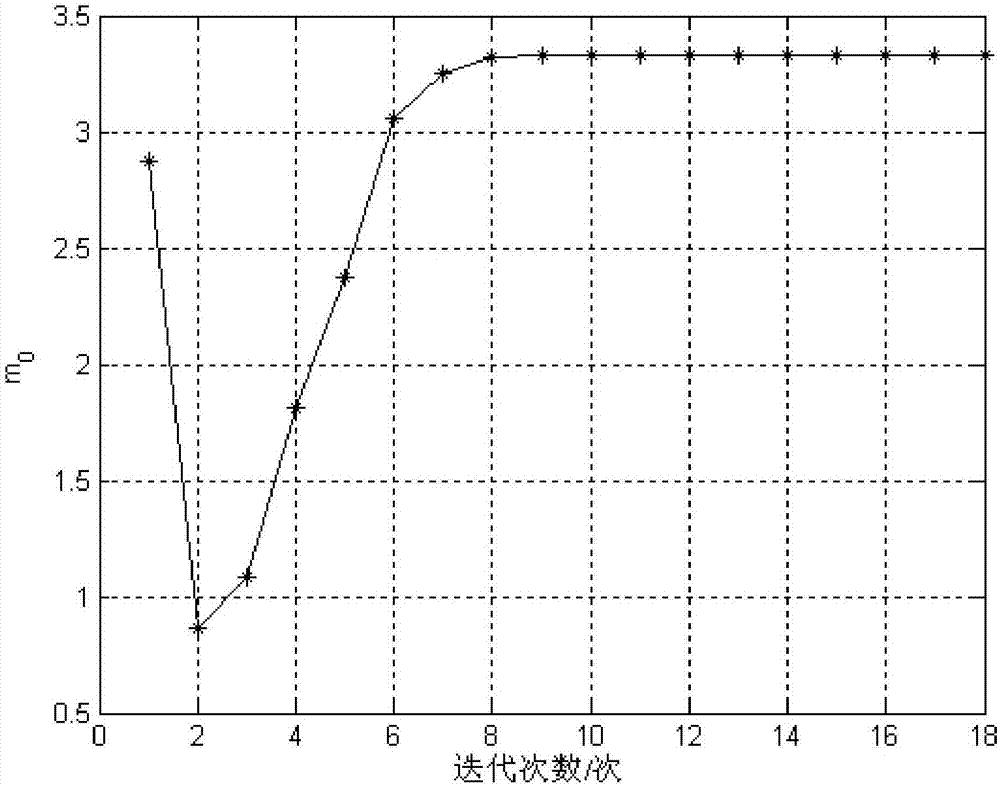
[0095] Using the Weibull distribution , solve ln(-ln(1-F))=m ln(T)-m ln(η) by least squares method and provide the solution of step (3) with parameters m and η: m=1.7618596, η=49.748074 . Fitting curves such as figure 2 As shown, the "*" point in the figure is the experimental data in Table 1, and the "o" point is the fitted value. It can be seen that there is still a large gap between the two. at this time,

[0096] Adopt the curve fitting method based on least square method of the present invention, p...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The present invention relates to a curve fitting method based on the least squares method. The method establishes a linear model on the basis of a nonlinear fitting curve, uses an iterative calculation method to provide coefficients in the function of the fitting curve, and substitutes the coefficients into The theoretical value obtained after fitting the function has the characteristics of the minimum sum of squares of errors, and the method can accurately provide the coefficients of the fitting function by means of precise iterative calculation, so that the sum of squares of errors of fitting is minimized; the present invention avoids The minimization problem existing in the least square method in the linear transformation process is solved, and the curve fitting method provided by the invention has high fitting precision, strong logic, easy programming, simple method and easy realization.

Description

technical field [0001] The invention relates to a curve fitting method based on the least square method, which belongs to the technical field of numerical analysis and reliability. Background technique [0002] There are many functions in scientific experiments and engineering applications, whose analytical expressions are not known. For example, with the prolongation of storage time, the performance and parameters of inertial devices will change, but this change process can only be measured by a series of nodes x i value on f i . The problem now is to seek the approximation function y(x) of the test point sequence, or in geometric language, to seek a curve y(x) to fit (smooth) these n points. [0003] The traditional curve fitting method is the method of least squares, which requires [0004] [0005] in, [0006] [0007] [0008] Then call y(x) as f(x) about the weight coefficient {ω i}'s least squares approximation, and the method for finding the approxima...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06F17/15
CPCG06F17/15
Inventor 魏宗康何远清
Owner BEIJING INST OF AEROSPACE CONTROL DEVICES
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com