Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Self-adaption evaluation method for reliability of systems of cascaded structures

A serial structure, self-adaptive technology, applied in special data processing applications, instruments, design optimization/simulation, etc., can solve problems such as large calculation consumption, and achieve accurate evaluation results

Inactive Publication Date: 2017-12-15
WUHAN UNIV
View PDF0 Cites 9 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

It can be seen that there are many limitations when using the traditional structural reliability evaluation method to evaluate the reliability of the series structure system, and the calculation consumes a lot

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Self-adaption evaluation method for reliability of systems of cascaded structures
  • Self-adaption evaluation method for reliability of systems of cascaded structures
  • Self-adaption evaluation method for reliability of systems of cascaded structures

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

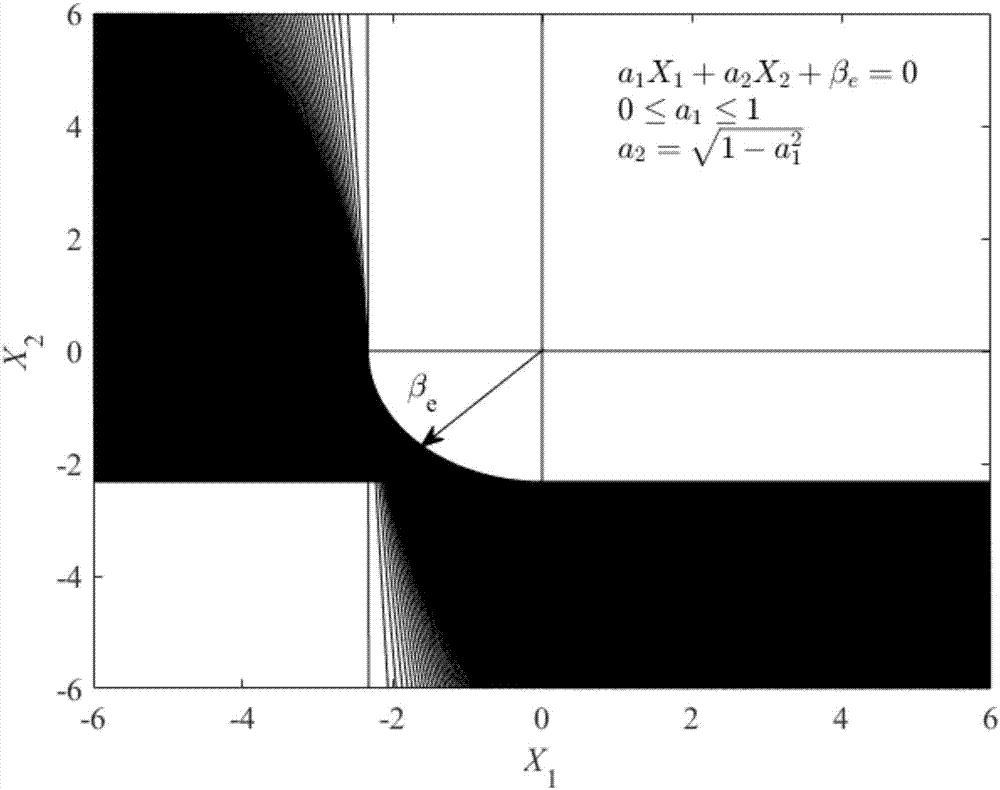
[0027] The system consists of 1000 failure modes in series, and the function function of the failure mode is Z=a 1 x 1 +a 2 x 2 +β e , where 0≤a 1 ≤1, Random variable X 1 、X 2 All obey the independent standard normal distribution, here take β e =2.326 can make the failure probability of each failure mode equal, about 0.01. figure 2 A schematic diagram of all functional functions in the standard normal space of Example 1 is drawn. Next, the technical solution proposed by the present invention will be used to evaluate the failure probability of the series structure system.

[0028] Step 1: Determine the input parameters, random variables and failure modes and their functional functions of the series structure system;

[0029] Step 2: Use Monte Carlo simulation to generate N groups of random samples that obey the given random variable distribution. For Example 1, N=10000 groups of two-dimensional independent standard normal distribution random samples can be generate...

Embodiment 2

[0047] The instability of any sliding surface in slope engineering will lead to the failure of the slope system, so the slope is usually regarded as a series system. Such as Figure 4 As shown, this embodiment is a single-layer soil slope, which is divided into 12659 sliding surfaces, each of which is a failure mode, and the soil slope can be regarded as a reliability problem of a series structure system containing 12659 failure modes.

[0048] The weight of the single-layer soil slope γ=20kN / m 3 , cohesion c and internal friction angle φ obey the logarithmic normal distribution, the average value is 10kPa, 30°, and the coefficient of variation is 0.3 and 0.2, respectively. Cohesion and internal friction angle were negatively correlated with a correlation coefficient of -0.7. In addition, the random field is used to simulate the spatial variability of soil parameters, the fluctuation ranges are 20m and 2m, respectively, and the correlation structure is exponential. The crit...

Embodiment 2

[0062] For embodiment 2, the equivalent sample analysis times of the Monte Carlo simulation method is N T,MCS =5000; and the equivalent sample analysis times required by the method of the present invention is N T,ADP =112×(1+5 000 / 12 659)=156, which is only 3.12% of the Monte Carlo simulation method.

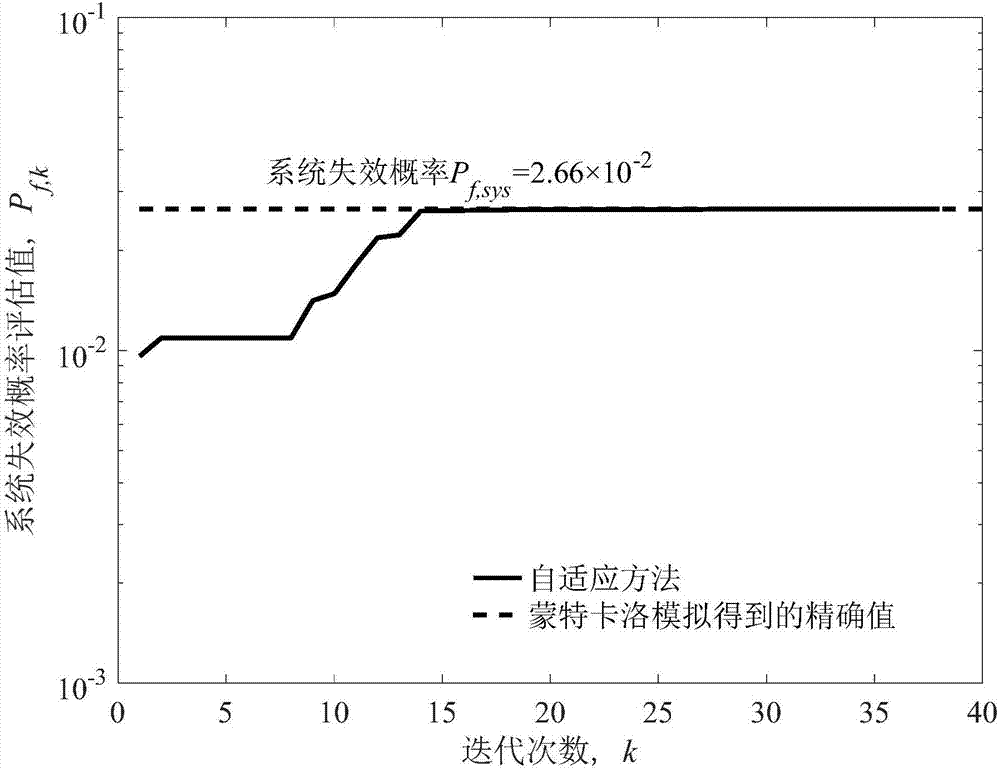
[0063] In order to further illustrate the convergence and calculation efficiency of the method proposed in the present invention, the method of the present invention was repeatedly executed 30 times for Example 2, and the obtained system reliability evaluation results are shown in Table 2.

[0064] Table 2 Example 2 System Reliability Evaluation Results

[0065]

[0066] The results in Table 2 show that the average system failure probability obtained by the method of the present invention through multiple simulations is 4.30×10 -3 , the system failure probability obtained by the Monte Carlo simulation method is 4.20×10 -3 , which are almost identical. The Unit COV of the ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

PropertyMeasurementUnit
Severeaaaaaaaaaa
Login to View More

Abstract

The invention discloses a self-adaption evaluation method for reliability of systems of cascaded structures. The method includes the steps that by means of iteration, Monte Carlo simulation analysis is conducted on each invalid pattern every time for recognizing an invalid sample, and a new invalid pattern is selected so that self-adaption evaluation of the reliability of the cascaded systems is achieved, and the calculation consumption can be effectively reduced. The method is simple in procedure, the reliability of the systems obtained after evaluation increases monotonously and is gradually convergent, the calculation consumption is less, and a new scheme is provided for the reliability evaluation of the systems of the cascaded engineering structures.

Description

technical field [0001] The invention belongs to the technical field of structure reliability evaluation, in particular to an adaptive evaluation method for the reliability of series structure systems. Background technique [0002] In actual engineering, structural failure is often a system reliability problem involving multiple failure modes. Structural failure can be divided into series system, parallel system and hybrid system according to the relationship between failure modes. A series system means that the failure of any failure mode in the system will lead to the failure of the whole system; a parallel system means that all the failure modes in the system are destroyed before the system as a whole fails; a hybrid system means that both series failure modes and parallel failure modes are included. system. It can be seen that the series structure system has the lowest redundancy and is more dangerous. There are many examples of series systems in actual engineering str...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06F17/50
CPCG06F30/20
Inventor 李典庆刘鑫曹子君唐小松
Owner WUHAN UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com