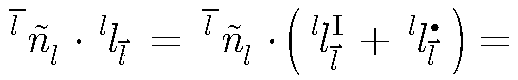Axis-invariant-based multi-axis robot inverse kinematics modeling and solving method

A multi-axis robot and inverse kinematics technology, applied in the field of robotics, can solve the problems of lack of design framework calculation and control methods

Active Publication Date: 2018-12-07
居鹤华
View PDF7 Cites 26 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0006] Therefore, although there are many robot-related theories, there is still a lack of a complete and effective design framework and corresponding calculation and control methods, which can solve all aspects of modeling in the actual development process of various robots. The operation structure and rules, to forward kinematics, inverse kinematics, and related issues of mechanical calculations

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Axis-invariant-based multi-axis robot inverse kinematics modeling and solving method
  • Axis-invariant-based multi-axis robot inverse kinematics modeling and solving method
  • Axis-invariant-based multi-axis robot inverse kinematics modeling and solving method

Examples

Experimental program
Comparison scheme
Effect test

example 22

[1146] Example 2.2 Given the axis sequence A = (i, c1, c2, c3, c4, c5, c], the parent axis sequence The axis type sequence is recorded as K=(F,R,R,R,P,P,P], and the joint coordinate sequence is recorded as q (i,c] =(φ c1 ,φ c2 ,φ c3 ,r c4 ,r c5 ,r c ]; so the kinematic chain is recorded as i l c =(i,c1,c2,c3,c4,c5,c]. And there is

[1147] i n c1 = c5 n c =1 [z] , c1 n c2 = c4 n c5 =1 [y] , c2 n c3 = c3 n c4 =1 [x] . (1.157)

[1148] This kinematic chain expresses: first perform the "3-2-1" rotation, and then perform the "1-2-3" translation. then there are

[1149]

[1150]

[1151]

[1152]

[1153] From formulas (1.157)~(1.160), we get

[1154]

[1155] Obviously,

[1156]

[1157] Therefore there is

[1158]

[1159] By formula (1.163) we get

[1160]

[1161] By formula (1.164) we get

[1162]

[1163] in:

[1164]

[1165] For precision electromechanical systems, orthogonal motion axes or measurement axes do n...

example 23

[1174] Example 2.3: The installation relationship of the camera system c relative to the patrol system r is determined by the angle between the coordinate axes of the two systems: in: represented by the axis x r to axis x c angle, and so on.

[1175] beg r Q c .

[1176] Solution: The projection of the camera coordinate axis x under the patrol system is The projection of the camera coordinate axis y under the patrol system is The projection of the camera coordinate axis z under the patrol system is Therefore there is

[1177]

[1178] Solved.

[1179]This example applies the direction cosine to compute the rotation transformation matrix, which is correct in principle. However, there is an important disadvantage in engineering: due to the errors in the nine angle measurements, the "orthonormality" constraint of the rotation transformation matrix is ​​broken. An example is as follows:

example 24

[1180] Example 2.4: Continuation of Example 2.3, measured by engineering

[1181]

[1182] calculated by the formula

[1183]

[1184] It can be seen from the calculation results r Q c Ill, with only 6 digits of precision.

[1185] Apply Equation (1.144) or Equation (1.146) to calculate the attitude angle It is theoretically established; the premise is: the rotation transformation matrix must satisfy the "orthonormality" constraint, when this constraint cannot be satisfied, The calculation error may be large. for sick Equations (1.144) and (1.146) are not fully utilized components, resulting in a sequence of attitude angles The accuracy is worse than the measurement accuracy of the cosine angle.

[1186] In addition to engineering measurement errors, the ill-conditioned rotation transformation matrix is ​​also caused by the existence of digital truncation errors in the computer. for kinematic chains k 1 j , because There is a certain morbidity that le...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to view more

PUM

No PUM Login to view more

Abstract

The invention provides an axis-invariant-based multi-axis robot inverse kinematics modeling and solving method. The method is implemented based on the fixed axis invariant D-H system and D-H parameterdetermination principle, the Ju-Gibbs quaternion and quasi direction cosine matrix principle, the axis invariant general 6R mechanical arm inverse solution principle and the axis invariant general 7Rmechanical arm inverse solution principle. The principles have the characteristics of being general, convenient and accurate and can be set into circuits and codes and be executed directly or indirectly and partially or totally in a multi-axis robot system. Besides, an analysis and verification system established based on the principles is also included and used for designing and verifying the multi-axis robot system.

Description

technical field [0001] The present invention relates to a robot, a robot autonomous control system and a method used in the robot autonomous control system, and in particular to a multi-axis robot, a multi-axis robot autonomous control system and a method used in the multi-axis robot autonomous control system. Background technique [0002] Robotics is a very hot field right now. Significant scientific and engineering manpower has been devoted to this field over the past few decades, and it has been studied for many years. However, once the number of axes and degrees of freedom increase to a certain number, according to the existing textbooks and the known observation, modeling, calculation and control methods, it often falls into complex and out-of-control, or even unsolvable problems. [0003] First, past practices lack the ability to generalize. For different robots, it is often necessary to re-study and establish corresponding kinematics and mechanical models. [0004]...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to view more

Application Information

Patent Timeline
no application Login to view more
Patent Type & Authority Applications(China)
IPC IPC(8): B25J9/16B25J9/00
CPCB25J9/0057B25J9/1607G05B2219/39077B25J9/1605B25J9/1602B25J9/1664B25J9/1697
Inventor 居鹤华
Owner 居鹤华
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Try Eureka
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap