Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Unconditionally stable seismic wave field continuation method based on staggered grid Lowrank decomposition

A staggered grid and wave field continuation technology, applied in the field of exploration geophysics, can solve problems such as time step stability constraints, and achieve the effect of suppressing numerical dispersion and improving computational efficiency

Inactive Publication Date: 2015-03-25
BC P INC CHINA NAT PETROLEUM CORP +1
View PDF0 Cites 17 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

Although the method is highly accurate, the choice of time step is still limited by the stability condition

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Unconditionally stable seismic wave field continuation method based on staggered grid Lowrank decomposition
  • Unconditionally stable seismic wave field continuation method based on staggered grid Lowrank decomposition
  • Unconditionally stable seismic wave field continuation method based on staggered grid Lowrank decomposition

Examples

Experimental program
Comparison scheme
Effect test

Embodiment Construction

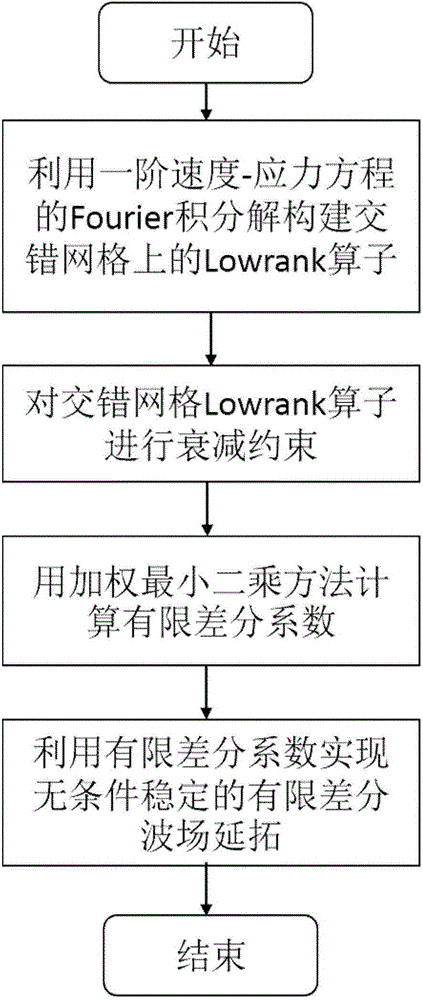
[0018] Such as figure 1 As shown, the unconditionally stable finite difference wavefield continuation method based on the staggered grid Lowrank operator is characterized in that it includes the following steps:

[0019] Step 1: Using the Fourier integral solution of the first-order velocity-stress equation to construct the Lowrank operator on the staggered grid

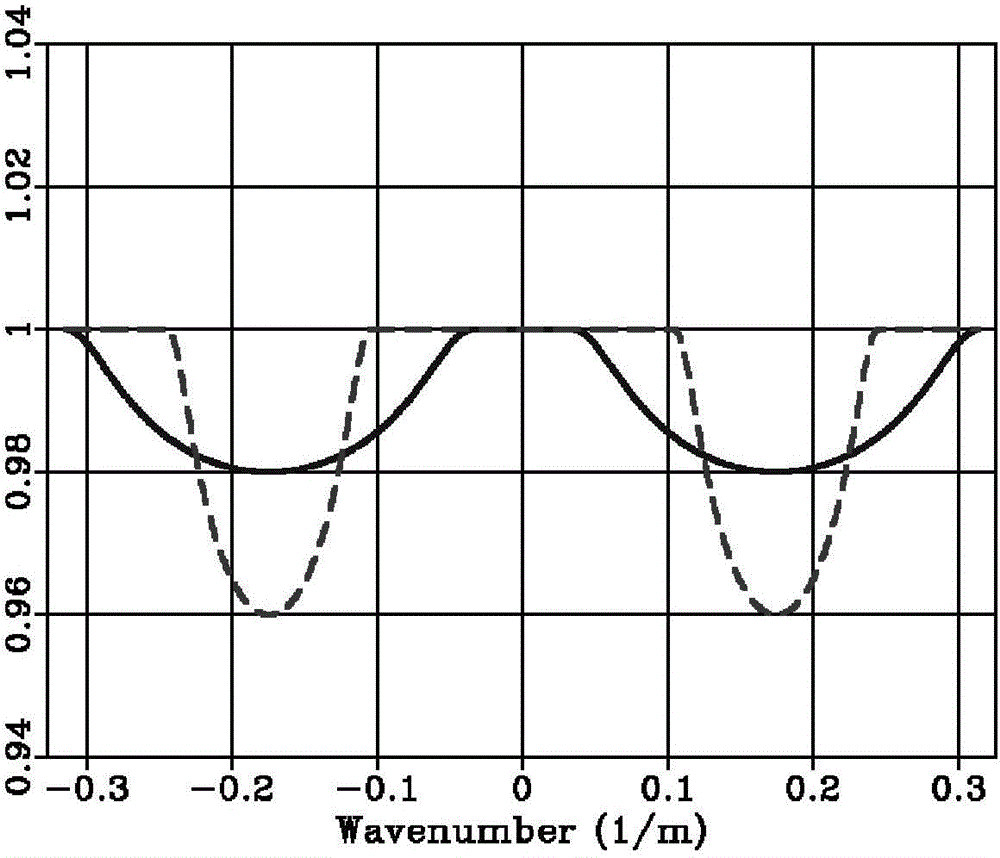
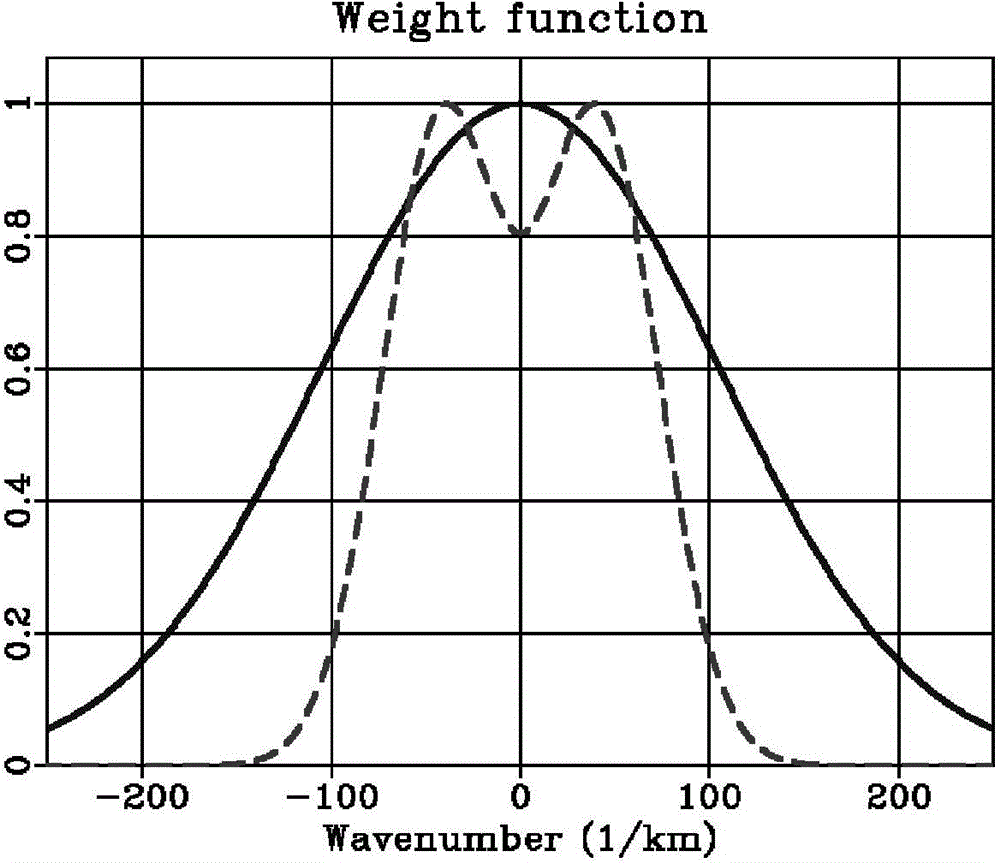
[0020] According to the velocity and density model of the underground medium and the wave field continuation parameters, the wave field continuation operator in the wave number-space domain is constructed on the staggered grid by using the Fourier integral solution of the first-order velocity-stress equation; The field continuation operator applies Lowrank decomposition to obtain the staggered grid Lowrank operator. The specific method is as follows:

[0021] The propagation of seismic wave field in underground medium can be approximated by the acoustic wave equation. There are many forms of acoustic wave equation...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention relates to an unconditionally stable finite difference seismic wave field continuation method based on a staggered grid Lowrank operator. The method comprises the steps that a first-order speed-stress equation Fourier integral solution is utilized for constructing the Lowrank operator on a staggered grid; an attenuation function is utilized for carrying out attenuation constraint on the staggered grid Lowrank operator; the weighted least square method is utilized for calculating a finite difference coefficient; the obtained finite difference coefficient is utilized for achieving unconditionally stable finite difference seismic wave field continuation. On the basis of the Lowrank decomposition approximate wave number-spatial domain operator, the computationally-stable and high-precision optimized finite difference coefficient is obtained through the attenuation constraint and the weighted least square method, and unconditionally stable finite difference seismic wave field continuation can be achieved through the constructed finite difference computational format.

Description

technical field [0001] The invention belongs to the field of exploration geophysics, and in particular relates to an unconditionally stable finite difference wave seismic field continuation method based on a staggered grid Lowrank operator. Background technique [0002] Oil and natural gas are important energy sources related to the national economy and people's livelihood. Seismic exploration is an effective method to find oil and gas resources and solve oil and gas exploration and development problems. Seismic forward modeling, seismic imaging and seismic inversion are important techniques in seismic exploration, and the computational efficiency and accuracy of these three techniques depend on the time-domain seismic wavefield continuation method adopted. The two most commonly used time-domain wavefield continuation methods include finite-difference methods and spectral methods. Finite difference uses difference instead of differential, which has the advantages of small ...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G01V1/28
Inventor 杜启振方刚
Owner BC P INC CHINA NAT PETROLEUM CORP
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com