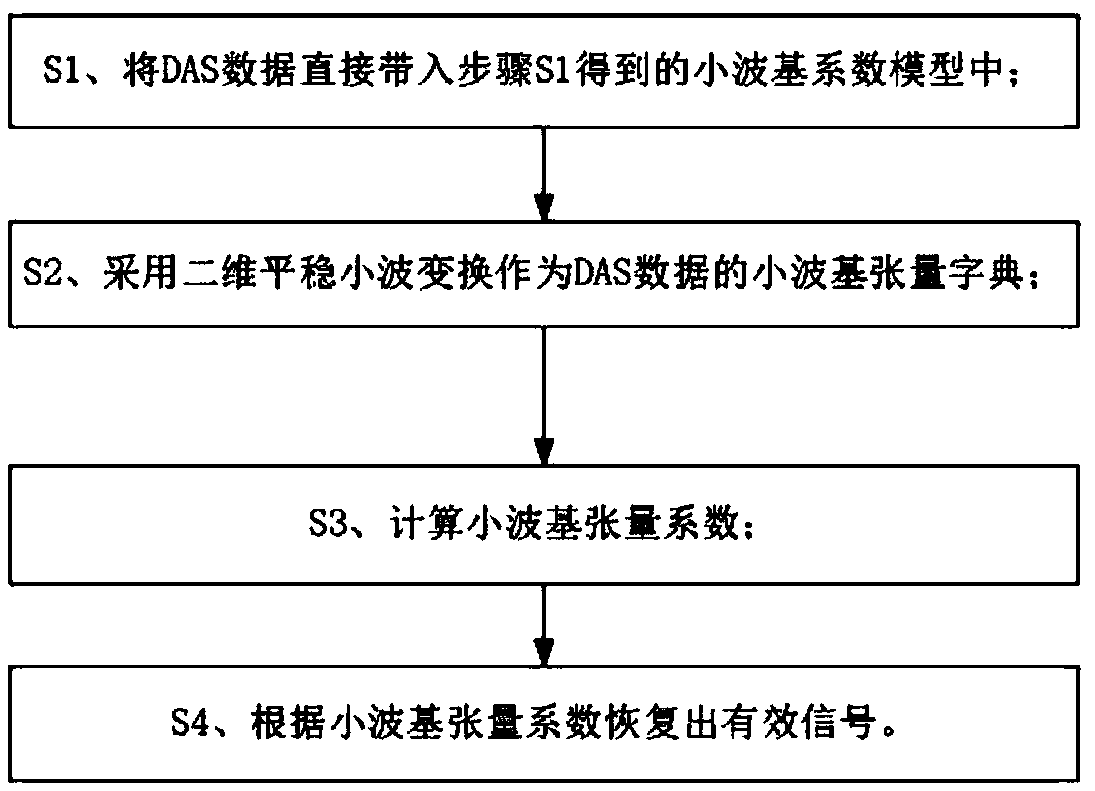
DAS data de-noising method based on wavelet base tensor sparse representation

A sparse representation, wave-based tensor technology, applied in impedance networks, adaptive networks, electrical components, etc., can solve problems such as complex geological structure, seismic interpretation errors, unsatisfactory denoising effect, etc., to reduce computational complexity , the effect of improving the operation speed

Active Publication Date: 2018-10-12
UNIV OF ELECTRONICS SCI & TECH OF CHINA
View PDF4 Cites 2 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

Although there are currently many optional models in theory, the selected model needs to be well matched with the noise distribution, otherwise an unsatisfactory denoising effect will be obtained
It is precisely because of the strict requirements of model selection that statistical denoising methods are not suitable for complex noise sources. Therefore, this method also has certain limitations in practical resea

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • DAS data de-noising method based on wavelet base tensor sparse representation
  • DAS data de-noising method based on wavelet base tensor sparse representation
  • DAS data de-noising method based on wavelet base tensor sparse representation

Examples

Experimental program
Comparison scheme
Effect test

Embodiment Construction

[0061] In order to facilitate those skilled in the art to understand the technical content of the present invention, the content of the present invention will be further explained below in conjunction with the accompanying drawings.

[0062] For ease of understanding content of the present invention, the present invention proposes following definition and inference:

[0063] The third-order tensor is expressed as The expression expanded along the third dimension is tensor The discrete Fourier transform of is expressed as tensor The transposition of and and And the superscript T stands for the transpose of the matrix.

[0064] For convenience, the tensor space and denoted respectively as and [k] represents the set {1,2,...,k}, tensor l 1 The norm and the Frobenius norm are denoted as and

[0065] Definition 1: The two tensors are and The tensor product of is a tensor and where * represents the circular convolution operator.

[0066] Corolla...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to view more

PUM

No PUM Login to view more

Abstract

The invention discloses a DAS data de-noising method based on wavelet base tensor sparse representation, which is applied to the field of seismic data processing and capable of effectively retaining structural information in DAS data and effectively removing noise. By proposing a new sparse representation model, the DAS data is expressed as a sparse tensor form. In the calculation process, an iterative compression threshold algorithm based on tensor product is used to calculate and reduce the computational complexity.

Description

technical field [0001] The invention belongs to the field of seismic data processing, in particular to a DAS data denoising technology. Background technique [0002] Distributed optical fiber sensing technology is a revolutionary new technology, which has been developed rapidly in the past two years. There are still few researches on the noise generated by optical fiber acquisition at home and abroad. In the field of signal processing, noise reduction technology has been relatively mature after long-term development. From the perspective of signal processing, the present invention divides noise into Gaussian noise and non-Gaussian noise according to types for processing. [0003] Gaussian noise is a common random noise whose n-dimensional probability density function obeys Gaussian distribution. Gaussian distribution, also known as normal distribution. For a random variable X, its probability density function is as follows figure 1 As shown, its distribution is denoted a...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to view more

Application Information

Patent Timeline
no application Login to view more
IPC IPC(8): H03H21/00
CPCH03H21/0043
Inventor 钱峰韩青云胡光岷
Owner UNIV OF ELECTRONICS SCI & TECH OF CHINA
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Try Eureka
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap