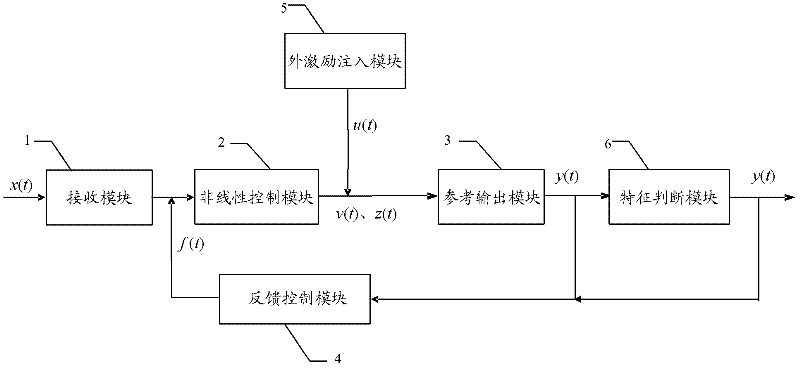
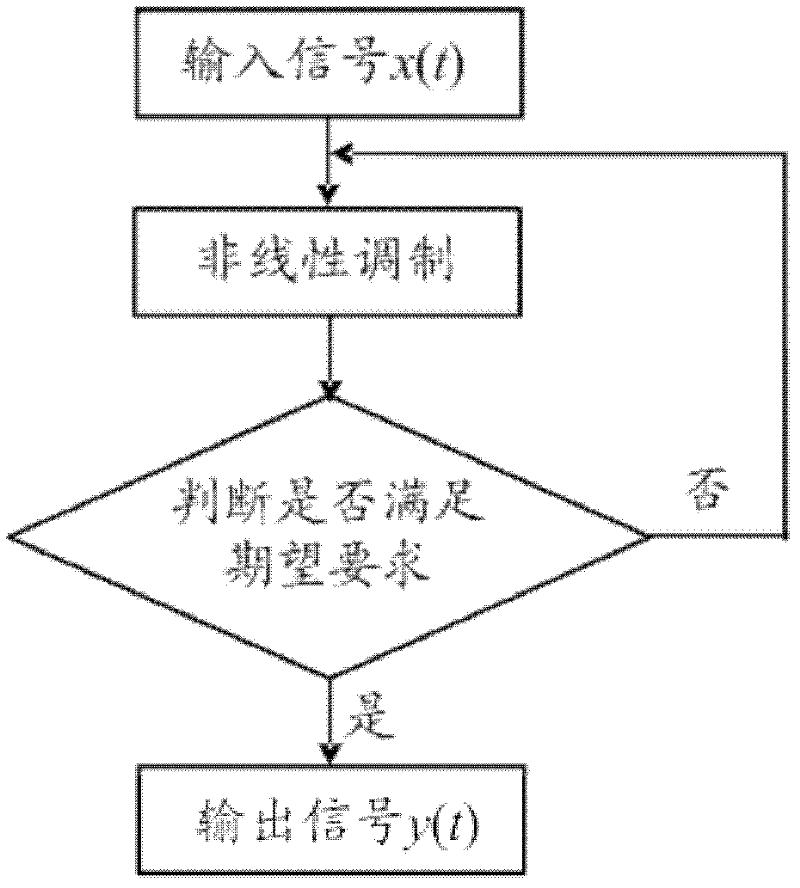
Low frequency broadening non-linear feedback control apparatus and method thereof

A nonlinear feedback and nonlinear control technology, applied in the direction of adaptive control, general control system, control/regulation system, etc., can solve problems such as instability, system structure instability, system performance change, etc.

Inactive Publication Date: 2012-03-28
HARBIN ENG UNIV
View PDF0 Cites 8 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0004] The feedback control system improves the system performance by introducing feedback, but also increases the complexity of the system and the number of components, but its unique advantag...

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Low frequency broadening non-linear feedback control apparatus and method thereof
  • Low frequency broadening non-linear feedback control apparatus and method thereof
  • Low frequency broadening non-linear feedback control apparatus and method thereof

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0071] The input signal frequency is 1kHz, and the output signal of the sinusoidal signal with the amplitude of 1 after the nonlinear control module 2 is simulated as follows, the control model is Simulation parameters: the sampling frequency is taken as 10 times the signal frequency, The power spectrum of the signal itself and the signal after cx 2 、dx 3 , The output power spectrum simulation results are shown in (a), (b), (c) and (d) of Figure 4. (2) Use the last 2000 points of the output signal to reconstruct the phase space, the embedding dimension is set to 2, and the delay time is set to 5, 10, 15 and 20 respectively. Then the phase diagrams of the nonlinear control module 2 are shown in (a), (b), (c) and (d) of FIG. 5 .

[0072] From Figure 4 and Figure 5, it can be concluded that the more orders the signal passes through the nonlinear control module 2, the more the output spectrum components are, and the spectrum shifts to the high frequency band. It can be see...

Embodiment 2

[0074] The block diagram of the control system is figure 1 As shown, there is no external excitation force acting on the system at this time Feedback control module 4 is output self-feedback process The input signal is a sinusoidal signal with a frequency of 1kHz and an amplitude of 1. The output signal after the nonlinear control module 2 is simulated as follows, the control model is Simulation parameters: embedding dimension time delay Take the last 2000 points for phase space reconstruction, the system output dynamics diagram is shown in Figure 6, and the time course diagram (a), power spectrum diagram (b) and phase diagram (c) of the feedback output signal are obtained by calculating the Lyapunov index Plot (d) of point i versus y(i).

[0075] From the time domain diagram and frequency spectrum diagram, after the signal is modulated, the amplitude and power spectrum value change greatly, and the phase diagram is still in a periodic state or a quasi-periodic stat...

Embodiment 3

[0077] The structural block diagram of the control system is shown in Figure 1, and the feedback control module 4 is an output self-feedback process At this time, there is an external high-frequency excitation source act on the system. The input signal is a sinusoidal signal sin(2πft) with a frequency of 1kHz and an amplitude of 1, which is input to the nonlinear control module 2, and the control model is Simulation Parameters: Embedding Dimensions time delay Take the last 2000 points for phase space reconstruction, The time course diagram (a) of the output signal after feedback, the power spectrum diagram (b), the phase diagram (c), and the diagram (d) of points i and Ly obtained by calculating the Lyapunov index. The dynamic states when the amplitude of the external high-frequency excitation source is 10 and the frequency is 50kHz are shown in Figure 7;

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to view more

PUM

No PUM Login to view more

Abstract

The invention provides a low frequency broadening non-linear feedback control apparatus and a method thereof. The apparatus is characterized in that: a receiving module receives an input signal and transmits the input signal to a non-linear control module; the non-linear control module carries out non-linear modulation on a signal input by the receiving module, transmits a modulated signal to a reference output module, and combined with a feedback signal output by a feedback control module, the non-linear control module carries out non-linear modulation and transmits a modulated signal to the reference output module; the reference output module computes difference between the modulated signal and a reference signal, and determines whether the modulated signal satisfies an expected requirement or not, if so, the modulated signal is output as an output signal, if not, the modulated signal is transmitted to the feedback control module. Through the non-linear control module and the feedback control module, the input signal output various orders of harmonic waves of a plurality of frequency components, amplitude is restricted, line spectrum is broadened, and under an effect of an appropriate parameter, reduction of input line spectrum energy is realized.

Description

technical field [0001] The invention relates to a closed-loop control device, in particular to a low-frequency widening non-linear feedback control device. The invention also relates to a nonlinear feedback control method for low-frequency broadening. Background technique [0002] The two most basic forms of control in control theory are open-loop control and closed-loop control. Each control form has its own characteristics. The open-loop controller and the controlled object only have a positive control effect. It is a memoryless system and has poor anti-interference ability. The closed-loop control system needs to continuously measure the feedback signal, and make a difference between the input and the feedback signal to judge whether the system output meets the expected requirements. If not, the difference is fed back to the controller, and the system output is continuously observed to obtain state information about the process. According to the design requirements, the...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to view more

Application Information

Patent Timeline
no application Login to view more
IPC IPC(8): G05B13/04
Inventor 杨德森兰朝凤时胜国李思纯
Owner HARBIN ENG UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Try Eureka
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap