Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

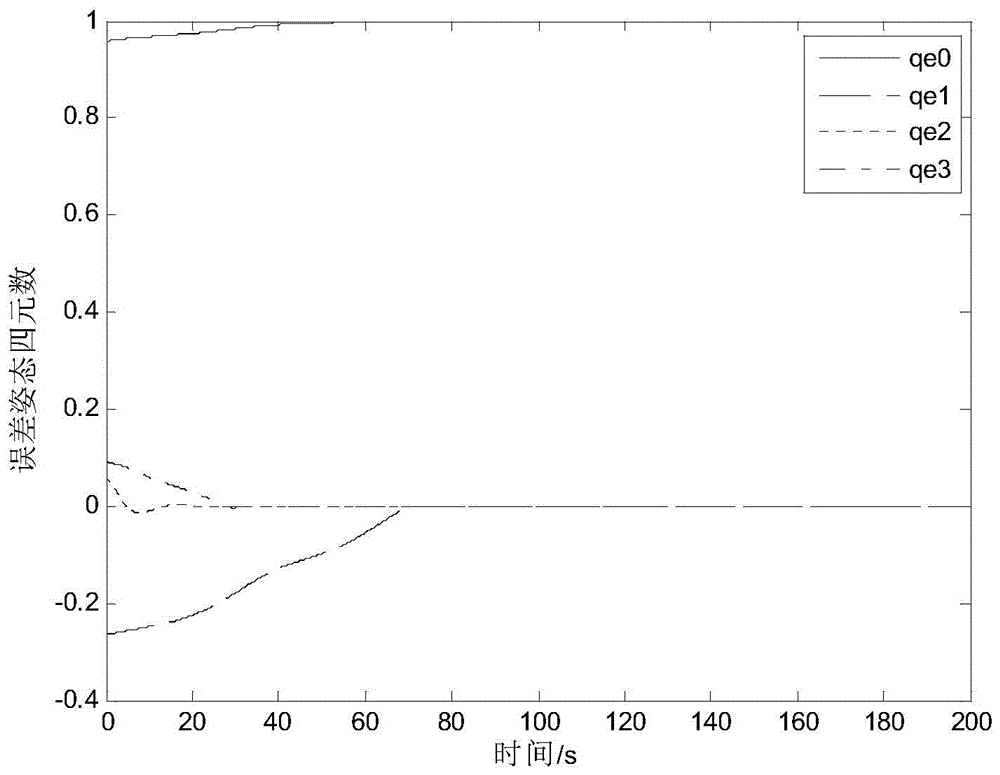
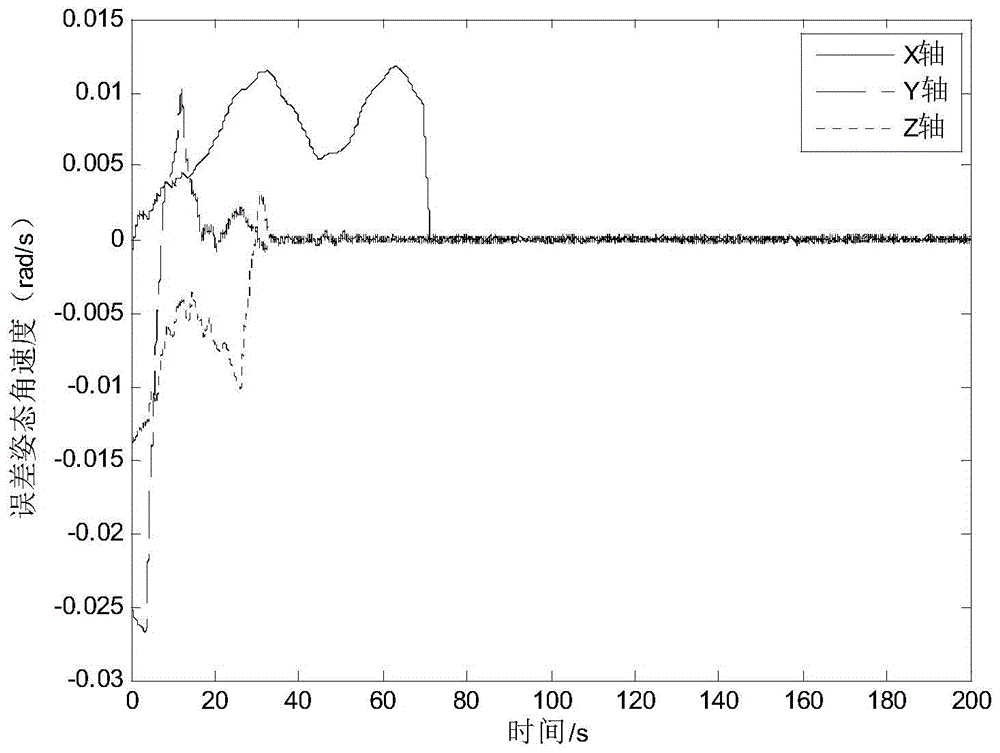
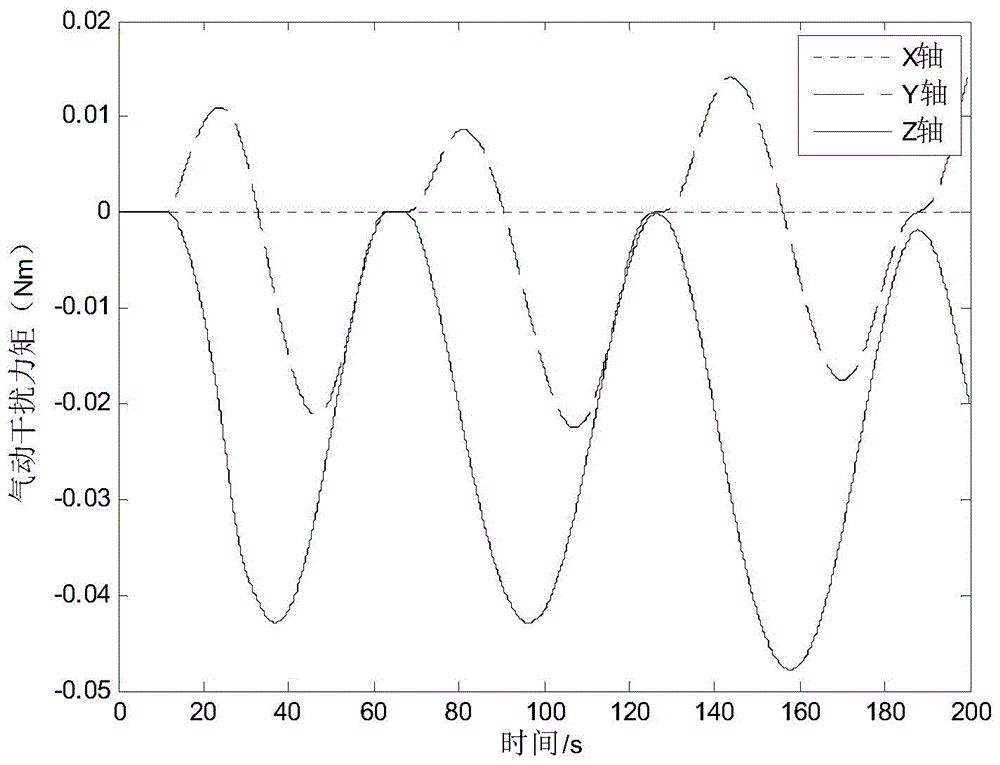
Attitude Tracking Control Method for Low Orbit Flexible Satellite Based on Sliding Mode Observer

A sliding mode observer and flexible satellite technology, applied in attitude control, adaptive control, general control system and other directions, can solve the problem of low accuracy of satellite attitude tracking control, and achieve improved accuracy, simplified control methods, and refined control. effect of effect

Active Publication Date: 2017-05-03
HARBIN INST OF TECH
View PDF3 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0003] The purpose of the present invention is to solve the problem of low satellite attitude tracking control accuracy caused by the large aerodynamic disturbance moment and the vibration of the flexible parts of the existing low-orbit flexible satellites, and propose a low-orbit low-orbit satellite based on a sliding mode observer. Attitude Tracking Control Method for Flexible Satellites

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Attitude Tracking Control Method for Low Orbit Flexible Satellite Based on Sliding Mode Observer
  • Attitude Tracking Control Method for Low Orbit Flexible Satellite Based on Sliding Mode Observer
  • Attitude Tracking Control Method for Low Orbit Flexible Satellite Based on Sliding Mode Observer

Examples

Experimental program
Comparison scheme
Effect test

specific Embodiment approach 1

[0036] The low-orbit flexible satellite attitude tracking control method based on the sliding mode observer of the present embodiment, the low-orbit flexible satellite attitude tracking control method is realized through the following steps:

[0037] Step 1: Establish the geocentric inertial coordinate system OX I Y I Z I and satellite body coordinate system OX b Y b Z b ;

[0038] Step 2: Define the modal vibration state Therefore, the attitude dynamic equation of the flexible satellite is rewritten as a state space expression:

[0039] In the formula, Represents the modal vibration velocity, δ represents the rigid-flexible coupling matrix, ω bI Indicates the current attitude angular velocity;

[0040] x is the system state quantity,

[0041] A represents the system matrix,

[0042] B represents the control input matrix,

[0043] y represents the measured output value of the state-space system,

[0044]H represents the measured output torque,

[0045] u repr...

specific Embodiment approach 2

[0057] The difference from the specific embodiment 1 is that the attitude tracking control method of the low-orbit flexible satellite based on the sliding mode observer in this embodiment, the earth-centered inertial coordinate system OX described in step 1 I Y I Z I , the coordinate origin of the geocentric inertial coordinate system is the earth's center of mass, OZ I axis along the direction of the earth's rotation axis pointing north, OX I The axis points to the direction of the vernal equinox, OY I Axis and OX I Shaft and OZ I The axes form a right-handed Cartesian coordinate system;

[0058] Satellite body coordinate system OX described in step 1 b Y b Z b , the coordinate origin of the satellite body coordinate system is the center of mass of the satellite, OX b Axis, OY b Shaft and OZ b The axis is fixedly connected with the star, and makes the OX of the coordinate axis b Axis, OY b Shaft and OZ b axis coincides with the satellite's main axis of inertia. ...

specific Embodiment approach 3

[0060] The difference from Embodiment 1 or Embodiment 2 is that the attitude tracking control method of the low-orbit flexible satellite based on the sliding mode observer in this embodiment, as described in step 2, rewrites the dynamic equation of the attitude of the flexible satellite into a state space expression: In the formula,

[0061] x is the state quantity of the system and x=(η T ψ T ω bI T ) T ,

[0062] η represents the modal vibration displacement,

[0063] A represents the system matrix and

[0064] B represents the control input matrix and

[0065] C represents the damping matrix of the modal vibration,

[0066] K represents the stiffness matrix of the modal vibration,

[0067] y represents the system measurement output value,

[0068] H represents the measurement output matrix. Since the observer only needs attitude angular velocity information to obtain the modal vibration, in order to facilitate the expression of the observer, then H=(0 0 I ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a low-orbit flexible satellite attitude tracking control method based on a sliding-mode observer, relates to a low-orbit flexible satellite attitude tracking control method based on a sliding-mode observer, and aims at solving the problems that an existing low-orbit flexible satellite is low in satellite attitude tracking control accuracy due to relatively large aerodynamic interference torque and vibration of flexile components. The low-orbit flexible satellite attitude tracking control method based on the sliding-mode observer comprises the following steps: building a geocentric inertial coordinate system and a satellite body coordinate system; building a state space expression, and determining an upper bound of an interference signal received by the observer; solving a gain matrix of the observer, a matching matrix of the observer and a Lyapunov equation matrix variable; observing to obtain an estimated mode vibration velocity value of the sliding-mode observer and an estimated mode vibration state value of the sliding-mode observer; rewriting a kinetic equation of a flexible satellite attitude into an error attitude tracking control model; determining a sliding-mode term gain of the control law, and carrying out tracking control on the error attitude tracking model by adopting the siding-mode control law according to measured satellite attitude quaternion, attitude angular velocity information and estimated mode quantity value. The low-orbit flexible satellite attitude tracking control method is applied to satellite attitude tracking control.

Description

technical field [0001] The invention relates to a low-orbit flexible satellite attitude tracking control method based on a sliding mode observer. Background technique [0002] With the development of satellite attitude control technology, the requirements for satellite attitude control accuracy are getting higher and higher, especially for satellites with optical cameras as payloads, the payload has extremely high requirements for ground pointing accuracy. For this type of satellites, in order to improve the accuracy of optical imaging, they often choose low-orbit operation. Compared with mid-orbit satellites, low-orbit satellites are more affected by the aerodynamic moment, and the aerodynamic moment varies with the satellite attitude. The change will have a great impact on the accuracy of satellite attitude control. Therefore, the influence of aerodynamic disturbance torque must be considered when designing a high-precision satellite attitude control method. In addition, ...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Patents(China)
IPC IPC(8): G05D1/08G05B13/04
Inventor 易涛陈雪芹耿云海曾奎李冬柏王峰张刚叶东
Owner HARBIN INST OF TECH
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com