Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Non-overshot fractional order time-varying sliding mode control method

A control method and fractional-order technology, applied in the field of control, can solve problems such as not attracting extensive attention in the engineering community, and the integer order model cannot well describe the dynamic behavior of viscoelasticity and diffusive systems.

Inactive Publication Date: 2015-12-23
BEIJING INSTITUTE OF TECHNOLOGYGY
View PDF3 Cites 19 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

However, due to the lack of physical background at that time, "fractional order" has been studied as a purely theoretical problem in the field of mathematics for a long time, and has not attracted widespread attention from the engineering community.
In recent years, it has been found that integer-order models cannot well describe the dynamic behavior of some systems with viscoelasticity, diffusion, etc.

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Non-overshot fractional order time-varying sliding mode control method
  • Non-overshot fractional order time-varying sliding mode control method
  • Non-overshot fractional order time-varying sliding mode control method

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0062] Consider the system:

[0063] D 2 x=u+du

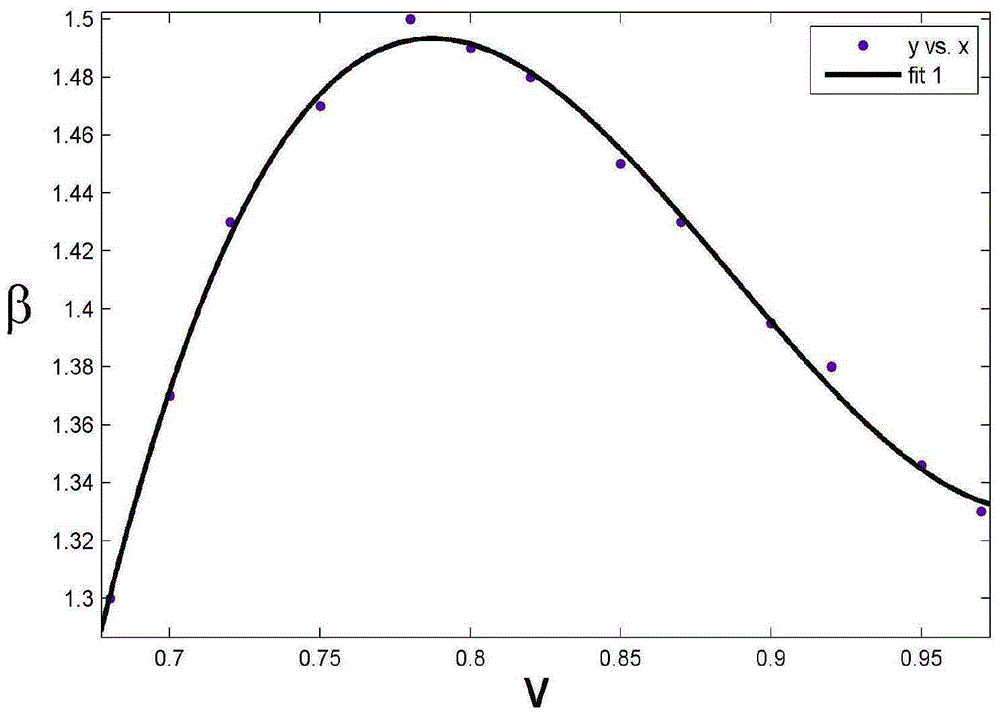
[0064] Let the sliding mode surface coefficient n=2, v=0.8, the controller parameter η=50000, the initial state of the system is x=0, and the tracking command of the system is x d =1, the disturbance parameter of the controlled object is du=2*cos(5t)+sin(2.5t). Time-varying terms A=-1, B=10.

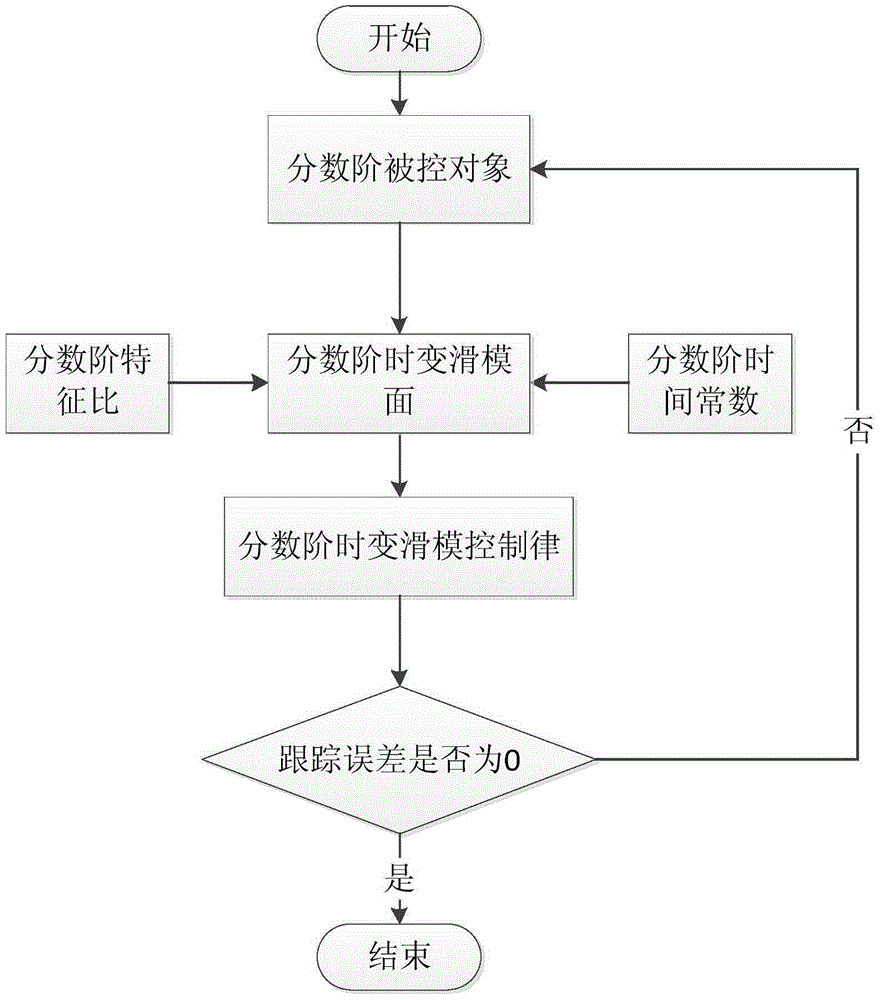
[0065] The present embodiment discloses a fractional time-varying sliding mode control method without overshoot, which specifically includes the following steps:

[0066] Step 1. Establish a dynamic model of the fractional-order uncertain system.

[0067] D 2 x=f(x)+g(x)u+du(1)

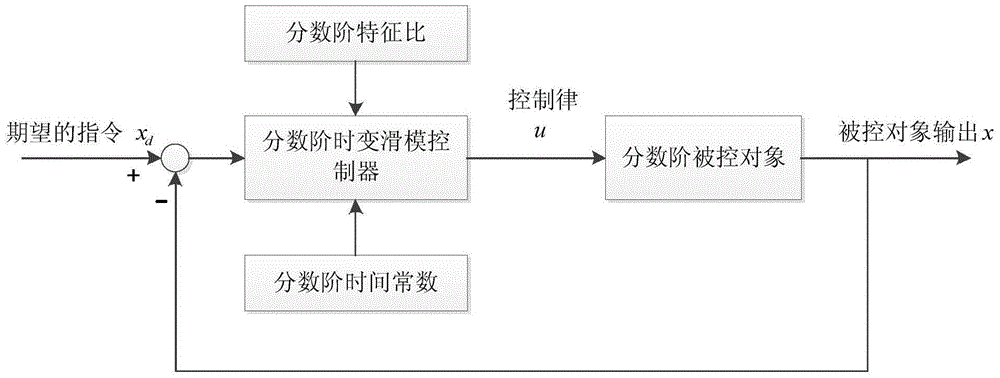
[0068] In the formula, x is the state of the system, f(x) and g(x)≠0 are smooth nonlinear functions about x, du is the external aggregate interference that the system receives, and the external aggregate interference is bounded |du|≤du max . D is the differential operator. u is the system control input. The reference trajectory of the system is x d .

[0069] Step 2. Des...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The present invention discloses a non-overshot fractional order time-varying sliding mode control method, relating to a time-varying sliding mode control method, belonging to the technical field of control. The method comprises a step of establishing the dynamic model of a fractional order uncertain system, a step of designing a fractional order time-varying sliding mode control law such that the system responds rapidly and has no overshot, a step of taking the control amount u obtained in the step (2) as a command and inputting the command into the dynamic model of the fractional order uncertain system to control the dynamic model, a step of taking a system state as the input of a fractional order time-varying sliding mode controller, and repeating the step (1) and the step (2) such that a tracking error converges to a zero value. According to the method, the characteristic ratio configuration method of an integer order system is promoted to the fractional order system, the system response non overshot is realized through determining the characteristic ratio, a system state is kept on a sliding mode surface from an initial time, and the robustness of the system is enhanced. In addition, the response rate of the system can be changed and the overshot amount of the system is not changed through individually adjusting a time constant.

Description

Technical field [0001] The invention relates to a time-varying sliding mode control method, in particular to a fractional-order time-varying sliding mode control method without overshoot, belonging to the technical field of control. Background technique [0002] Sliding mode control has the characteristics of global convergence, easy implementation, strong robustness to external disturbances, and insensitive to parameter changes and model errors, which makes it widely used in nonlinear control. Sliding mode control makes the system state converge to the equilibrium point along the sliding mode surface through the output of the controller. The control process can be divided into reach section and sliding section. The arrival section has poor robustness, and the sliding section has high frequency chattering. These are two major shortcomings of sliding mode control. Sliding mode control is widely used in various nonlinear systems: motor servo control, aircraft attitude control, e...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G05B13/04
Inventor 盛永智薛晨琛刘向东
Owner BEIJING INSTITUTE OF TECHNOLOGYGY
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com