Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

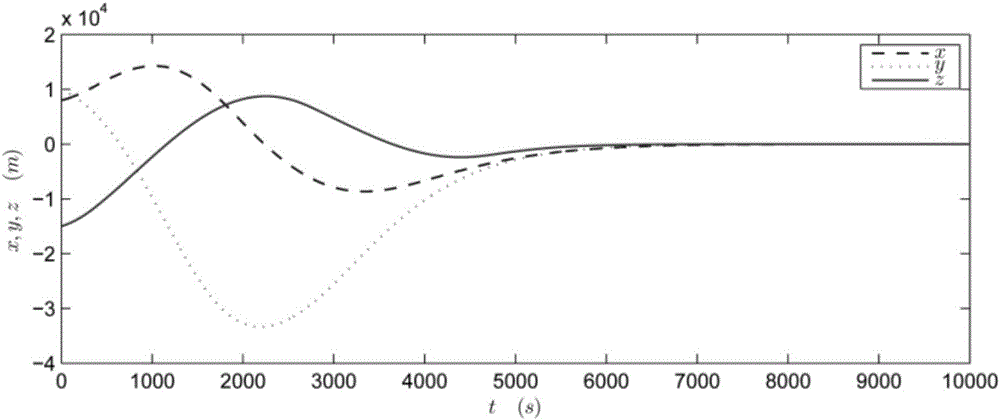
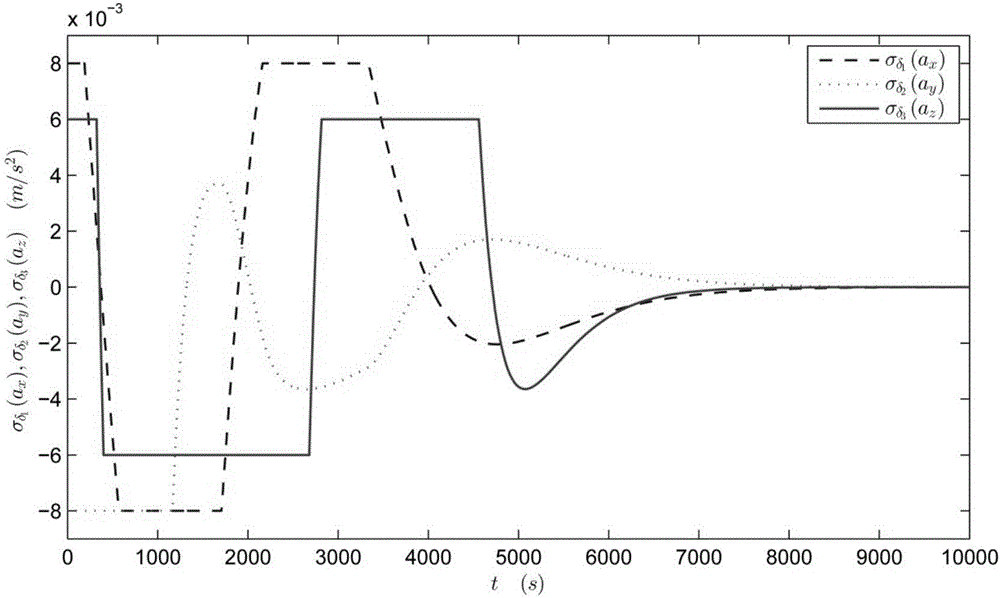
Linear-feedback global stabilization method for controlling limited spacecraft rendezvous control system

A technology of control system and linear feedback, which is applied in the direction of instruments, special data processing applications, electrical digital data processing, etc., can solve the problems of linear control law without relevant achievement reports, complex control law implementation, difficult to debug, etc., to achieve convenient design and Realization, fast convergence speed, and strong robustness

Active Publication Date: 2017-02-15
黑龙江省工研院资产经营管理有限公司
View PDF3 Cites 13 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

The control laws designed using these existing control methods generally have two forms: one is nonlinear control law, its disadvantage is that the realization of the control law is more complicated and difficult to debug; the other is linear control law, its disadvantage is that it can only guarantee the closed-loop system. Local stability (partial linear control law can achieve semi-global stabilization)
Up to now, there is no relevant achievement report on the linear control law to achieve global stabilization

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Linear-feedback global stabilization method for controlling limited spacecraft rendezvous control system
  • Linear-feedback global stabilization method for controlling limited spacecraft rendezvous control system
  • Linear-feedback global stabilization method for controlling limited spacecraft rendezvous control system

Examples

Experimental program
Comparison scheme
Effect test

specific Embodiment approach 1

[0025] Specific implementation mode one: a linear feedback global stabilization method for a control-constrained spacecraft rendezvous control system includes the following steps:

[0026] Step 1: Introduce the target spacecraft orbital coordinate system o-xyz, its origin o is located at the center of mass of the target spacecraft, the x-axis is along the direction of the radius of the circular orbit, the y-axis is along the flight direction of the tracking spacecraft, and the z-axis points to the orbital plane The outer and x-axis and y-axis form a right-handed coordinate system; based on the linearization model of the spacecraft rendezvous system in the x-y plane described by the C-W equation, when the thrusters in the x and y-axis directions are both working, a linear system for global stabilization is established. Feedback control law:

[0027] u=FX(1)

[0028]

[0029] in is the state vector, where x and are the position and velocity in the x-axis direction, y and...

specific Embodiment approach 2

[0037] Specific embodiment two: the difference between this embodiment and specific embodiment one is: the specific process of establishing a linear feedback control law to realize global stabilization in the step one is:

[0038] Step 11: Establishment of the system model;

[0039] The linear C-W equation is used as the mathematical model of the spacecraft rendezvous control system, and its specific form is:

[0040]

[0041] where a x ,a y ,a z are the accelerations on the x, y, and z coordinate axes of the thrusters installed on the catching-up spacecraft, respectively, is a saturation function;

[0042] σ δ (·): R→[-δ,δ] is a standard saturation function, the specific form is

[0043]

[0044] In order to simplify the description, let σ(x) represent σ 1 (x).

[0045] The spacecraft rendezvous process is described as a state vector From a non-zero initial value φ(t 0 ) transition to state φ(t f )=0 process, t 0 Indicates the start time of the rendezvous ...

specific Embodiment approach 3

[0097] Specific embodiment three: the difference between this embodiment and one of the specific embodiments one to two is: the specific process of establishing the conditions to ensure that the closed-loop system has the fastest convergence rate in the step two is:

[0098] Since the closed-loop systems (1) and (6) (or (12)) are globally asymptotically stable, the system will work in the linear region after a finite time and become a linear system. At this time, the convergence speed of the system depends on the position of the pole set λ(A+BF) of the system:

[0099] λ(A+BF)=λ(nA 0 +nB 0 f 0 ) = nλ(A 0 +B 0 f 0 ) (26)

[0100] where λ(A 0 +B 0 f 0 ) has nothing to do with the angular velocity n, so that the closed-loop system has the fastest convergence speed, and the feedback gain F 0 is the optimal solution to the following extremum problem:

[0101]

[0102] λ(A 0 +B 0 f 0 ) is related to μ; even when μ is given, the above extremum problem is a nonlinear ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention provides a linear-feedback global stabilization method for controlling a limited spacecraft rendezvous control system and relates to a controller design method of the spacecraft rendezvous control system. A thruster arranged on a chasing spacecraft can only provide finite thrust, so that the system must face the problem of control limitation. The control quality is reduced or even the system instability is generated if the problem is ignored. Aiming at the defect of the prior art, a linear state feedback-based global stabilization control law is provided and an optimal selection scheme of control law parameters is given, so that the condition that a closed-loop system has a fastest convergence speed is ensured. The linear-feedback global stabilization method has the advantages that: firstly, the provided control law is linear and is convenient to design and implement; secondly, the global asymptotic stability of the closed-loop system is ensured; and finally the optimal parameters are given to ensure that the closed-loop system has the fastest convergence speed. The linear-feedback global stabilization method is used for the field of spacecraft rendezvous control.

Description

technical field [0001] The invention relates to a controller design method of a spacecraft rendezvous control system. Background technique [0002] Spacecraft rendezvous and docking is the basis for realizing spacecraft maintenance, interception, port entry, large-scale assembly of multiple spacecraft, multi-satellite network collaboration, and astronaut rescue and other related space missions. The success of spacecraft rendezvous and docking directly affects the realization of the above tasks. The controller design for spacecraft rendezvous system has important engineering significance. [0003] Since the thrusters installed on the pursuit aircraft can only provide limited thrust, only limited control acceleration can be produced. If the controller is not designed with this problem in mind, the acceleration that the thruster needs to produce may be greater than the maximum acceleration that the thruster itself can provide. At this time, the actual closed-loop system will...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G06F17/50
CPCG06F30/15G06F30/367
Inventor 周彬姜怀远
Owner 黑龙江省工研院资产经营管理有限公司
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com