Method for improving precision of carbonatite dynamic and static Young modulus conversion model

A technology of static Young's modulus and Young's modulus, which can be used in measuring devices, geographic modeling, geophysical measurement, etc., and can solve the problems of loss of engineering guidance, low accuracy, and lack of dynamic and static Young's modulus conversion Model accuracy and other issues, to achieve the effect of ensuring feasibility and convenience, and good correction effect

Active Publication Date: 2020-09-15
CHINA UNIV OF PETROLEUM (EAST CHINA)
View PDF3 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

For rock formations with good physical properties and low anisotropy, a dynamic and static Young's modulus conversion model with high accuracy can be fitted through experiments. For rock formations with strong anisotropy and complex pore structure, the dynamic and static Young's modulus conversion model obtained through indoor experiment fitting is usually not very accurate, and has lost its guiding significance for actual engineering
[0010] Carbonatite reservoirs are c

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Method for improving precision of carbonatite dynamic and static Young modulus conversion model
  • Method for improving precision of carbonatite dynamic and static Young modulus conversion model
  • Method for improving precision of carbonatite dynamic and static Young modulus conversion model

Examples

Experimental program
Comparison scheme
Effect test

Example Embodiment

[0033] The specific embodiments of the present invention will be further described below in conjunction with the accompanying drawings and an example well in a certain carbonate block:

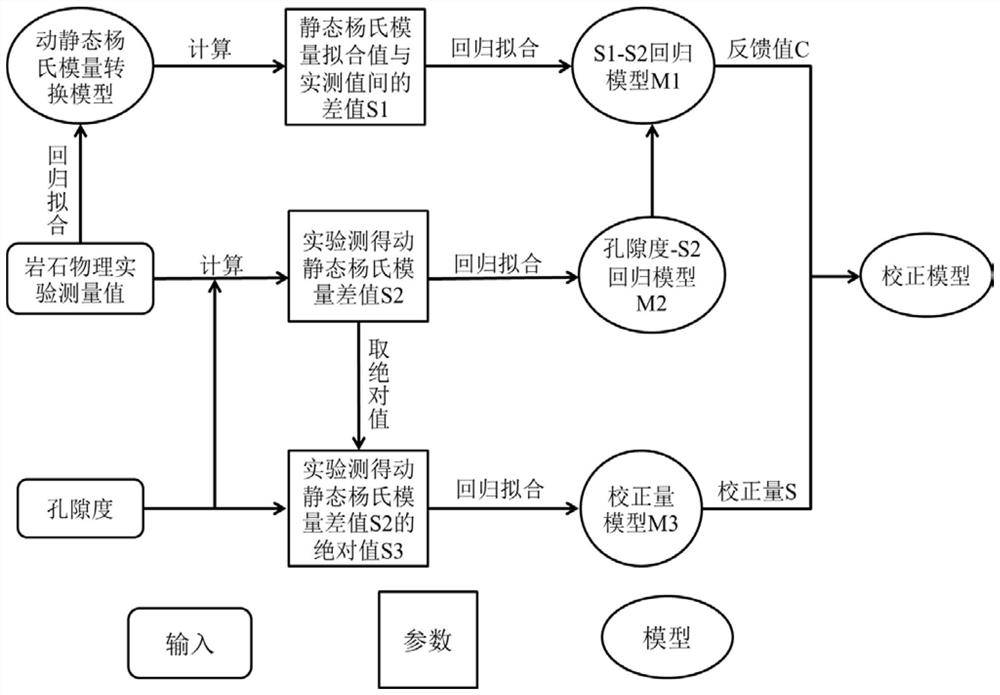
[0034] figure 1 Shown is a flow chart of a method for improving the accuracy of the dynamic and static Young's modulus conversion model of carbonate rock, which specifically includes the following steps:

[0035] Step 1, establish a dynamic and static Young's modulus conversion model;

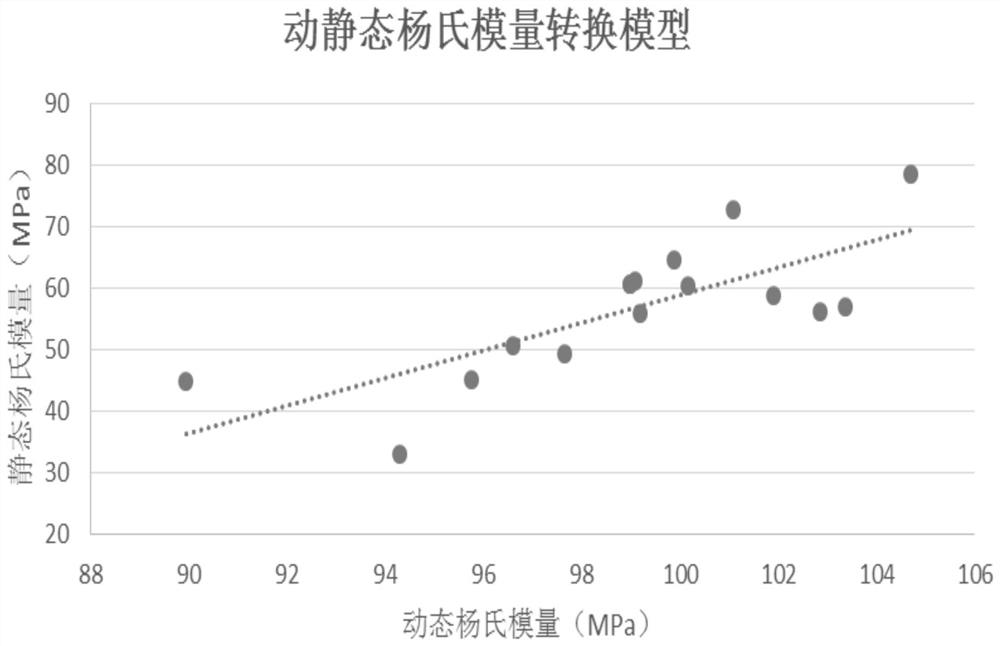
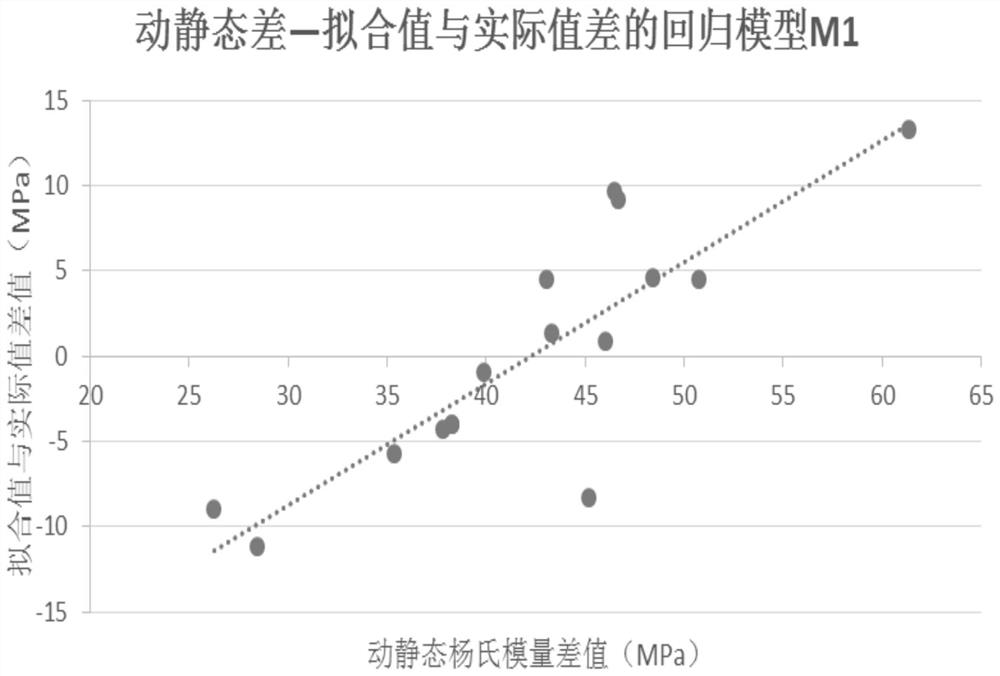
[0036] In this example, 16 standard-sized cores from a sample well in a certain carbonate block are used for petrophysical experiments, and the core size is 250 mm. The porosity, experimental dynamic Young's modulus and The experimental static Young's modulus, the measurement results are shown in Table 1, the regression analysis software was used to linearly fit the experimental dynamic Young's modulus and the experimental static Young's modulus of the carbonate rock sample, and the dynamic and static Young's ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to view more

PUM

No PUM Login to view more

Abstract

The invention discloses a method for improving the precision of a carbonatite dynamic and static Young modulus conversion model, and particularly relates to the field of carbonatite reservoir logging.The method comprises the following steps: 1, establishing a dynamic and static Young modulus conversion model according to rock physical experiment data; 2, establishing a regression model M1 of an experimental dynamic and static Young modulus difference value S2--a static Young modulus fitting value and an experimental value difference value S1 by utilizing the dynamic and static Young modulus conversion model and rock physical experimental data; 3, establishing a regression model M2 of the experimental dynamic and static Young modulus difference S2 -- porosity according to the rock physicalexperimental data; 4, establishing a correction value model M3; and 5, calculating the fitting static Young modulus of the point to be measured, and correcting by using the regression model M1, the regression model M2 and the correction value model M3 to obtain the corrected static Young modulus. According to the method, the precision of the carbonatite dynamic and static Young modulus conversionmodel is improved, and well logging evaluation and oil field development of the carbonatite reservoir are facilitated.

Description

technical field [0001] The invention relates to the field of well logging of carbonatite reservoirs, in particular to a method for improving the precision of a dynamic and static Young's modulus conversion model of carbonatite. Background technique [0002] In the process of reservoir evaluation and oilfield development, the determination of parameters such as Young's modulus and Poisson's ratio in the target block is very important. If there is a large error in the measured value, it will directly affect the engineering design, construction, reservoir fracturing, etc. successfully launch. [0003] According to different test methods and principles, Young's modulus is divided into dynamic Young's modulus and static Young's modulus; dynamic Young's modulus refers to the Young's modulus calculated by measuring the time difference of longitudinal and transverse waves in rock mass based on elastic theory Quantity, dynamic Young's modulus E d As follows: [0004] [0005] I...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to view more

Application Information

Patent Timeline
no application Login to view more
IPC IPC(8): G06F30/20G06F17/18G01V99/00
CPCG06F30/20G06F17/18G01V99/005
Inventor 张冉孙天威闫伟超张鹏云
Owner CHINA UNIV OF PETROLEUM (EAST CHINA)
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Try Eureka
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap