Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Sparsity-adaptive irregular seismic data reconstruction method and system

A technology of seismic data and sparsity, applied in the field of seismic exploration, can solve the problem of low reconstruction accuracy of seismic data, and achieve the effect of solving the problem of low reconstruction accuracy

Pending Publication Date: 2022-03-04
INST OF GEOLOGY & GEOPHYSICS CHINESE ACAD OF SCI
View PDF4 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0004] The object of the present invention is to provide a method and system for reconstructing irregular seismic data with self-adaptive sparsity. Based on the Pareto curve combined with the secant method, the sparsity information of seismic data can be searched, which effectively solves the problems caused by the current unknown sparsity. The problem of low reconstruction accuracy of seismic data

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Sparsity-adaptive irregular seismic data reconstruction method and system
  • Sparsity-adaptive irregular seismic data reconstruction method and system
  • Sparsity-adaptive irregular seismic data reconstruction method and system

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0050] Such as figure 1 As shown, the present embodiment provides a sparsely adaptive non-standard seismic data reconstruction method, the method comprising:

[0051] S1, get a perceived matrix, original seismic data, and noise level.

[0052] The seismic data acquisition process based on the compressed perception can generally be expressed as y = aθ, where Y is the sparse seismic wave field data collected, a ∈R M×N In order to perceive the matrix and m << n, θ is a sparse coefficient of the complete seismic wave field in the conversion domain.

[0053] S2, initialization of the first iterative sparseness and the first iterative sparse coefficient. That is, the first iterative sparseness is set to 0, and the first iterative sparse coefficient is set to zero vector.

[0054] S3, according to the sensing matrix, the first iterative sparse coefficient and the original seismic data calculates an error function corresponding to the first iterative sparse coefficient: f (θ 1 ) = || aθ 1...

Embodiment 2

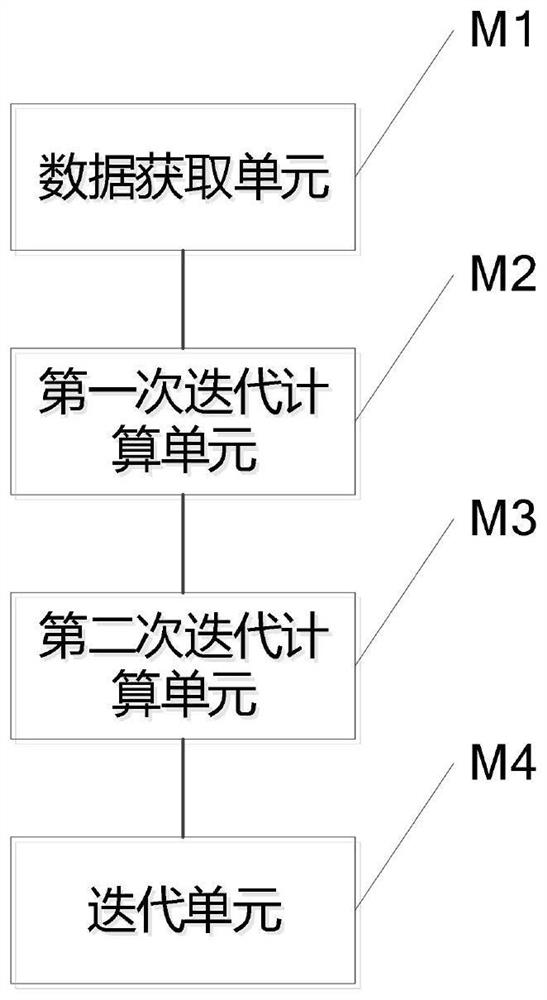
[0089] Such as image 3 As shown, this embodiment provides a sparse-adaptive non-standard seismic data reconstruction system including:

[0090]The data acquisition unit M1 is used to obtain a perceived matrix, the original seismic data, and the noise level;

[0091] The first iteration calculation unit M2 is used for:

[0092] Initialize the first iterative sparseness and the sparse coefficient of the first iteration;

[0093] According to the sensing matrix, the first iterative sparse coefficient and the original seismic data calculate an error function corresponding to the first iterative sparse coefficient;

[0094] The second iterative calculation unit M3 is used for:

[0095] Set the second iterative sparseness to: k 2 = Max (0.001n, 1); where n represents the size of the second dimension of the perceived matrix;

[0096] Using the hard threshold tracking algorithm to solve the second iteration, the constraint function is || θ 2 || 0 ≤ K 2 Where θ 2 Represents the scribble co...

Embodiment 3

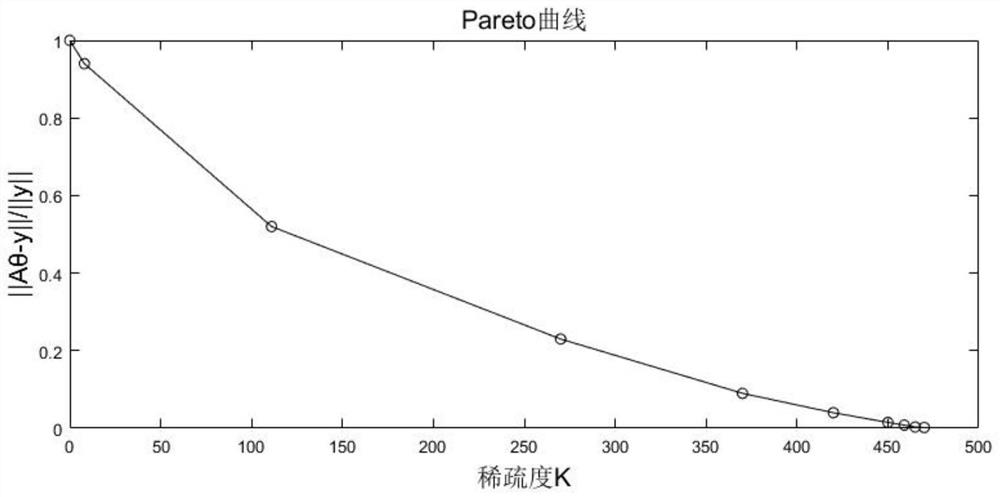
[0112] A simple layered speed model is designed in this embodiment, such as Figure 4 As shown, the speed model is positive and the simulation is performed, and the interval of 400 markings, the road spacing is 4m, the time sampling interval 4ms, total acquisition time 1.2s, and the simulated single cannon earthquake record Figure 5 Distance Figure 5 Exemplary shows the original single cannon seismic record. See Image 6 , Image 6 An exemplary showed a single cannon seismic record after randomly extracting 70% seismic road, in this example Figure 5 The single cannon seismic records shown were randomly exhausted and obtained 70% of the single cannon seismic records. See Figure 7 , Figure 7 An exemplary showed a single cannon seismic record after the data reconstruction in the present embodiment, and in the present embodiment Image 6 The sparse single cannon seismic record is reconstructed by seismic data reconstruction using sparse-adaptive compressed sensing seismic data reconstruct...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention relates to a sparseness-adaptive irregular seismic data reconstruction method and system, and the method comprises the steps: firstly, automatically tracking the sparseness information of seismic data based on a Pareto curve in combination with a secant method, and obtaining a better reconstruction result compared with a previous reconstruction algorithm for the irregular seismic data with unknown sparseness; secondly, solving an optimal sparse coefficient by using a hard threshold tracking algorithm under the current sparseness, and solving a minimization problem by using a Nesterov accelerated gradient descent algorithm which has higher efficiency compared with a least square algorithm and a common gradient descent algorithm aiming at a large-scale seismic data reconstruction problem; and finally, inverse sparse transformation is carried out according to the sparse coefficient obtained by the optimal sparseness to obtain reconstructed seismic wave field data. According to the method, on the premise that the sparseness of the seismic data is unknown, the seismic data reconstruction can be quickly and accurately realized through an efficient reconstruction algorithm.

Description

Technical field [0001] The present invention relates to the field of seismic exploration techniques, in particular to a sparse-adaptive non-standard seismic data reconstruction method and system. Background technique [0002] During existing compressed sensing reconstruction, most signal reconstruction algorithms require the sparseness of the signal, so, before the reconstruction signal, the coefficient of the sparse signal itself or the compressible signal is subjected to a sparseness. Metrics, estimated sparse dense or too small will seriously affect the reconstruction accuracy of the reconstruction algorithm. For seismic data, it does not know if the degree of sparse in the transform domain, and whether the size of the sparseness accurately affects the reconstruction accuracy of seismic data. [0003] Therefore, it is urgent in the art to have a sparse-adaptive non-standard seismic data reconstruction scheme. Inventive content [0004] It is an object of the present invention...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G01V1/30G01V1/36G01V1/28
CPCG01V1/307G01V1/36G01V1/28
Inventor 穆盛强霍守东舒国旭黄亮周旭晖邹佳儒
Owner INST OF GEOLOGY & GEOPHYSICS CHINESE ACAD OF SCI
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com