Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

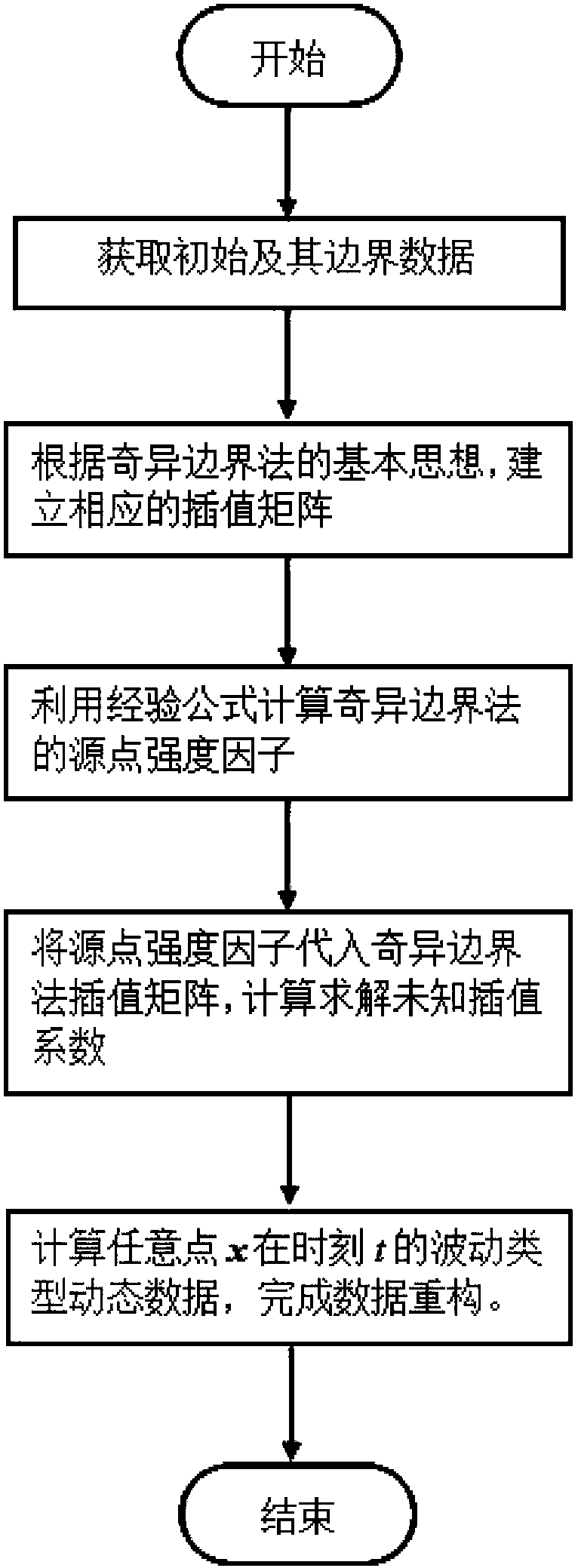
A Dynamic Data Reconstruction Method Based on Singular Boundary Method

A technology of dynamic data and fluctuating data, applied in complex mathematical operations and other directions, it can solve problems such as long calculation time, large calculation load, and complexity, and achieve the effect of fast calculation, high precision and simple mathematics

Inactive Publication Date: 2018-08-21
HOHAI UNIV
View PDF2 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0004] Purpose of the invention: The purpose of the present invention is to provide a simple, efficient, integral-free and grid-free method based on the singular boundary method, aiming at the defects of complex process of dynamic data reconstruction technology of fluctuation type and long calculation time in the prior art. The fluctuation type dynamic data reconstruction method, so as to save reconstruction time and improve reconstruction efficiency

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • A Dynamic Data Reconstruction Method Based on Singular Boundary Method
  • A Dynamic Data Reconstruction Method Based on Singular Boundary Method
  • A Dynamic Data Reconstruction Method Based on Singular Boundary Method

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

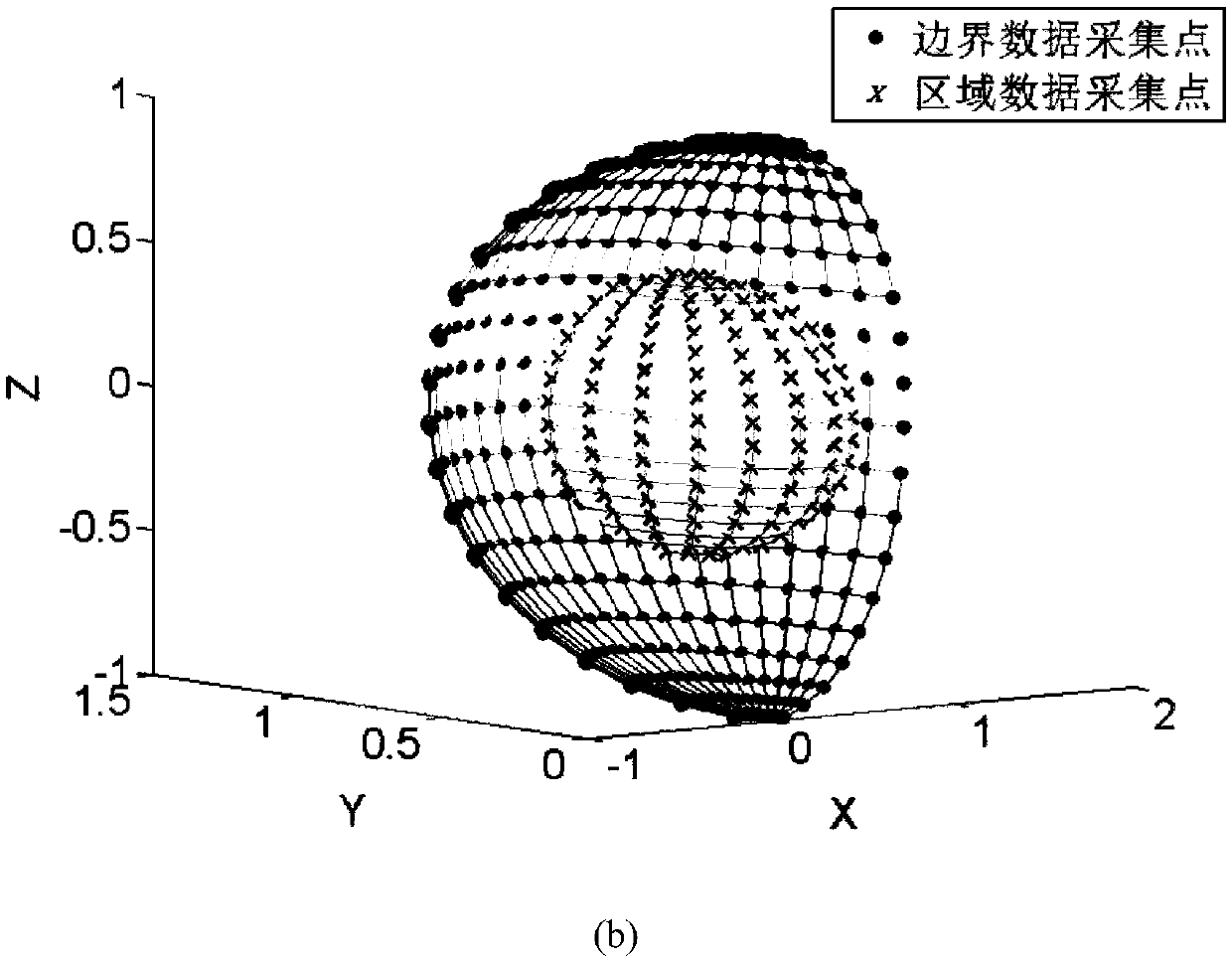
[0074] Example 1: Consider as Figure 4 The tire-shaped regional fluctuation data reconstruction shown, the regional equation is

[0075]

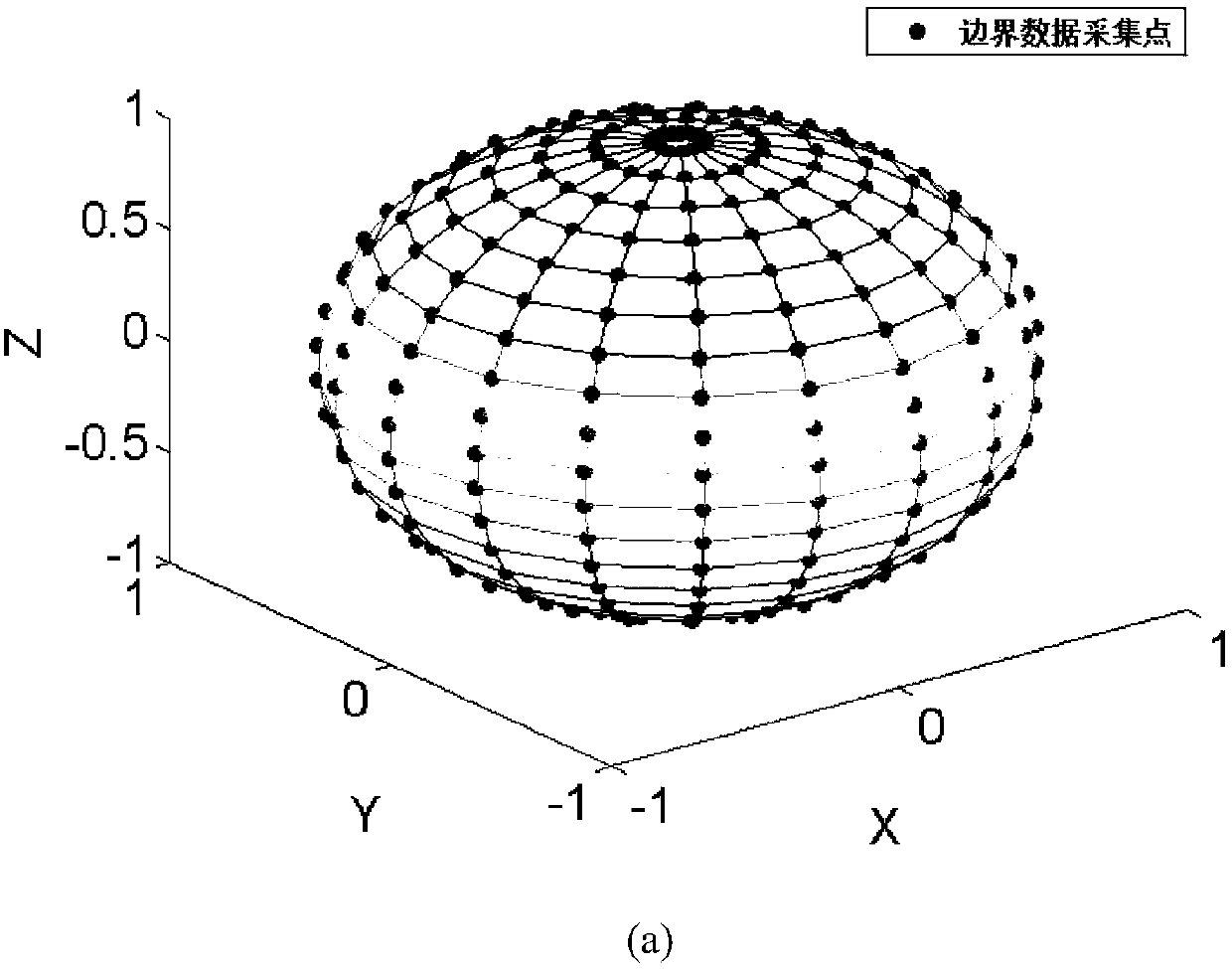
[0076] Where R=0.8, r=0.2, boundary data collection points such as image 3 shown.

[0077] The governing equation and exact solution of the fluctuation data are:

[0078]

[0079] In this example, we apply a time step of Δt = 2×10 -1 , wave velocity c=10, arrange regional data collection points N for each test point f =1255, the test points are arranged on the time layer with a radius of 0.8 and t=1s, Figure 5 The numerical accuracy convergence diagram of the singular boundary method with the increase of the number of boundary acquisition data points is given, where the wave numbers are (k 1 =0.5,k 2 =5,k 3 =10). It can be found that the singular boundary method converges rapidly at the second-order convergence rate, and when k=5, the convergence rate even reaches C=5.0.

[0080] Thereafter, apply a time step of Δt = 2×10 ...

Embodiment 2

[0081] Example 2: Consider the reconstruction of sound pressure radiation data in a unit sphere, where radius a=1, wave velocity c=v 0 , the exact solution can be expressed as:

[0082]

[0083] where z 0 = ρ 0 c, represents dielectric impedance, ρ 0 is the medium density, c is the wave velocity, and ω=kc is the wave frequency. Figure 7 and Figure 8 Partial solution of dimensionless acoustic compaction is given and the imaginary part solution At the boundary data collection point N s =400, the numerical knot reconstruction results under the condition of time step Δt=0.5s. It can be found that the reconstruction results of the singular boundary method and the exact solution fit accurately. In contrast, when the virtual boundary d=0.5, the reconstruction results of the basic solution method and the exact solution fit well, but when the virtual boundary d=0.9 , the data reconstruction results are obviously discrete. At the same time, it should be pointed out that ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a wave type dynamic data reconstruction method based on a singular boundary method. A wave equation time elementary solution is directly used as a kernel function, a source point intensity factor is used for replacing a source point singular item, no numerical value integrals, no singular integrals and no mesh generation are related, the wave equation time elementary solution is directly used as the kernel function, no complex mathematical manipulation exists, and the simple and efficient characters meet the feature requirements of wave type dynamic data reconstruction for quickness, stability and precision of the data reconstruction technology. Experiment comparisons show that when the technology is used for processing the wave type dynamic data reconstruction problem, under the condition of obtaining the similar precision, generally, only about 10% of time taken by a traditional linear boundary element algorithm is consumed, and the method has the advantages that the precision is high, calculation is quick, mathematics is simple, and the procedure is simple and convenient to conduct and can be applied to the engineering technical fields such as sound wave noise reduction, sea wave energy dissipation, earthquake prediction and other wave type data reconstruction and image processing.

Description

technical field [0001] The invention relates to a method for reconstructing dynamic data of a fluctuation type, in particular to a method for reconstructing dynamic data of a fluctuation type based on a singular boundary method. Background technique [0002] As one of the most common natural phenomena in nature, fluctuation phenomenon has a wide range of applications and influences in engineering applications. For example, scientific research and engineering fields such as acoustic noise reduction, ocean wave energy dissipation, and seismic wave prediction need to process a large amount of dynamic data of fluctuation types. Due to the uniqueness of the basic governing equations of wave phenomena, such as the hysteresis of wave propagation, three-dimensional no aftereffect, etc., the basic solutions of the wave equation have obviously different forms from the basic solutions of the diffusion equation and the Laplace equation, which makes the wave Refactoring of type dynamic d...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Patents(China)
IPC IPC(8): G06F17/15
CPCG06F17/15
Inventor 陈文李珺璞
Owner HOHAI UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com