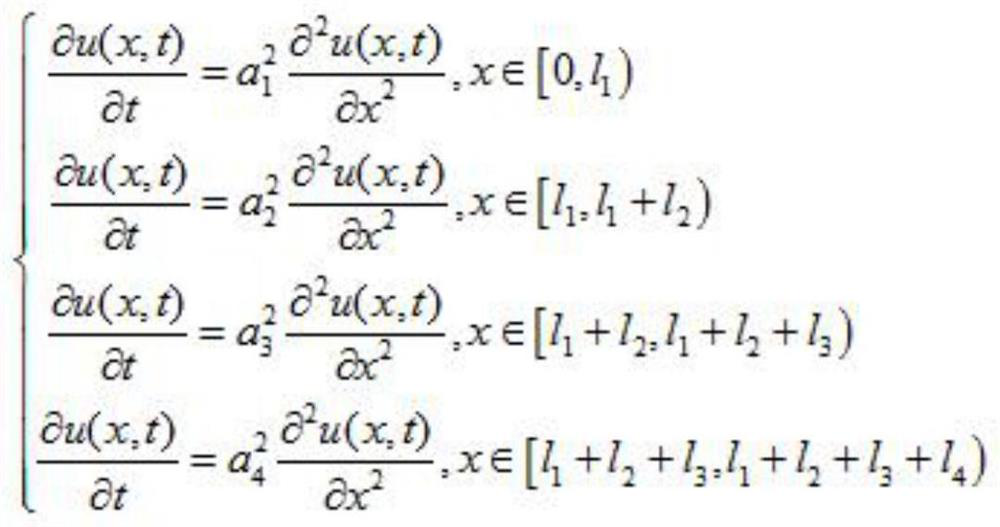Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Method for optimizing thickness of multi-layer heat insulation medium

A technology of medium thickness and optimization method, applied in the field of thermal insulation materials, can solve the problems of inability to optimize the thickness of the medium layer, poor method adaptability, optimization of coating thickness, etc., and achieve the effect of shortening the research and development cycle and reducing the research and development cost.

Pending Publication Date: 2020-10-16
永基众合(山东)智能科技有限公司
View PDF1 Cites 1 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0004] To sum up, the existing methods can only optimize the thickness of the coating, but cannot optimize the thickness of any medium layer, and the method has poor adaptability, and it is impossible to set dynamic constraints according to actual needs, such as the temperature on one side of the medium at t The time does not exceed m minutes

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Method for optimizing thickness of multi-layer heat insulation medium
  • Method for optimizing thickness of multi-layer heat insulation medium
  • Method for optimizing thickness of multi-layer heat insulation medium

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

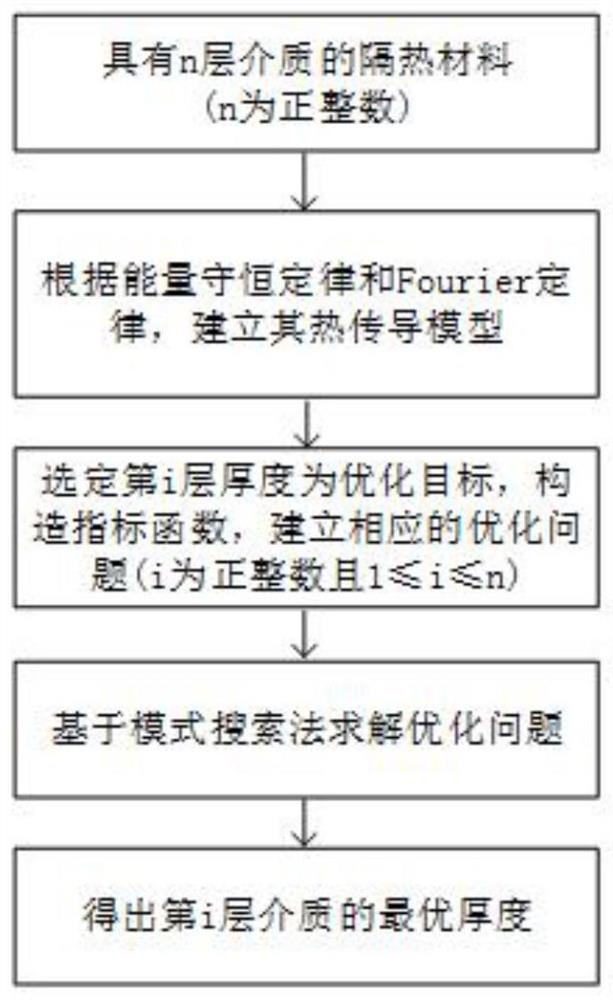
[0028] see figure 1 , a method for optimizing the thickness of a multilayer heat insulating medium, a method for optimizing the thickness of a multilayer heat insulating medium, comprising the following steps:

[0029] Step 1: For a given thermal insulation material with n layers of media, where n is a positive integer, according to the law of energy conservation and Fourier's law, establish its heat conduction model;

[0030] The second step: select the i-th layer thickness as the optimization target, construct the index function, and establish the corresponding optimization problem, i is a positive integer and 1≤i≤n;

[0031] Step 3: Numerically solve the optimization model based on the pattern search method Finally, the optimal thickness of the i-th layer of media can be obtained.

[0032] The existing method can only optimize the thickness of the coating, but cannot optimize the thickness of any medium layer and the method has poor adaptability, and it is impossible to se...

Embodiment 2

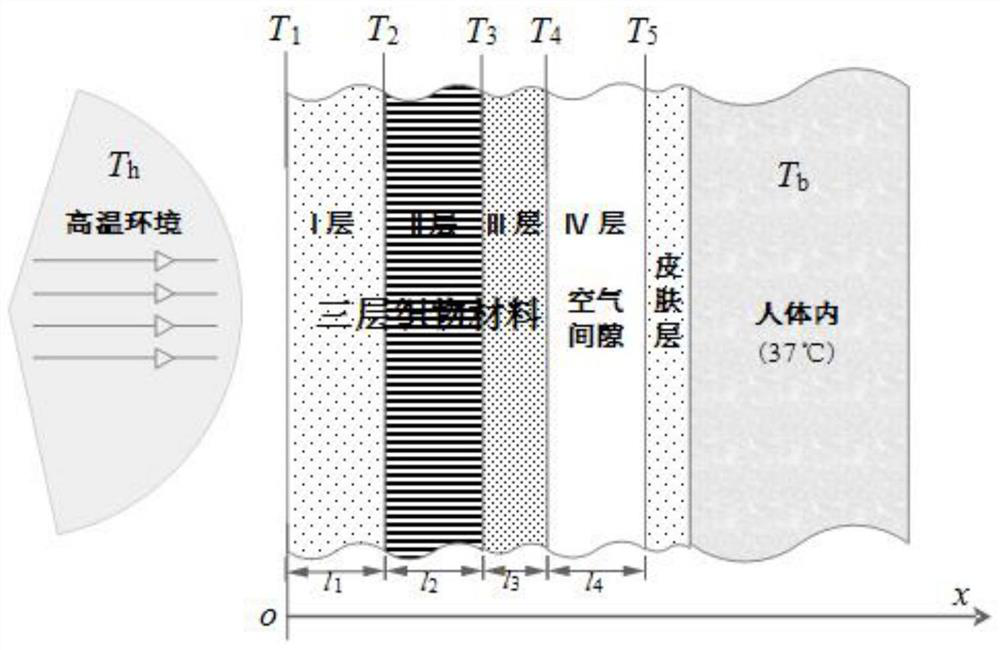
[0036] see figure 2 , image 3 and Figure 4 , when the one-dimensional heat conduction model of multi-layer media is established, at time t, the temperature at any point x in layer I-IV layer media is recorded as u(x,t), where x is the distance from the origin O, according to the uniform The one-dimensional heat conduction equation of the medium can obtain the one-dimensional heat conduction model of the multi-layer medium, such as image 3 As shown, among them, image 3 The initial value and boundary conditions of Equation 1 shown in Figure 4 As shown, among them, k 0 is the convective heat transfer coefficient between the external environment and the surface of layer I, k 5 is the convective heat transfer coefficient between layer IV and the skin surface, is the temperature distribution function at the initial moment.

Embodiment 3

[0038] When constructing an index function and establishing an optimization problem, in order to optimize the thickness of the second layer, customize the index function,

[0039]

[0040] s.t.0.62 <25,(4),(5)

[0041] by t w Indicates the time when the temperature is greater than 44°C, T 5is the skin surface temperature after the experiment. The last two terms are added in because t w and T 5 Neither is an optimization variable. Since it is difficult to constrain it, we adopt the method of penalty function, incorporate it into the indicator term, and convert it into a nonlinear optimization problem with only solution domain constraints. (t w >300) is a judgment statement whose value is:

[0042]

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a method for optimizing the thickness of a multi-layer heat insulation medium. The method comprises the following steps: 1, for a given thermal insulation material with n layers of media, wherein n is a positive integer, establishing a heat conduction model based on the law of conservation of energy and the Fourier law; 2, selecting the thickness of the ith layer as an optimization target, constructing an index function, and establishing a corresponding optimization problem, wherein i is positive integer and is greater than or equal to 1 and less than or equal to n; and3, carrying out numerical solution on the optimization model based on a mode search method to obtain the optimal thickness of the ith layer of medium. The optimal medium thickness is determined through a numerical experiment mode, the research and development cost is reduced, and the research and development period is shortened.

Description

technical field [0001] The invention relates to the technical field of heat insulation materials, in particular to a method for optimizing the thickness of a multilayer heat insulation medium. Background technique [0002] Thermal insulation materials are often composed of multiple media, and the thickness of different media has an important impact on the performance and cost of thermal insulation materials. The application of heat insulation materials is very extensive, and heat insulation materials are divided into three categories: porous materials, heat reflective materials and vacuum materials. The former uses the pores contained in the material itself to insulate heat, because the thermal conductivity of air or inert gas in the pores is very low, such as foam materials, fiber materials, etc.; heat reflective materials have a high reflection coefficient and can reflect heat, such as Gold, silver, nickel, aluminum foil or metal-plated polyester, polyimide film, etc. Va...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G06F30/20G16C60/00G06F111/04G06F111/06G06F111/10G06F113/12G06F119/08
CPCG06F30/20G16C60/00G06F2111/04G06F2111/06G06F2111/10G06F2113/12G06F2119/08
Inventor 祝宝龙张然甘有霖程玉印
Owner 永基众合(山东)智能科技有限公司
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com