Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Discrete Fractional Order Sliding Mode Control Method for Contour Tracking of Linear Motor Platform

A technology of fractional-order sliding mode and fractional-order sliding mode surface, which is applied in the general control system, adaptive control, control/regulation system, etc., and can solve the problems of low control precision, unsuitable digital processor, unstable control process, etc. problems, achieve high control accuracy, avoid chattering effects, and achieve good contour tracking control effects

Active Publication Date: 2022-03-08
HARBIN INST OF TECH
View PDF6 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0004] The purpose of the present invention is to solve the problems that the traditional continuous time control method does not match with the digital processor, and the control process is unstable and the control precision is low, and proposes a discrete fractional order sliding mode control for linear motor platform contour tracking method

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Discrete Fractional Order Sliding Mode Control Method for Contour Tracking of Linear Motor Platform
  • Discrete Fractional Order Sliding Mode Control Method for Contour Tracking of Linear Motor Platform
  • Discrete Fractional Order Sliding Mode Control Method for Contour Tracking of Linear Motor Platform

Examples

Experimental program
Comparison scheme
Effect test

specific Embodiment approach 1

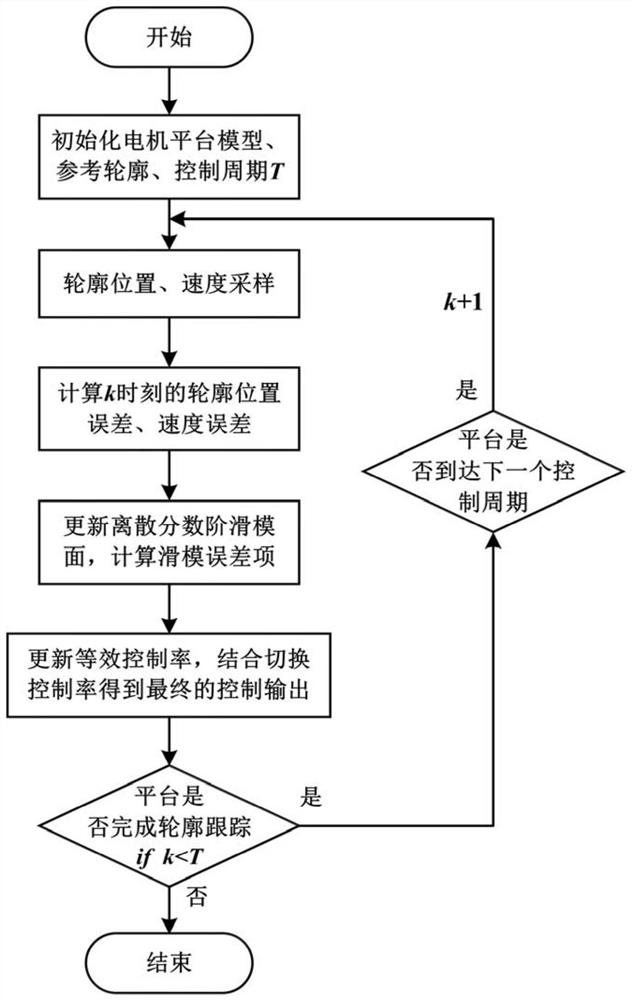
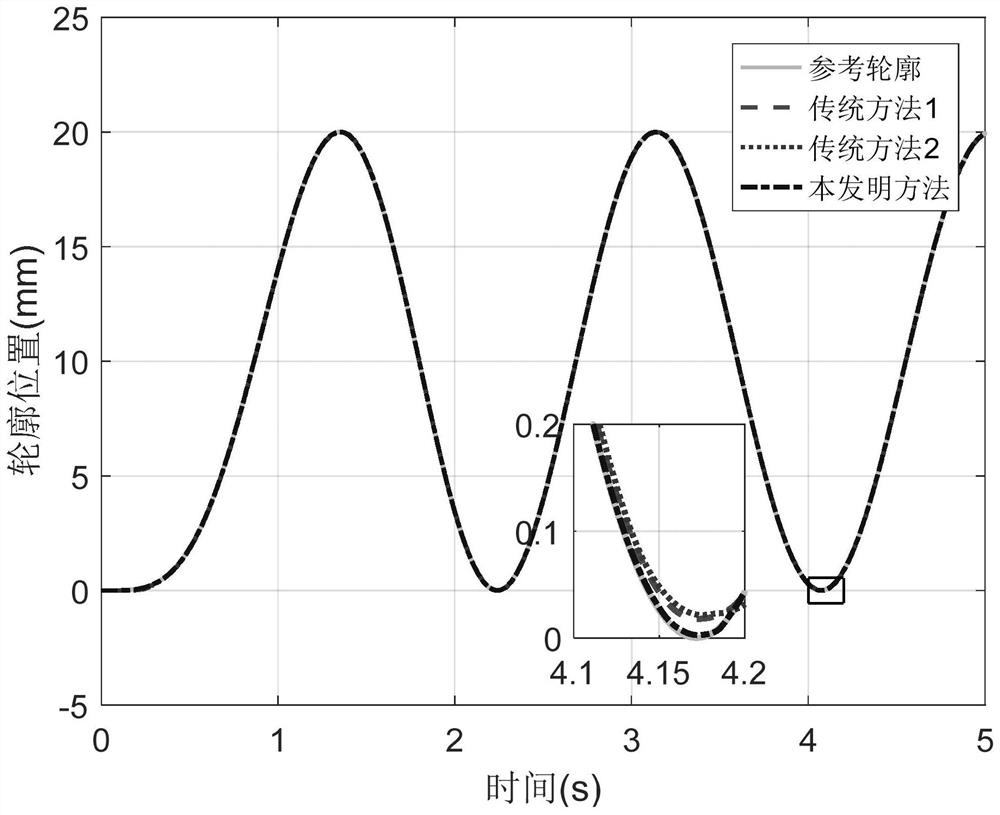
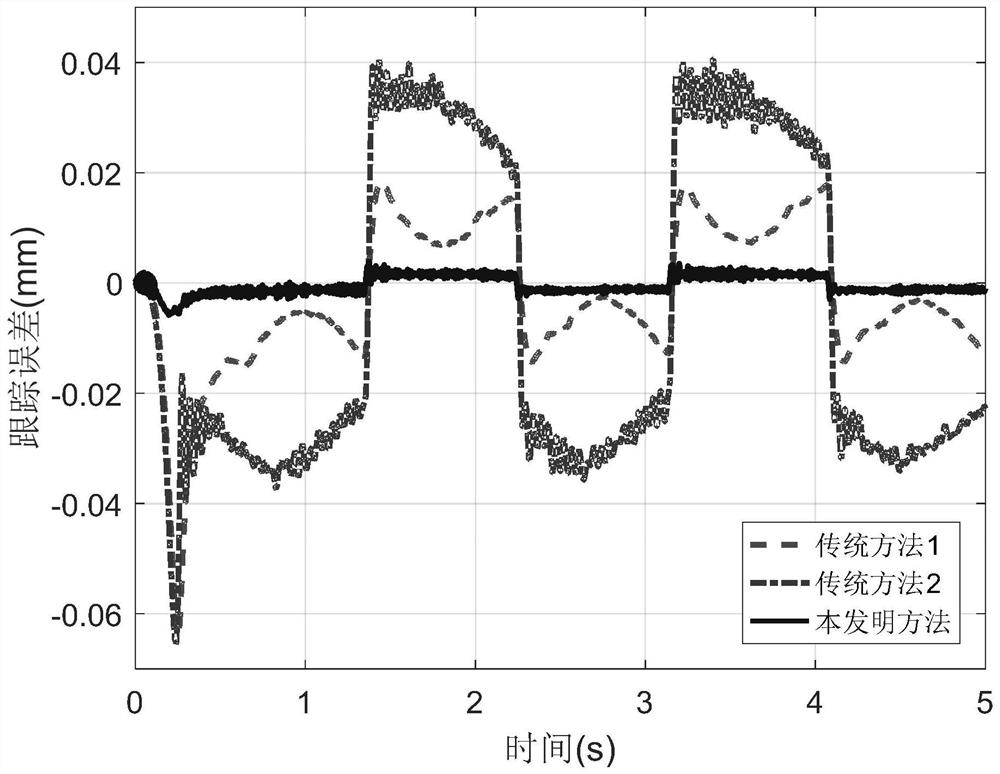
[0053] Specific implementation mode 1. Combination figure 1 This embodiment will be described. A discrete fractional-order sliding mode control method for linear motor platform contour tracking described in this embodiment can effectively solve the problem of incompatibility between traditional continuous time control methods and digital processors, and improve the control accuracy of platform contour tracking. Stabilize the control process and save control costs. In addition, when considering the existence of interference and uncertainty in engineering practice, the method of the present invention can still ensure high-speed, high-precision contour tracking control performance. The inventive method specifically comprises the following steps:

[0054] Step 1: Set the sampling interval h of the control method, obtain the sampling time as t=kh, and k is the discrete system time after sampling. For the linear motor platform system, a discrete linear motor platform system model...

specific Embodiment approach 2

[0078] Specific embodiment 2: This embodiment is a further limitation to specific embodiment 1. The discrete fractional operator is calculated according to the definition of Grünwald-Letnikov fractional order, and its specific expression is as follows:

[0079]

[0080] Among them, λ is any real number, and g(λ, j) is a binomial expression as follows

[0081]

[0082] Other steps and parameters are the same as those in Embodiment 1.

specific Embodiment approach 3

[0083] Specific implementation mode three: this implementation mode is a further limitation to specific implementation mode two. Considering practical engineering applications, a finite step size L is used to calculate the discrete fractional operator as follows, and L is determined according to the digital processor memory and calculation efficiency The positive integer of , thus the discrete fractional order sliding mode method can reduce the cost of control calculation based on the concept of digital control.

[0084]

[0085] where the sum of the coefficients can be expressed as

[0086] Other steps and parameters are the same as in the second embodiment.

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a discrete fractional order sliding mode control method for contour tracking of a linear motor platform, which belongs to the technical field of contour tracking control of a linear motor platform. The invention solves the problems that the traditional continuous time control method is not compatible with the digital processor, and the control process is unstable and the control precision is low. The present invention considers the Coulomb friction and viscous friction generated when the linear motor platform moves, and designs a discrete fractional-order sliding mode contour tracking control method. Benefiting from the historical memory effect of fractional-order calculus, the output response of the designed control method Fast and smooth, better contour tracking control effect and higher control precision can be obtained. Moreover, the method of the invention is suitable for being directly applied to a digital processor, can avoid the influence of chattering on contour tracking, and obtain a more stable control effect. The invention can be applied to the contour tracking of the linear motor platform.

Description

technical field [0001] The invention relates to the technical field of linear motor platform contour tracking control for modern industrial control and high-precision machining, in particular to a discrete fractional order sliding mode control method for linear motor platform contour tracking. Background technique [0002] In the field of modern industrial control and high-precision machining, linear motors have gained widespread attention in recent years due to their advantages of high speed, high precision and large thrust. Linear motors do not have internal magnetic field gaps like permanent magnet servo motors, and do not rely on mechanical transmission mechanisms such as belts, lead screws, and drive shaft gears, so their operation is more stable, with high reliability and long life. The linear motor adopts a direct drive method, which significantly reduces the influence of non-linear factors such as dead zone and friction. Therefore, the linear motor platform system h...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Patents(China)
IPC IPC(8): G05B13/04
CPCG05B13/042
Inventor 孙光辉李晓磊匡治安姚蔚然刘健行
Owner HARBIN INST OF TECH
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com