Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

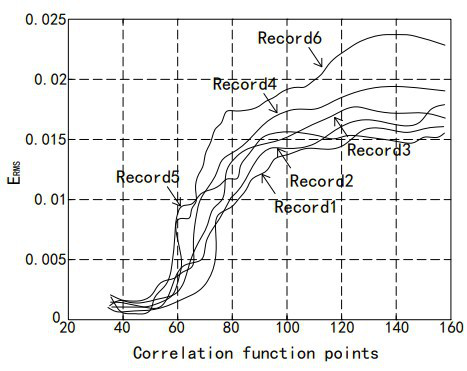
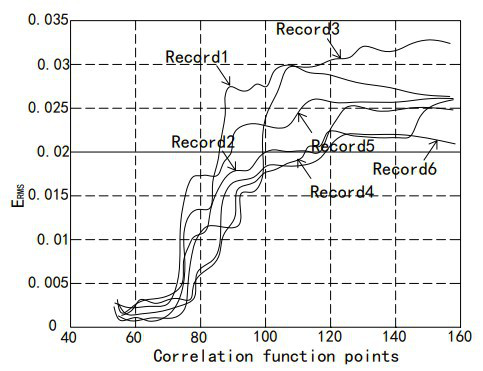
Method for determining root-mean-square error threshold value of optimal truncation position of autocorrelation function

A technology of autocorrelation function and root mean square error, which is applied in the direction of measuring devices, instruments, particle and sedimentation analysis, etc., can solve the problems such as inability to select correlation function points, lack of self-adaptive ability, and inability to select correlation function points, etc., to achieve Effects that improve accuracy and repeatability

Active Publication Date: 2021-10-01
澳谱特科技(上海)有限公司
View PDF4 Cites 0 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

However, under different noise levels, the number of correlation function points selected by this method is basically the same, so this method does not have adaptive ability, and the number of correlation function points cannot be selected according to the noise level of the correlation function
[0007] It can be seen that the two truncation methods of fixed correlation function points and fixed correlation function threshold cannot select the number of correlation function points according to the measured particle size and the noise level of the correlation function.

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Method for determining root-mean-square error threshold value of optimal truncation position of autocorrelation function
  • Method for determining root-mean-square error threshold value of optimal truncation position of autocorrelation function
  • Method for determining root-mean-square error threshold value of optimal truncation position of autocorrelation function

Examples

Experimental program
Comparison scheme
Effect test

Embodiment Construction

[0034] Figure 1-8 It is the best embodiment of the present invention, below in conjunction with attached Figure 1-8 The present invention will be further described.

[0035] Such as figure 1 As shown, a root mean square error threshold method (RMSET) to determine the best truncation position of the autocorrelation function includes the following steps:

[0036] Step 1001, collect the scattered light intensity, and calculate the autocorrelation function;

[0037] Collect the scattered light intensity of nanoparticles through the experimental equipment, and calculate the light intensity autocorrelation function g (2) (τ), when the scattered light field has Gaussian statistical properties, the light intensity autocorrelation function g (2) (τ) and electric field autocorrelation function g (1) The measured value of (τ) satisfies the following relationship:

[0038] g (2) (τ)=1+β|g (1) (τ)| 2

[0039] Among them, τ represents the delay time, β represents the intercept o...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a method for determining the root-mean-square error threshold value of the optimal truncation position of autocorrelation function, and belongs to the technical field of photon correlation spectra. The method is characterized by comprising the following steps: step 1001, collecting scattered light intensity, and calculating an autocorrelation function; step 1002, cutting off an electric field autocorrelation function at a 0.1 position; step 1003, inverting particle size distribution; step 1004, calculating a root-mean-square error of an electric field autocorrelation function; step 1005, judging whether the root mean square error ERMS of the electric field autocorrelation function is greater than 0.0035 or not; step 1006, reducing the number of electric field autocorrelation function points; and step 1007, outputting a final result. According to the method for determining the root-mean-square error threshold value of the optimal truncation position of the autocorrelation function, the optimal truncation position of the correlation function is selected through the correlation function root-mean-square error threshold value method, the method can adapt to measurement of particles of different sizes and has certain adaptive capacity to the noise level of the correlation function, and the accuracy and repeatability of an inversion result are improved.

Description

technical field [0001] The invention relates to a root mean square error threshold value method for determining the best truncation position of an autocorrelation function, which belongs to the technical field of photon correlation spectroscopy. Background technique [0002] Photon correlation spectroscopy technology calculates the autocorrelation function by collecting the scattered light intensity of nanoparticles, and then inverts to obtain the particle size distribution. Although many algorithms have been proposed, none of them can solve the inversion problem well. [0003] During the inversion process, the correlation function data with different points will affect the inversion results. The experimental data show that because the correlation function attenuated to the baseline contains more noise, the inversion process contains more correlation function points, and the accurate particle size distribution results may not be obtained. However, when the number of correl...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G01N15/02
CPCG01N15/0211
Inventor 秦福元秦和义刘伟申晋
Owner 澳谱特科技(上海)有限公司
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com