Normalization possibilistic fuzzy entropy clustering method based on Gaussian kernel hybrid artificial bee colony algorithm
A technology of artificial bee colony algorithm and clustering method, applied in the field of normalized possibility fuzzy entropy clustering, can solve the problem of unstable clustering of separable structure data, and achieve the improvement of global optimization characteristics, good global characteristics, The effect of overall performance improvement
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment
[0076] This embodiment further illustrates the present invention in conjunction with the machine learning standard test set wine data.
[0077] The Wine data is a 13-dimensional dataset with 178 data samples in 3 categories.
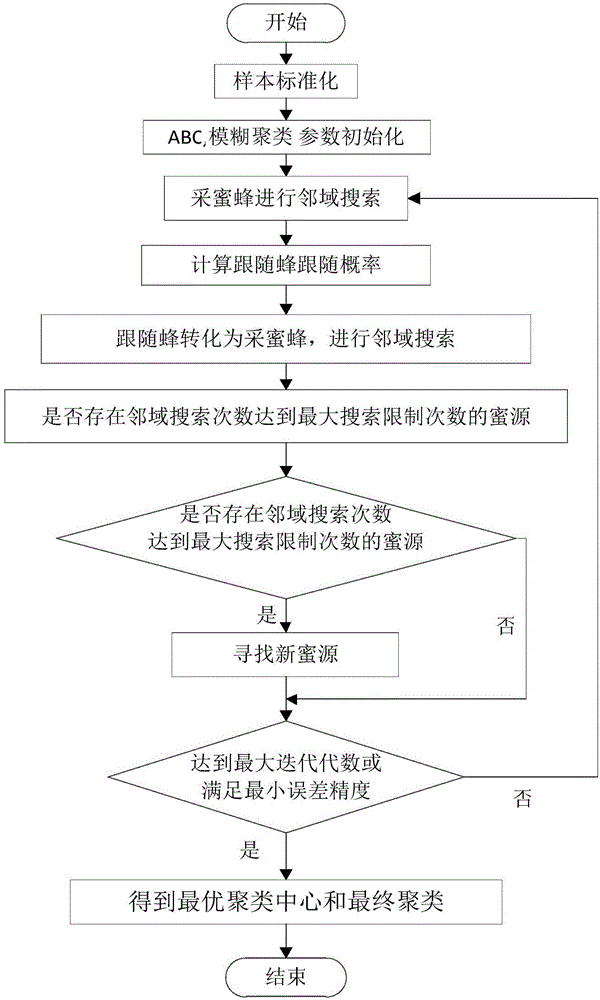
[0078] Such as figure 1 As shown, the normalized likelihood fuzzy entropy clustering method based on Gaussian kernel hybrid artificial bee colony algorithm, the flow chart is as follows figure 1 shown, including the following steps:
[0079] (1) Input the wine data of the sample to be clustered, and perform normalization preprocessing on it to obtain a new sample X_New, so that the new sample X_New falls in the interval [0,1], avoiding the clustering results caused by different dimensions influences.
[0080] (2) Parameter initialization, the population number of the artificial bee colony algorithm NP=50, the number of honey bees SN=25, the local optimal limit number limit=50, the maximum number of iterations maxcycle=500; the number of clusters c=3, ...
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com