Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Signture dynamic discrimination method

A dynamic and trajectory technology, applied in character and pattern recognition, input/output of user/computer interaction, instruments, etc., can solve problems such as difficult linear velocity identification, great difference in stroke strength, and difficulties

Inactive Publication Date: 2008-08-13
UNITED PRECISION MACHINE
View PDF0 Cites 4 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

Due to the consistency of the signatures of people, it is very difficult to identify the authenticity of each person's different signatures and at the same time identify the counterfeit signatures only by plane patterns.
There is also a third dimension that increases the pen-down depth caused by stroke strength on a two-dimensional basis. Image recognition is performed in a three-dimensional space coordinate system, but the stroke strength of people signing on different substrates is very different, and it is necessary to sense the stroke strength The requirements for the pen have become very high; there is also a factor of increasing the speed of the pen on the basis of two dimensions, but it is very difficult to calculate the linear speed including continuous pen, broken pen, jump point and subsequent identification

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Signture dynamic discrimination method
  • Signture dynamic discrimination method
  • Signture dynamic discrimination method

Examples

Experimental program
Comparison scheme
Effect test

specific Embodiment approach 1

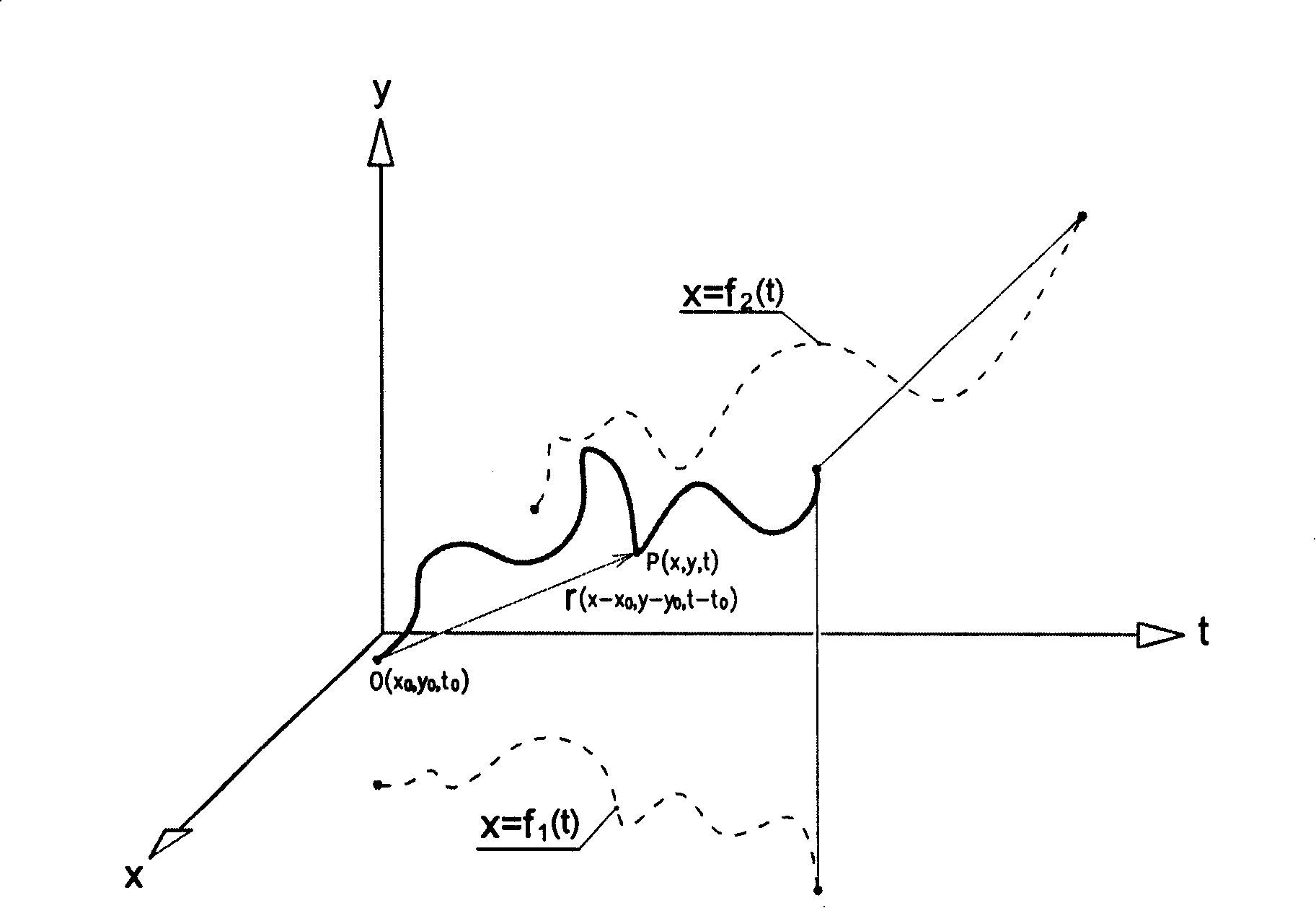
[0032] A scheme for dynamic authentication of signatures, figure 1 Shown is the signature trajectory in the space-time coordinate system x-y-t, the signature trajectory can be expressed as a function of the y-direction position and x-direction position of a pen-down point changing with time t respectively. x=f 1 (t) and y = f 2 (t), and the relationship y=f(x, t) between y and x can be established. The space-time coordinate value of a point P on the signature trajectory is P(x, y, t), and the space-time coordinate value of the initial pen-down point 0 is 0(x 0 ,y 0 , t 0 ). With the initial pen-down point 0 as the starting point and the point P in the signing process as the end point, a set of space-time position vectors r(x-x 0 ,y-y 0 ,t-t 0 ) to describe the spatio-temporal location characteristics of each point on the signature trajectory. The space-time position vector r(x-x 0 ,y-y 0 ,t-t 0 ) group is compared with the space-time position vector group of each co...

specific Embodiment approach 2

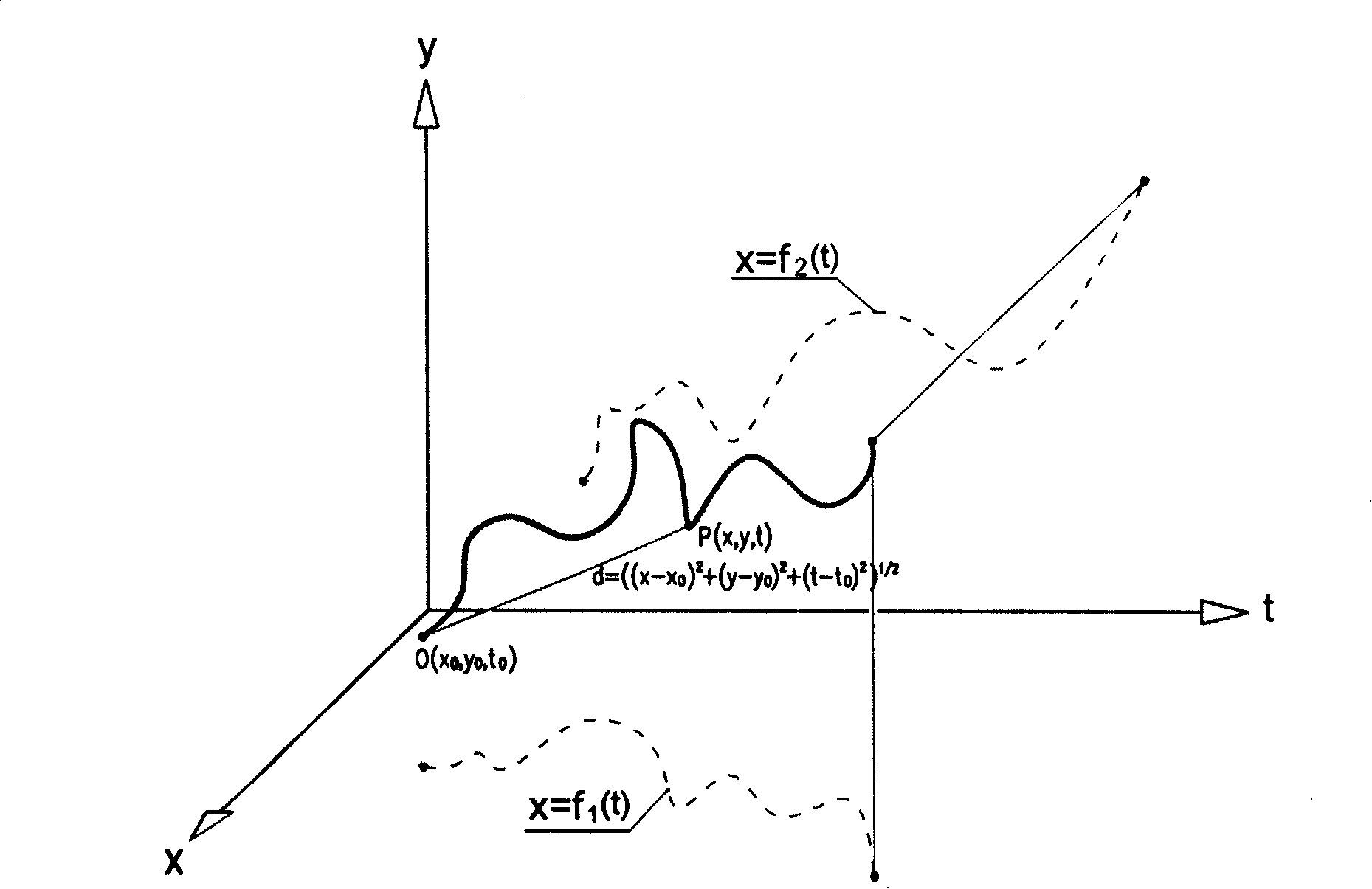
[0034] A scheme for dynamic authentication of signatures, figure 2 Shown is the signature trajectory in the space-time coordinate system x-y-t, the signature trajectory can be expressed as a function of the y-direction position and x-direction position of a pen-down point changing with time t respectively. x=f 1 (t) and y = f 2 (t), and the relationship y=f(x, t) can be established. The space-time coordinate value of a point P on the signature trajectory is P(x, y, t), and the space-time coordinate value of the initial pen-down point 0 is 0(x 0 ,y 0 , t 0 ). Taking the initial pen-down point 0 as one end point and point P in the signing process as the other end point, the space-time distance d=((x-x 0 ) 2 +(y-y 0 ) 2 +(t-t 0 ) 2 ) 1 / 2 , to describe the spatio-temporal location characteristics of each point on the signature trajectory. The space-time distance d=((x-x 0 ) 2 +(y-y 0 ) 2 +(t-t 0 ) 2 ) 1 / 2 The group is compared with the time-space distance group...

specific Embodiment approach 3

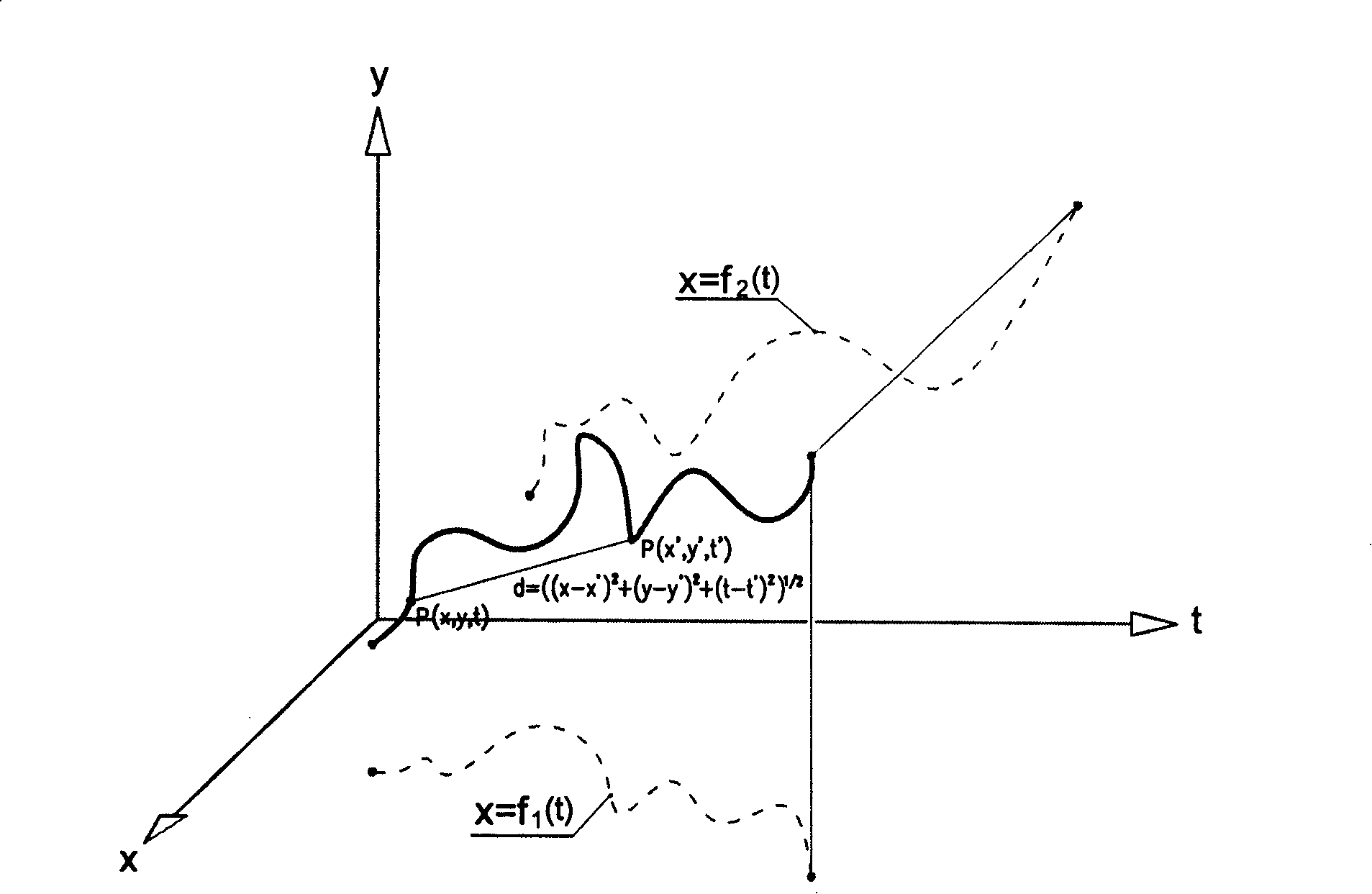
[0036] A scheme for dynamic authentication of signatures, figure 2 Shown is the signature trajectory in the space-time coordinate system x-y-t, the signature trajectory can be expressed as a function of the y-direction position and x-direction position of a pen-down point changing with time t respectively. x=f 1 (t) and y = f 2 (t), and the relation y=f(x, t) can be established. The space-time coordinate value of a point P on the signature trajectory is P(x, y, t), and the space-time coordinate value of the initial pen-down point 0 is 0(x 0 ,y 0 , t 0 ). Taking the initial pen-down point 0 as one end point and point P in the signing process as the other end point, the space-time distance d=((x-x 0 ) 2 +(y-y 0 ) 2 +(t-t 0 ) 2 ) 1 / 2 To describe the spatio-temporal location characteristics of each point on the signature trajectory. In the space-time coordinate system x-y-t, the partial derivative of signature trajectory function y to x ∂ y ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The present invention provides a dynamic authentication method of signature, forming spatio-temporal position of signature track by utilizing time dimension characteristic of being abstrusely imitated of signature, adopting comparing signature track dynamic spatio-temporal position to identify authenticity. The invention records signature track spatio-temporal position and records signature track time position at the same time based on the space coordinate adding time coordinate, making signature more effect by changing signature to the time dimension.

Description

technical field [0001] The invention relates to a signature authentication method, in particular to a signature dynamic authentication method. Background technique [0002] Although the size of the word, the angle and the relative length of each stroke are different when the signer signs each time. It is a mature technology to record the spatial trajectory of written characters, perform mathematical processing and recognition on the trajectory, and convert written characters into printed characters. But it is still difficult to identify the authenticity of the signature. [0003] The signature authentication involved in the present invention is not for converting the signature into standard printed characters, but for authenticating the signature. The so-called signature, what is signed is not necessarily a word, but should be regarded as a mark in a broad sense. [0004] The current signature identification is a two-dimensional pattern identification, which is to record ...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06K9/00G06K9/80G06F3/03
Inventor 陈其良
Owner UNITED PRECISION MACHINE
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com